
01 Indefinite Integrals And The Net Change Theorem Mam1020f Studocu 01 indefinite integrals and the net change theorem. lecture notes on interation. course. mathematics for engineers 1a (mam1020f) 01 indefinite integrals and the net change theorem. course: mathematics for engineers 1a (mam1020f) studocu is not affiliated to or endorsed by any school, college or university. Studying mam1020f mathematics for engineers 1a at university of cape town? on studocu you will find 63 tutorial work, 47 lecture notes, 41 practice materials and.
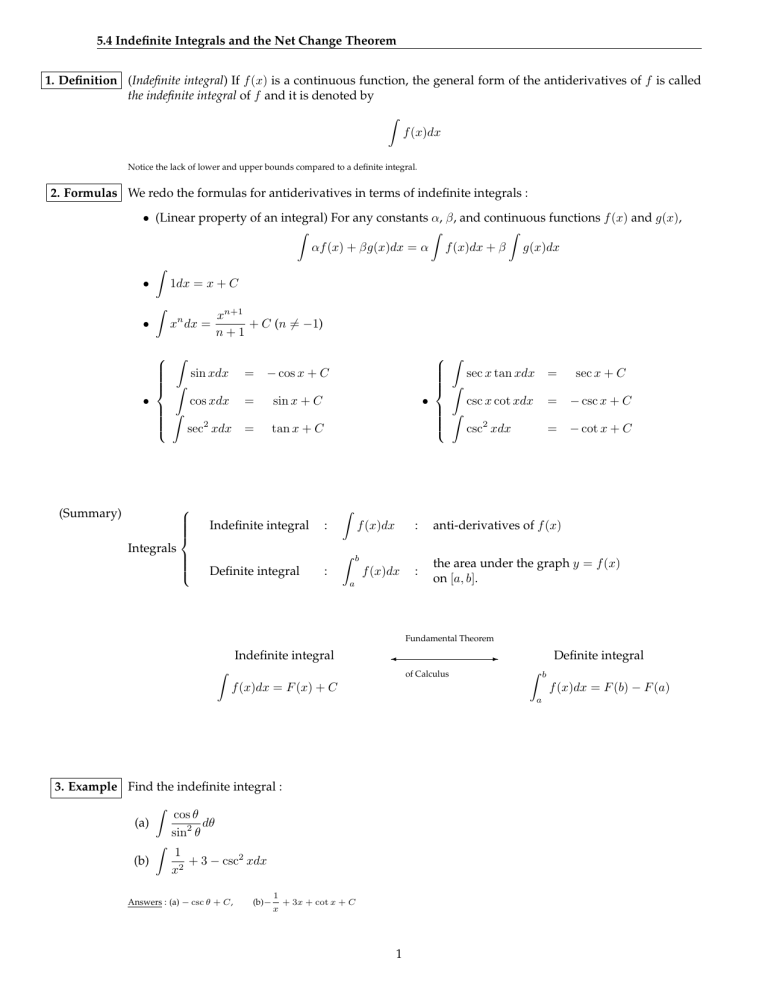
5 4 Indefinite Integrals And The Net Change Theorem 1 Definition An indefinite integral is f ( ) x dx function. basically, use the derivative chart backwards. remember to have a " c "at the end of all indefinite integrals! recall: the most general antiderivative on an interval is defined only when the function was. continuous on the interval. f ( ) x f x dx ( ) 0 constant. n ax. 1. 1. a n x c n. The net change theorem considers the integral of a rate of change. it says that when a quantity changes, the new value equals the initial value plus the integral of the rate of change of that quantity. Result 3.1. if f′(x) is the rate of change of f(x), then the definite integral of f′(x) from a to b is equal to the net change of f(x) over [a,b]. more precisely, the integral of a rate of change is the net change i.e. z b a f′(x)dx = f(b)− f(a). this can be interpreted in many different ways depending upon what f(x) is measuring. It defines indefinite integrals as representing an entire family of antiderivatives rather than a single function. the net change theorem states that the integral of a rate of change between two values is equal to the net change in the related function over that interval.
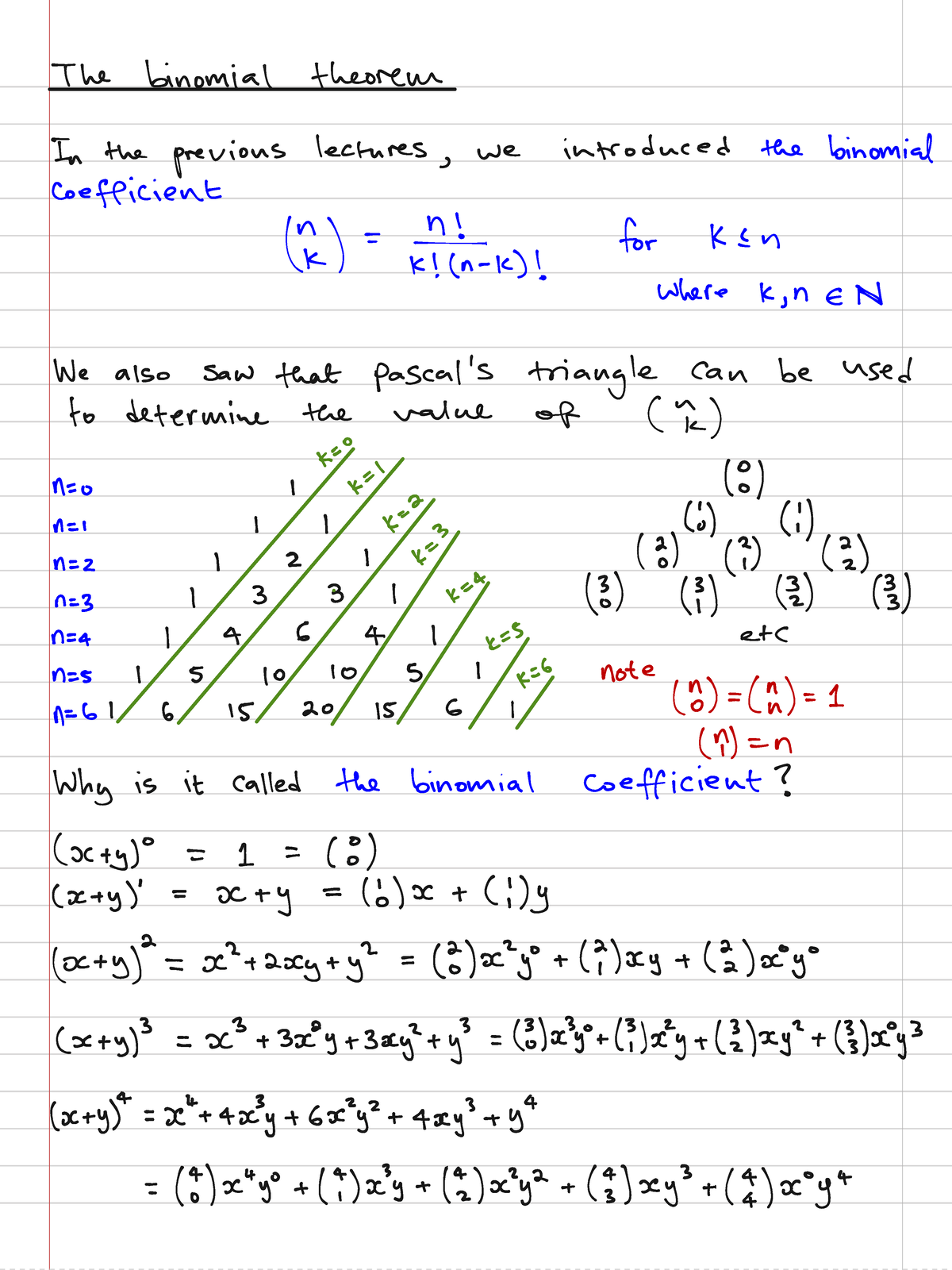
22 The Binomial Theorem Mam1020f Studocu Result 3.1. if f′(x) is the rate of change of f(x), then the definite integral of f′(x) from a to b is equal to the net change of f(x) over [a,b]. more precisely, the integral of a rate of change is the net change i.e. z b a f′(x)dx = f(b)− f(a). this can be interpreted in many different ways depending upon what f(x) is measuring. It defines indefinite integrals as representing an entire family of antiderivatives rather than a single function. the net change theorem states that the integral of a rate of change between two values is equal to the net change in the related function over that interval. Terms: net clange theorenn, elisplacemct, indefinite integrals sourre: stevart's calculus earl transccrndentals (9th ca.) 5 the net change theorem recall. ftc 2: 1ffis eontimois on a, b}. then sr)dr = f (b) f (a). note. another formulation of the ftc 2: if f (t) is continuous ou [a, b], then f (t) dt = f (b) f (a). Note: indefinite integrals are those integrals without boundary, i.e. ∫f (x)dx. ∫f (x)dx =f(x) c; where . f'(x) = f (x) ex: evaluate the following integrals: a) ∫(5x−1)(x 3)dx. Indefinite integrals and the net change theorem what is an indefinite integral? an indefinite integral of a function f is an anti derivative of f, and is denoted r f(x)dx. that is: z f(x)dx = f(x) means f0(x) = f(x) convention #1: we always write all anti derivatives. e.g. z xdx = 1 2 x2 c convention #2: the formula for an anti derivative. The net change theorem states that when a quantity changes, the final value equals the initial value plus the integral of the rate of change. net change can be a positive number, a negative number, or zero.

Applications Of Integrals 1 Moments And Net Vs Chegg Terms: net clange theorenn, elisplacemct, indefinite integrals sourre: stevart's calculus earl transccrndentals (9th ca.) 5 the net change theorem recall. ftc 2: 1ffis eontimois on a, b}. then sr)dr = f (b) f (a). note. another formulation of the ftc 2: if f (t) is continuous ou [a, b], then f (t) dt = f (b) f (a). Note: indefinite integrals are those integrals without boundary, i.e. ∫f (x)dx. ∫f (x)dx =f(x) c; where . f'(x) = f (x) ex: evaluate the following integrals: a) ∫(5x−1)(x 3)dx. Indefinite integrals and the net change theorem what is an indefinite integral? an indefinite integral of a function f is an anti derivative of f, and is denoted r f(x)dx. that is: z f(x)dx = f(x) means f0(x) = f(x) convention #1: we always write all anti derivatives. e.g. z xdx = 1 2 x2 c convention #2: the formula for an anti derivative. The net change theorem states that when a quantity changes, the final value equals the initial value plus the integral of the rate of change. net change can be a positive number, a negative number, or zero.
