
Readings On Vectors From Essential Physics Chapter 1 Adding and subtracting vectors. working with vectors in 1d, 2d & 3d. calculating magnitude of vectors. calculating a unit vector parallel to a vector. We can add vectors in any order we want: a b = b a. we say that vector addition is “commutative”. we express vectors in component form using the unit vectors i, j and k, which each have magnitude 1 and point along the x, y and z axes of the coordinate system, respectively.

Physics 20 Lesson 1 3 Unit Vectors And Product Of Vectors Part 2 There are a variety of methods for determining the magnitude and direction of the result of adding two or more vectors. the two methods that will be discussed in this lesson and used throughout the entire unit are: the pythagorean theorem and trigonometric methods; the head to tail method using a scaled vector diagram. Vector addition. there are 2 graphical methods to perform vector addition. let us assume we have vectors such that \(\vec{a} \vec{b} =\vec{c}\). 1) the parallelogram method: place vectors \(\vec{a}\) and \(\vec{b}\) together tail to tail and then construct a parallelogram. To add several vectors, use the head to tail method. the vectors can be added in any order. figure 1.14 shows how to subtract vectors. product ca has magnitude |c|a. figure 1.15 illustrates multiplication of a vector by a positive scalar and a negative scalar. first add the vectors graphically. The classic way to add vectors is to represent them with arrows, draw them to scale, and then measure out things with a ruler and protractor to see what the answer would be.
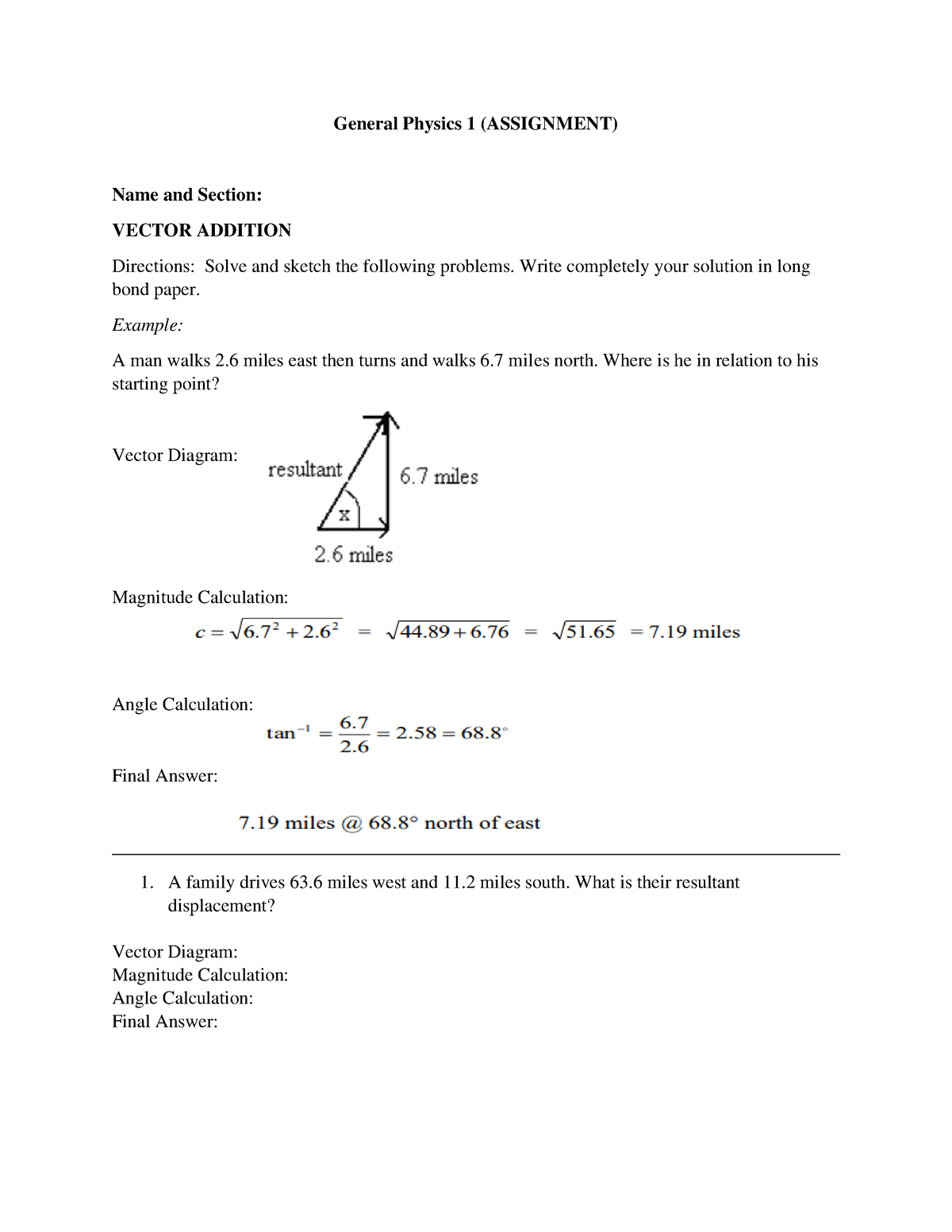
Adding Vectors Assignment General Physics 1 Assignment Name And To add several vectors, use the head to tail method. the vectors can be added in any order. figure 1.14 shows how to subtract vectors. product ca has magnitude |c|a. figure 1.15 illustrates multiplication of a vector by a positive scalar and a negative scalar. first add the vectors graphically. The classic way to add vectors is to represent them with arrows, draw them to scale, and then measure out things with a ruler and protractor to see what the answer would be. •identify the magnitude and direction of a vector. •combine vectors graphically and calculate the magnitude and direction. success criteria: . • magnitude is calculated correctly (pythagorean theorem). • direction is correct: angle (using trigonometry) or direction (e.g.,“south”, “to the right”, “in the negative direction”, etc.) . Exercise 1.31 a disoriented physics professor drives 3.25 km north, then 2.20 km west, and then 1.50 km south. find the magnitude and direction of the resultant displacement, using the method of components. in a vector addition diagram (roughly to scale), show that the resultant displacement found from your diagram is. To make vectors more useful, and to add or subtract them, we need to know how to find the components of a vector. we can do that by using the trig functions. we can also use those to find the magnitude and angle of a vector when starting with the components. Q: check if the magnitude of ෝ𝒂is indeed 1. • vectors can be added, subtracted, and multiplied by an scalar. • vector multiplication is not uniquely defined, but a number of different types of products, such as scalar product and cross product can be defined for a pair of vectors. exercise: 1. find the magnitude and unit vector.

3 2 Unit Vectors Adding Vectors By Components Pdf Euclidean Vector •identify the magnitude and direction of a vector. •combine vectors graphically and calculate the magnitude and direction. success criteria: . • magnitude is calculated correctly (pythagorean theorem). • direction is correct: angle (using trigonometry) or direction (e.g.,“south”, “to the right”, “in the negative direction”, etc.) . Exercise 1.31 a disoriented physics professor drives 3.25 km north, then 2.20 km west, and then 1.50 km south. find the magnitude and direction of the resultant displacement, using the method of components. in a vector addition diagram (roughly to scale), show that the resultant displacement found from your diagram is. To make vectors more useful, and to add or subtract them, we need to know how to find the components of a vector. we can do that by using the trig functions. we can also use those to find the magnitude and angle of a vector when starting with the components. Q: check if the magnitude of ෝ𝒂is indeed 1. • vectors can be added, subtracted, and multiplied by an scalar. • vector multiplication is not uniquely defined, but a number of different types of products, such as scalar product and cross product can be defined for a pair of vectors. exercise: 1. find the magnitude and unit vector.
Three Unit Vectors Are Directed As Shown In The Figure Find The Magnitud To make vectors more useful, and to add or subtract them, we need to know how to find the components of a vector. we can do that by using the trig functions. we can also use those to find the magnitude and angle of a vector when starting with the components. Q: check if the magnitude of ෝ𝒂is indeed 1. • vectors can be added, subtracted, and multiplied by an scalar. • vector multiplication is not uniquely defined, but a number of different types of products, such as scalar product and cross product can be defined for a pair of vectors. exercise: 1. find the magnitude and unit vector.
