
Factorise The Following Using The Identity A 2 2 A B B Factorise the following, using the identity a² 2ab b² = (a b)². (i) x² 6x 9, (ii) x² 12x 36, (iii) x² 14x 49, (iv) x² 2x 1, (v) 4x² 4x 1, (vi) a²x² . solution: (i) x² 6x 9. given, x² 6x 9. using the identity a² 2ab b² = (a b)², the expression can be written as, = (x)² 2 (x) (3) (3)². = (x 3)². Summary: factorising the following, using the identity (a b) 2 = a 2 2ab b 2, (i) x 2 8x 16 , (ii) x 2 10x 25, (iii) y 2 14y 49 , (iv) p 2 2p 1, (v) 4a 2 4ab b 2, (vi) p 2 y 2 2py 1, (vii) a 2 y 2 2aby b 2, (viii) 9x 2 12x 4, (ix) 4y 2 12y 9, (x) x 2 4 2x 4 (xi) a 2 y 3 2aby 2 b 2 y (xii) 9y 2.

1 Factorise The Following Using The Identity A2 2ab B2 A B 2 I X2 Take suitable number of cards given in the adjoining diagram [g(x × x) representing x 2, r(x × 1) representing x and y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles: x 2 4x 4. Factorise the following using the identity a² b² = (a b) (a b). solution: (i) x² 9. given, x² 9 = x² (3)². now, using the identity: a² b² = (a b) (a b), where a = x and b = 3 the expression can be written as, x² 9 = x² (3)² = (x 3)(x 3) (ii) 4x² 25y². given, 4x² 25y² = (2x)² (5y)². Expansion of (a b)2 = a2 2ab b2. is there an error in this question or solution? q 89. (iv) q 89. (iii) q 89. (v) exercise | q 89. (iv) | page 234. Using identities, evaluate 99 2. using a 2 − b 2 = (a b) (a − b), find (1.02) 2 − (0.98) 2. expand `("a" 2 "b" 3)^2` use an expansion formula to find the value. (1005) 2. use the formula to multiply the following. (a 6) (a − 6) expand: (2x 3y) 2. expand: (51) 2. factorise the following, using the identity a 2 2ab b 2 = (a b.
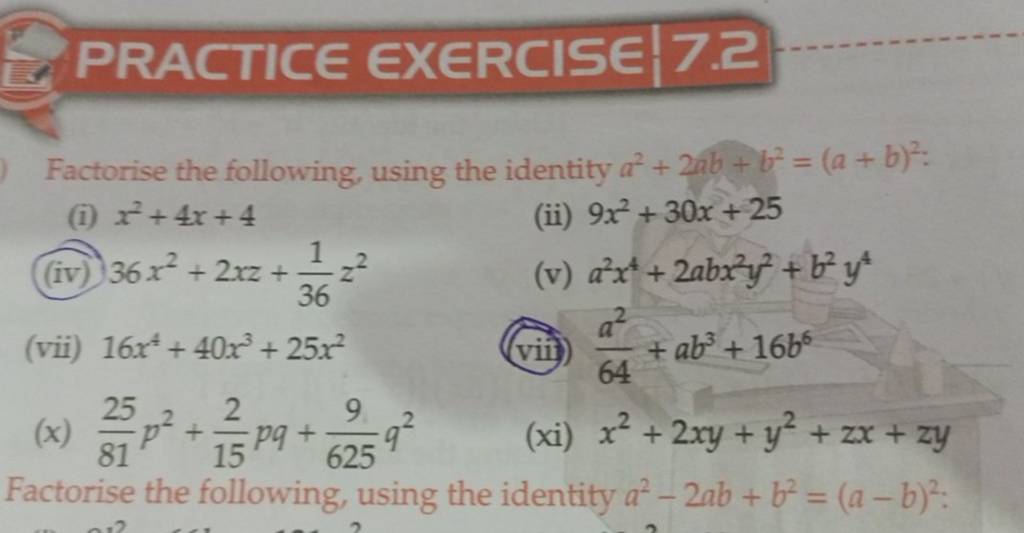
Factorise The Following Using The Identity A2 2ab B2 A B 2 I X2 4x Expansion of (a b)2 = a2 2ab b2. is there an error in this question or solution? q 89. (iv) q 89. (iii) q 89. (v) exercise | q 89. (iv) | page 234. Using identities, evaluate 99 2. using a 2 − b 2 = (a b) (a − b), find (1.02) 2 − (0.98) 2. expand `("a" 2 "b" 3)^2` use an expansion formula to find the value. (1005) 2. use the formula to multiply the following. (a 6) (a − 6) expand: (2x 3y) 2. expand: (51) 2. factorise the following, using the identity a 2 2ab b 2 = (a b. A2 2ab b2 = (a b)2. a2 2ab b2 = (a b)2. a2 b2 = (a b)(a b) x2 (a b)x ab = (x a)(x b) dividing a polynomial by a monomial: when dividing a polynomial by a monomial, you can divide each term of the polynomial by the monomial or use the common factor technique. question:2(iv) factorise the following expressions $(iv. Let’s factorise using these identities x 2 4x 4 x 2 4x 4 = x 2 4 4x = x 2 (2) 2 2 × 2 × x using (a b) 2 = a 2 b 2 2ab where a = x , b = 2 = (x 2) 2 4x 2 12x 9 4x 2 12x 9 = 4x 2 9 12x = (2x) 2 3 2 2 × 2x × 3. (a b)(a b 2c) step by step explanation: the given expression can be factorised as follows: a² b² 2ab 2bc 2ac using the identity (a b)2 = a2 b2 2ab. applying formula,we get ⇒(a b)² 2(bc ca) looking for common sub expressions : (a b) ⇒(a b)² 2c(b a) ⇒(a b) (a b 2c) can't be factorized any more. Learn from their 1 to 1 discussion with filo tutors. was this solution helpful?.

Ex 2 4 3 I Factorise 9x2 6xy Y2 Using Appropriate Identities A2 2ab b2 = (a b)2. a2 2ab b2 = (a b)2. a2 b2 = (a b)(a b) x2 (a b)x ab = (x a)(x b) dividing a polynomial by a monomial: when dividing a polynomial by a monomial, you can divide each term of the polynomial by the monomial or use the common factor technique. question:2(iv) factorise the following expressions $(iv. Let’s factorise using these identities x 2 4x 4 x 2 4x 4 = x 2 4 4x = x 2 (2) 2 2 × 2 × x using (a b) 2 = a 2 b 2 2ab where a = x , b = 2 = (x 2) 2 4x 2 12x 9 4x 2 12x 9 = 4x 2 9 12x = (2x) 2 3 2 2 × 2x × 3. (a b)(a b 2c) step by step explanation: the given expression can be factorised as follows: a² b² 2ab 2bc 2ac using the identity (a b)2 = a2 b2 2ab. applying formula,we get ⇒(a b)² 2(bc ca) looking for common sub expressions : (a b) ⇒(a b)² 2c(b a) ⇒(a b) (a b 2c) can't be factorized any more. Learn from their 1 to 1 discussion with filo tutors. was this solution helpful?.
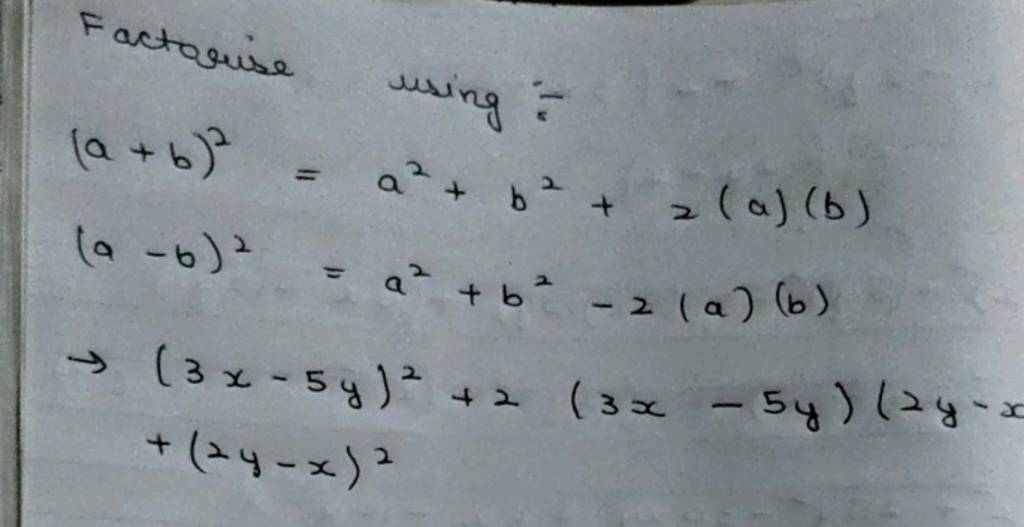
Factorise Using A B 2 A2 B2 2 A B A B 2 A2 B2 2 A B 3x 5y 2 2 3x (a b)(a b 2c) step by step explanation: the given expression can be factorised as follows: a² b² 2ab 2bc 2ac using the identity (a b)2 = a2 b2 2ab. applying formula,we get ⇒(a b)² 2(bc ca) looking for common sub expressions : (a b) ⇒(a b)² 2c(b a) ⇒(a b) (a b 2c) can't be factorized any more. Learn from their 1 to 1 discussion with filo tutors. was this solution helpful?.
