
11th Ans Q 11 If The Roots Of The Quadratic Equation B Cx2 X C A A B 0 If the roots of the equation (b c)x 2 (c a)x (a b) = 0 are equal, then prove that 2b = a c. solution show solution the given quadric equation is(b c)x 2 (c a)x (a b) = 0, and roots are real. A polynomial equation of degree n has n roots; the values of a variable, that satisfy the given equation are called roots of a quadratic equation; the solution to quadratic equations can also be calculated using the factorisation method; if α and β are the roots of a quadratic equation, then the equation is x 2 – (α β) x αβ = 0.
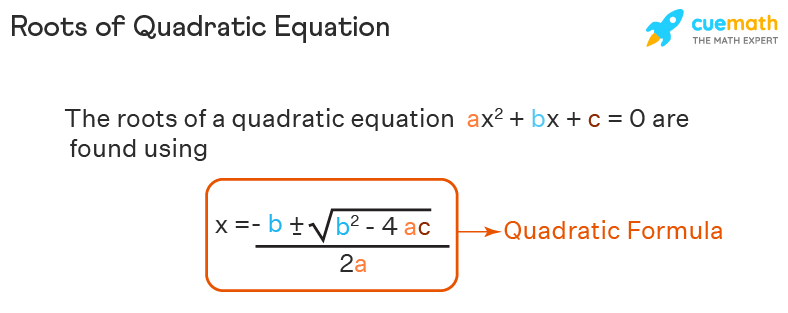
Roots Of Quadratic Equation Formula How To Find Examples 5. if p and q are the roots of the equation x 2 px q =0 then, what are the values of p and q? a) p = 1, q = 2 b) p = 0, q = 1 c) p = 2, q = 0 d) p = 2, q = 1 view answer. If one root of the equation \[x^2 px 12 = 0\] while the equation \[x^2 px q = 0\] has equal roots, the value of q is. For what value of θ, 1 lies between the roots of the quadratic equation 3x 2 – 3sinθ x – 2cos 2 θ = 0? a) 2nπ π 6 < θ < 2nπ 5π 6 b) 2nπ π 3 < θ < 2nπ 5π 3. Quadratic equation: if roots of quadratic equation ax2 bx c = 0 (a ≠ 0) are α and β, then sum of roost = s = α β = b a = – coefficient of x coefficient of x 2 product of roots = p = α.
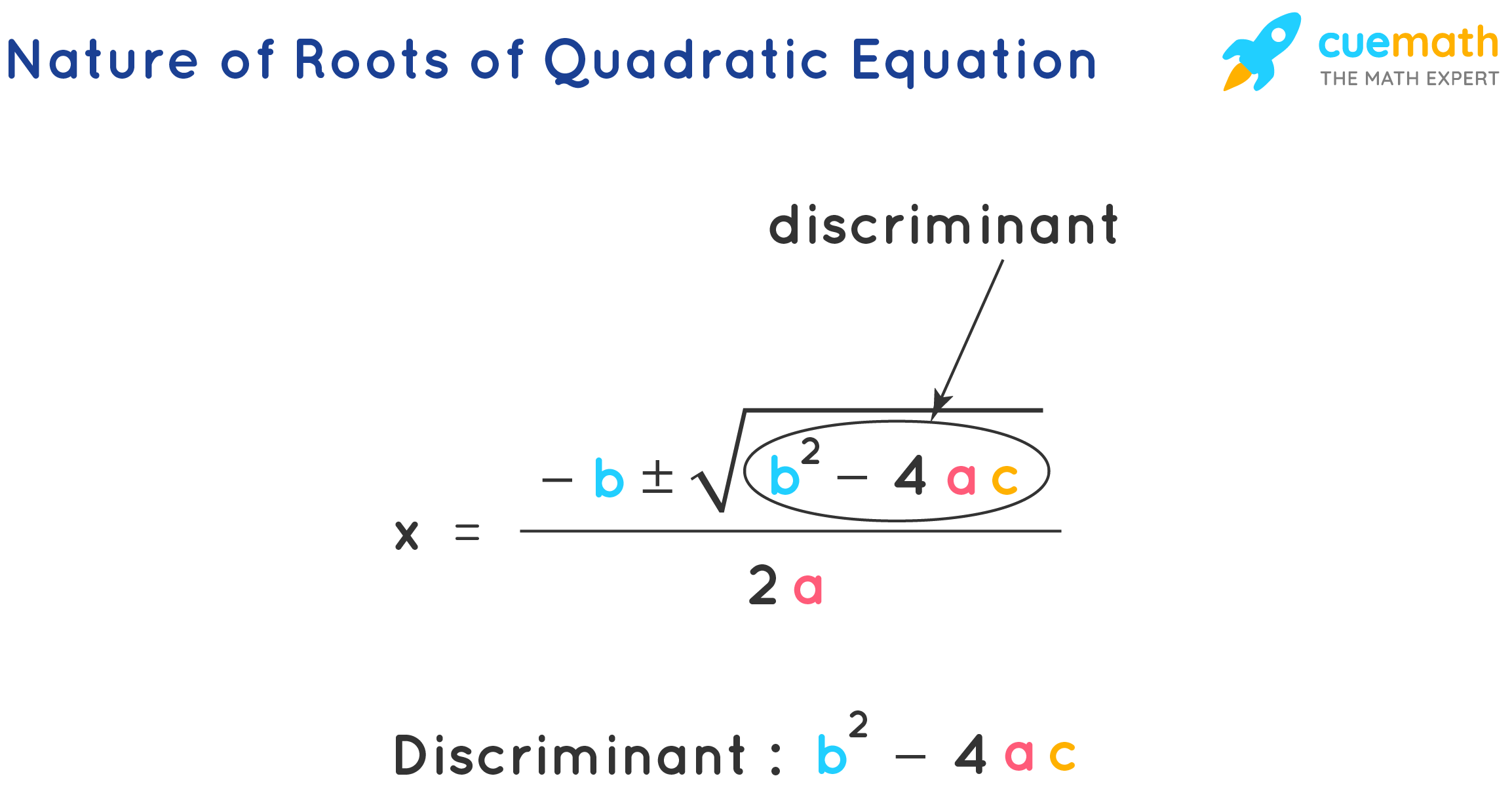
Quadratic Equations Formulas Methods And Examples For what value of θ, 1 lies between the roots of the quadratic equation 3x 2 – 3sinθ x – 2cos 2 θ = 0? a) 2nπ π 6 < θ < 2nπ 5π 6 b) 2nπ π 3 < θ < 2nπ 5π 3. Quadratic equation: if roots of quadratic equation ax2 bx c = 0 (a ≠ 0) are α and β, then sum of roost = s = α β = b a = – coefficient of x coefficient of x 2 product of roots = p = α. 1) construct a quadratic equation with roots 7 and −3. solution. 2) a quadratic polynomial has one of its zeros 1 √5 and it satisfies p(1) = 2. find the quadratic polynomial. solution. 3) if α and β are the roots of the quadratic equation x 2 √2x 3 = 0, form a quadratic polynomial with zeroes 1 α, 1 β . solution. If α & β are the roots of the quadratic equation ax bx c = 0 , then ; (i) αβ = b a. (ii) α β = c a. (iii) α β = d. nature of roots. (1) consider the quadratic equation ax bx c = 0 where then ; (i) d > 0 ⇔ roots are real & distinct (unequal) (ii) d = 0 ⇔ roots are real & coincident (equal) (iii) d < 0 ⇔ roots are imaginary. A quadratic equation can be solved to get two x values or the problem's two roots. the roots of the quadratic equation can be found using one of four ways. the following are the four ways of solving quadratic equations. factoring of quadratic equation. formula method of finding roots. method of completing the square. graphing method to find the. • quadratic formula : if b2 – 4ac ≥ 0, then the real roots of the quadratic equation ax2 bx c = 0 are given by 2 4 22 −− ± b b ac aa. • the expression b2 – 4ac is called the discriminant of the quadratic equation. • existence of roots of a quadratic equation: a quadratic equation ax2 bx c=0 has quadratic equations chapter 4.

3 Ways To Find The Roots Of A Quadratic Equation Wikihow 1) construct a quadratic equation with roots 7 and −3. solution. 2) a quadratic polynomial has one of its zeros 1 √5 and it satisfies p(1) = 2. find the quadratic polynomial. solution. 3) if α and β are the roots of the quadratic equation x 2 √2x 3 = 0, form a quadratic polynomial with zeroes 1 α, 1 β . solution. If α & β are the roots of the quadratic equation ax bx c = 0 , then ; (i) αβ = b a. (ii) α β = c a. (iii) α β = d. nature of roots. (1) consider the quadratic equation ax bx c = 0 where then ; (i) d > 0 ⇔ roots are real & distinct (unequal) (ii) d = 0 ⇔ roots are real & coincident (equal) (iii) d < 0 ⇔ roots are imaginary. A quadratic equation can be solved to get two x values or the problem's two roots. the roots of the quadratic equation can be found using one of four ways. the following are the four ways of solving quadratic equations. factoring of quadratic equation. formula method of finding roots. method of completing the square. graphing method to find the. • quadratic formula : if b2 – 4ac ≥ 0, then the real roots of the quadratic equation ax2 bx c = 0 are given by 2 4 22 −− ± b b ac aa. • the expression b2 – 4ac is called the discriminant of the quadratic equation. • existence of roots of a quadratic equation: a quadratic equation ax2 bx c=0 has quadratic equations chapter 4.
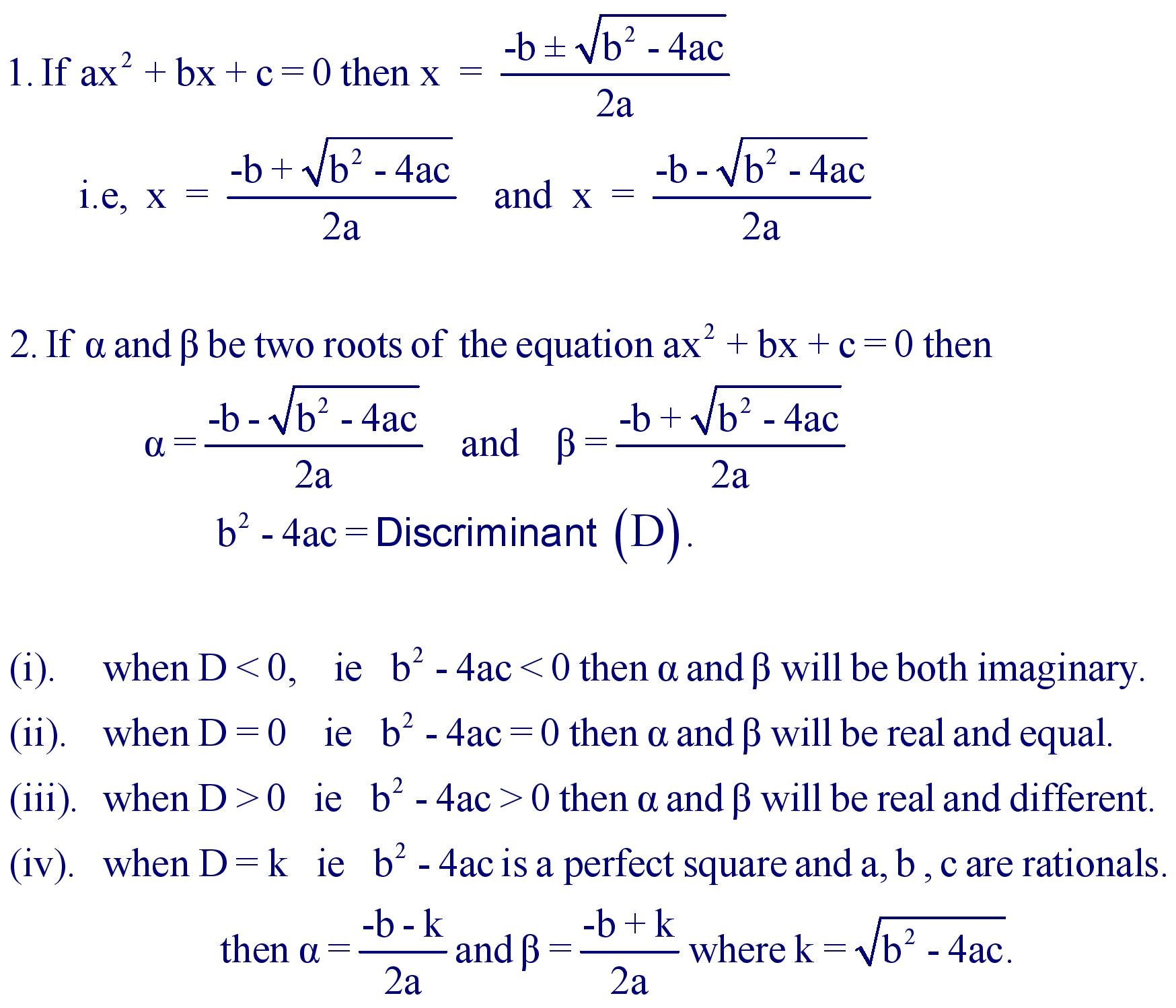
Quadratic Equations Formula For 11th Class Formula In Maths A quadratic equation can be solved to get two x values or the problem's two roots. the roots of the quadratic equation can be found using one of four ways. the following are the four ways of solving quadratic equations. factoring of quadratic equation. formula method of finding roots. method of completing the square. graphing method to find the. • quadratic formula : if b2 – 4ac ≥ 0, then the real roots of the quadratic equation ax2 bx c = 0 are given by 2 4 22 −− ± b b ac aa. • the expression b2 – 4ac is called the discriminant of the quadratic equation. • existence of roots of a quadratic equation: a quadratic equation ax2 bx c=0 has quadratic equations chapter 4.
