
Answered A Charge Is Uniformly Distributed In A Spherical Volume O A negative charge q is uniformly distributed throughout the spherical volume of radius r as shown above. a positive point charge q is placed at the center of the sphere. A thin spherical shell of radius a has a charge q evenly distributed over its surface. find the electric field both inside and outside the shell. solution: step 1: the charge distribution is spherically symmetric. step 2: since q is uniformly distributed on the shell, the electric field must be radially symmetric and directed outward.

A Charge Q Is Distributed Uniformly Throughout A Spherical Volume Of If the charge is uniformly distributed throughout the sphere, this is just \(\frac{q r}{4\pi\epsilon 0 r}\). here \(q r\) is the charge contained within radius \(r\), which, if the charge is uniformly distributed throughout the sphere, is \(q(r^3 a^3)\). A charge ` q` is uniformly distributed in a spherical volume of radius r. a particle of charge ` q` and mass m projected with velocity `v 0` the surface of the sherical volume to its centre inside a smooth tunnel dug across the sphere. the minimum value of `v 0` such tht it just reaches the centre (assume that thee is no resistance on the. A particle of charge q and mass m projected with velocity v 0 from the surface of the spherical volume to its centre inside a smooth tunnel dug across the sphere. the minimum value of v 0 such that it just reaches the centre (assume that there is no resistance on the particle except electrostatic force) of the spherical volume is. A positive charge q is uniformly distributed throughout the volume of a dielectric sphere of radius r. a point mass having charge q and mass misfired towards the centre of the sphere with velocity v from a point at distance r (r >r) from the centre of the sphere. find the minimum velocity v so that it can penetrate r 2 distance of the sphere.

Solved Charge Q Is Uniformly Distributed In A Sphere Of Chegg A particle of charge q and mass m projected with velocity v 0 from the surface of the spherical volume to its centre inside a smooth tunnel dug across the sphere. the minimum value of v 0 such that it just reaches the centre (assume that there is no resistance on the particle except electrostatic force) of the spherical volume is. A positive charge q is uniformly distributed throughout the volume of a dielectric sphere of radius r. a point mass having charge q and mass misfired towards the centre of the sphere with velocity v from a point at distance r (r >r) from the centre of the sphere. find the minimum velocity v so that it can penetrate r 2 distance of the sphere. A charge q, is uniformly distributed within a sphere of radius r. find the electric field, due to this charge distribution, at a point distant r from the centre of the sphere where : (i) 0 < r < r (ii) r > r. A non conducting sphere has a total charge q uniformly distributed throughout its volume. the centre of the sphere is at origin and its radius is r. let u 1 be the electrostatic potential energy in the region inside the sphere and u 2 be the electrostatic potential energy in another imaginary spherical shell, having inner radius r and outer. The total charge \(q\) is uniformly distributed across the volume of the sphere with radius \(a\). the volume of the sphere is \(\frac{4}{3}\pi a^3\). then, since \(n = q v\), the number density \(n\) is \(\frac{3q}{4\pi a^3}\). this is a key piece in calculating the current density \(j\). A charge q is uniformly distributed throughout the volume of a spherical shell that has an inner radius a and an outer radius b. determine the electric field everywhere.
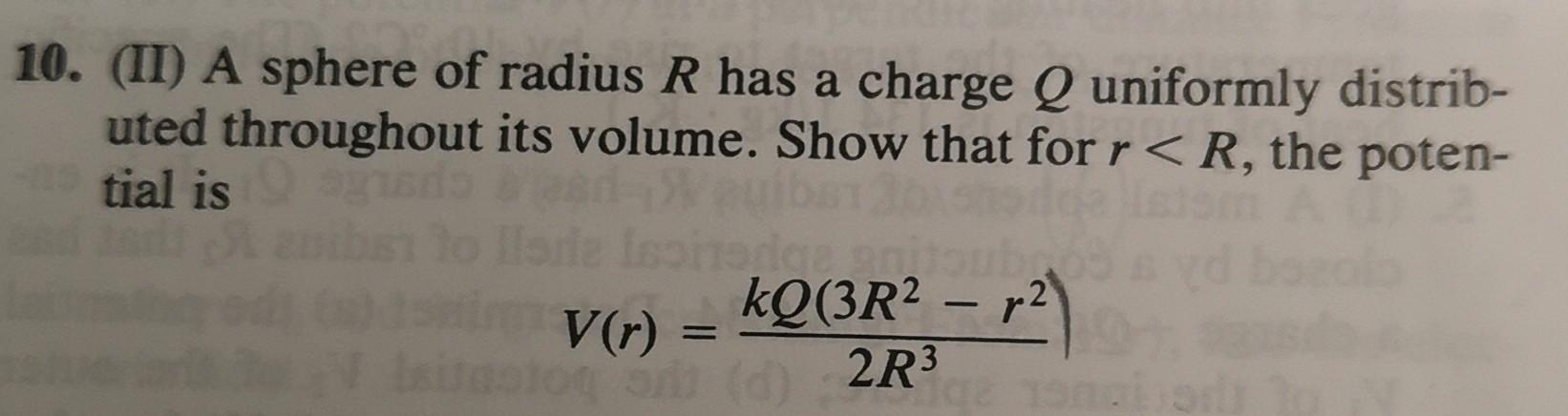
Solved 10 Ii A Sphere Of Radius R Has A Charge Q Chegg A charge q, is uniformly distributed within a sphere of radius r. find the electric field, due to this charge distribution, at a point distant r from the centre of the sphere where : (i) 0 < r < r (ii) r > r. A non conducting sphere has a total charge q uniformly distributed throughout its volume. the centre of the sphere is at origin and its radius is r. let u 1 be the electrostatic potential energy in the region inside the sphere and u 2 be the electrostatic potential energy in another imaginary spherical shell, having inner radius r and outer. The total charge \(q\) is uniformly distributed across the volume of the sphere with radius \(a\). the volume of the sphere is \(\frac{4}{3}\pi a^3\). then, since \(n = q v\), the number density \(n\) is \(\frac{3q}{4\pi a^3}\). this is a key piece in calculating the current density \(j\). A charge q is uniformly distributed throughout the volume of a spherical shell that has an inner radius a and an outer radius b. determine the electric field everywhere.
