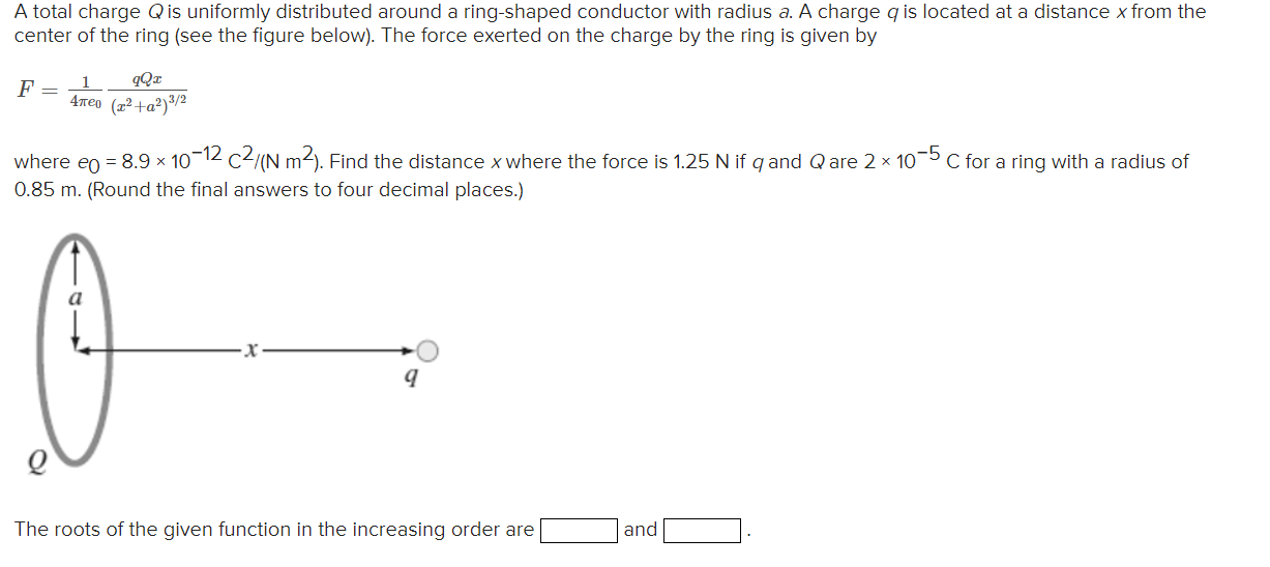
Solved A Total Charge Q Is Uniformly Distributed Around A Chegg A charge q is located at a distance x from the center of the ring (fig. p7.31). the force exerted on the charge by the ring is given by i qqx f (x2 a2)3 2 where e = 8.85 x 10 12 c (n m²), q = q = 2 x 10 5 c, and a = 0.9 m. A total charge \(q\) is uniformly distributed around a ring shaped conductor with radius a. a charge \(q\) is located at a distance \(x\) from the center of the ring (fig. \(p 8.31\) ). the force exerted on the charge by the ring is given by $$f=\frac{1}{4 \pi e {0}} \frac{q q {x}}{\left(x^{2} a^{2}\right)^{3 2}}$$ where \(e {0}=8.85 \times.
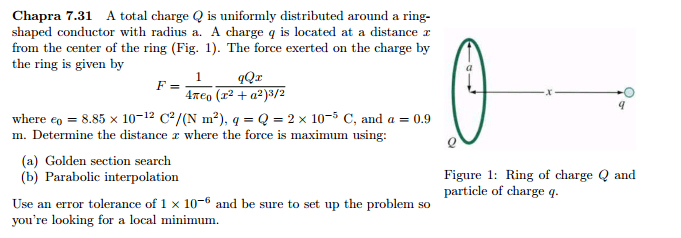
A Total Charge Q Is Uniformly Distributed Around A Chegg To find the electric field at a point p on the axis of a ring of charge, we can use the principle of superposition and symmetry. here are the steps: consider a small element of charge $$dq$$dq on the ring. the charge $$q$$q is uniformly distributed around the ring, so the linear charge density $$\lambda$$λ is given by:. $ \mathrm{a}$ total charge $q$ is uniformly distributed around a ringshaped conductor with radius $a$. a charge $q$ is located at a distance $x$ from the center of the ring (fig. $\mathrm{p} 8.31$ ). the force exerted on the charge by the ring is given by \[f=\frac{1}{4 \pi e {0}} \frac{q q x}{\left(x^{2} a^{2}\right)^{3 2}}\]. A total charge q is uniformly distributed around a ring shaped conductor with radius. a charge q is located at a distance x form the center of the ring. the force a exerted on the charge by the ring is given by 1 q q x f 41 e. (x2 a2) 2 where e, = 8.9 x 10 12 c2 nm2. In this case, the charge q is uniformly distributed along a semicircular thread, meaning that the charge density is constant. understanding this distribution is crucial for calculating the electric potential, as the contribution to the potential from each infinitesimal segment of the thread must be integrated over the entire semicircle.
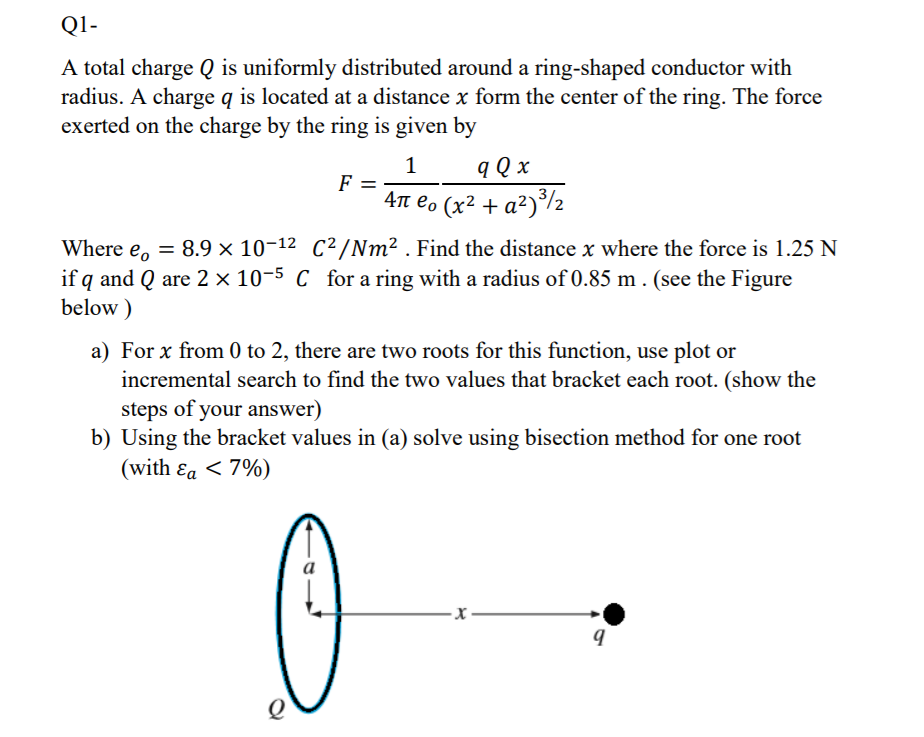
Solved Q1 A Total Charge Q Is Uniformly Distributed Around Chegg A total charge q is uniformly distributed around a ring shaped conductor with radius. a charge q is located at a distance x form the center of the ring. the force a exerted on the charge by the ring is given by 1 q q x f 41 e. (x2 a2) 2 where e, = 8.9 x 10 12 c2 nm2. In this case, the charge q is uniformly distributed along a semicircular thread, meaning that the charge density is constant. understanding this distribution is crucial for calculating the electric potential, as the contribution to the potential from each infinitesimal segment of the thread must be integrated over the entire semicircle. In our case, the charge is uniformly distributed in a spherical volume, leading to a uniform charge density \( \rho \). the charge density \( \rho \) is calculated by dividing the total charge \( q \) by the volume \( v \) of the sphere, giving \( \rho = \frac{3q}{4\pi r^{3}} \). A solid sphere of radius r contains a total charge q distributed uniformly throughout its volume. find the energy needed to assemble this charge by bringing infinitesimal charges from far away. this energy is called the “self energy” of the charge distribution. A total charge q is uniformly distributed around a ring shaped conductor with radius a. a charge is located at a distance from the center of the ring (see figure). o the force exerted on the charge by the ring is given by f 1 ames ( a) where ep = 8.85 x 10 12 cz (n m2, q= q = 2 x 10 5c, and a = 1.5 m. A total charge $q$ is uniformly distributed around a ringshaped conductor with radius $a$. a charge $q$ is located at a distance $x$ from the center of the ring (fig. $p 16.23$ ). the force exerted on the charge by the ring is given by \[f=\frac{1}{4 \pi e {0}} \frac{q q x}{\left(x^{2} a^{2}\right)^{3 2}}\].
