Solved Use The Ratio Test Or The Root Test To Investigate Chegg For example \(\sum {n=1}^\infty ( 1)^{n 1} {1\over n^2}\) converges absolutely, while \(\sum {n=1}^\infty ( 1)^{n 1} {1\over n}\) converges conditionally. 11.6 absolute convergence and the ratio and root tests the most common way to test for convergence is to ignore any positive or negative signs in a se ries, and simply test the corresponding series of positive terms. does it seem reasonable that the convergence of the series ¥ å n=1 n 3n = 1 3 2 9 3 27 4 81 5 243 6 729.

Mths221 Note 18 The Ratio Test Root Test And Absolute And As with the ratio test this test will also tell whether a series is absolutely convergent or not rather than simple convergence. suppose that we have the series ∑an ∑ a n. define, then, if l <1 l <1 the series is absolutely convergent (and hence convergent). if l> 1 l> 1 the series is divergent. Test the following series for convergence p1 nn n=1 n!. Use the ratio test to determine absolute convergence of a series. use the root test to determine absolute convergence of a series. describe a strategy for testing the convergence of a given series. in this section, we prove the last two series convergence tests: the ratio test and the root test. Example: determine if the series is absolute convergent, conditionally convergent, or divergent? lim ian i lim lim by the root test the series will diverge. the root test: (not covered in this course but is in the textbook) (a) if lim n i l, with 0 < l < 1 then the series an is absolutely con 00, then the series an is divergent.

Solved Test The Series Below For Convergence Using The Ro Chegg Use the ratio test to determine absolute convergence of a series. use the root test to determine absolute convergence of a series. describe a strategy for testing the convergence of a given series. in this section, we prove the last two series convergence tests: the ratio test and the root test. Example: determine if the series is absolute convergent, conditionally convergent, or divergent? lim ian i lim lim by the root test the series will diverge. the root test: (not covered in this course but is in the textbook) (a) if lim n i l, with 0 < l < 1 then the series an is absolutely con 00, then the series an is divergent. For series involving both powers and factorials use the ratio test (rt). example: use the root test to determine if the following series diverges or converges. Math 152 copyright joe kahlig, 21a page 3 the ratio test: (a) if lim n!1 n a n 1 a n = l, with 0 l < 1, then the series x1 n=1 a is absolutely convergent (and therefore convergent). (b) if lim n!1 a n 1 a n n= l > 1 or lim n!1 a n 1 a n = 1, then the series x1 n=1 a is divergent. note: if the limit for the ratio test is 1, then this test fails. 2n 5 3n converges absolutely by the ratio test. example5: use the ratio test to decide whether the series x∞ n=1 (2n)! (n!)2 converges absolutely, is conditionally convergent or diverges. lim n→∞ a n 1 a n = lim n→∞ (2(n 1))! ((n 1)!)2 · (n!)2 (2n)! = lim n→∞ (2n 2)! (n 1)!·(n 1)! · n!·n! (2n)! = lim n→∞ (2n 2)·(2n 1. 11.6: absolute convergence and the ratio and root tests definition: a series an is called absolutely convergent if the series |an| = |a1| |a2| |an| is convergent. if a series is convergent but not absolutely convergent, it is called conditionally convergent.

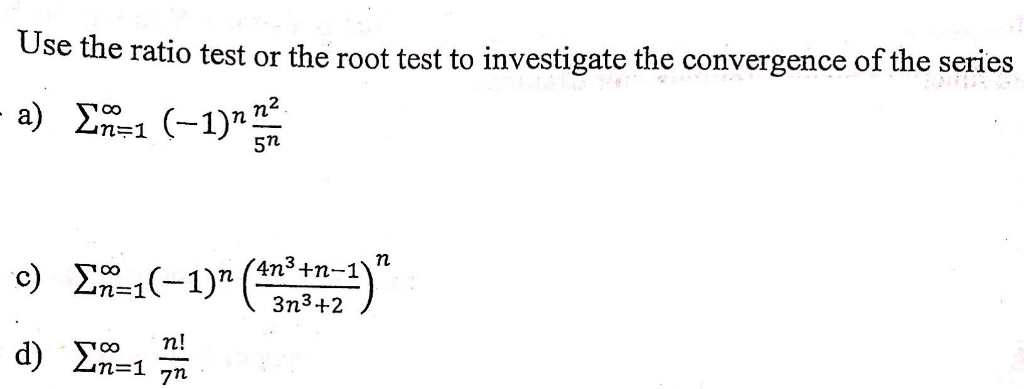
Solved 1 Use The Ratio Test Or The Root Test To Investigate Chegg For series involving both powers and factorials use the ratio test (rt). example: use the root test to determine if the following series diverges or converges. Math 152 copyright joe kahlig, 21a page 3 the ratio test: (a) if lim n!1 n a n 1 a n = l, with 0 l < 1, then the series x1 n=1 a is absolutely convergent (and therefore convergent). (b) if lim n!1 a n 1 a n n= l > 1 or lim n!1 a n 1 a n = 1, then the series x1 n=1 a is divergent. note: if the limit for the ratio test is 1, then this test fails. 2n 5 3n converges absolutely by the ratio test. example5: use the ratio test to decide whether the series x∞ n=1 (2n)! (n!)2 converges absolutely, is conditionally convergent or diverges. lim n→∞ a n 1 a n = lim n→∞ (2(n 1))! ((n 1)!)2 · (n!)2 (2n)! = lim n→∞ (2n 2)! (n 1)!·(n 1)! · n!·n! (2n)! = lim n→∞ (2n 2)·(2n 1. 11.6: absolute convergence and the ratio and root tests definition: a series an is called absolutely convergent if the series |an| = |a1| |a2| |an| is convergent. if a series is convergent but not absolutely convergent, it is called conditionally convergent.

Solved Use The Root Test To Determine The Convergence Or Chegg 2n 5 3n converges absolutely by the ratio test. example5: use the ratio test to decide whether the series x∞ n=1 (2n)! (n!)2 converges absolutely, is conditionally convergent or diverges. lim n→∞ a n 1 a n = lim n→∞ (2(n 1))! ((n 1)!)2 · (n!)2 (2n)! = lim n→∞ (2n 2)! (n 1)!·(n 1)! · n!·n! (2n)! = lim n→∞ (2n 2)·(2n 1. 11.6: absolute convergence and the ratio and root tests definition: a series an is called absolutely convergent if the series |an| = |a1| |a2| |an| is convergent. if a series is convergent but not absolutely convergent, it is called conditionally convergent.

Use The Root Test To Determine The Convergence Or Chegg
