Absolute Vs Conditional Convergence Iacedcalculus Conditional convergence is a type of convergence in which the sum of a series converges, but the sum of the absolute values of the terms does not. a classic example is the series $\displaystyle\sum {n=1}^\infty ( 1)^ {n 1}\cdot\dfrac {1} {n}$. In this section, we discuss one way to characterise this sort of delicate convergence — especially in the presence of changes of sign. converges. is conditionally convergent. if you consider these definitions for a moment, it should be clear that absolute convergence is a stronger condition than just simple convergence.

Absolute Vs Conditional Convergence By Solomon Xie Calculus Basics In summary, absolute convergence is a stronger condition, ensuring the series converges regardless of term signs, while conditional convergence relies on the alternating nature of the series and can fail if the terms are not arranged correctly. understanding both is crucial for analyzing the convergence behavior of infinite series. First, let’s go back over the definition of absolute convergence. a series ∑an ∑ a n is called absolutely convergent if ∑|an| ∑ | a n | is convergent. if ∑an ∑ a n is convergent and ∑|an| ∑ | a n | is divergent we call the series conditionally convergent. we also have the following fact about absolute convergence. In this section we are going to take a look at a test that we can use to see if a series is absolutely convergent or not. recall that if a series is absolutely convergent then we will also know that it's convergent and so we will often use it to simply determine the convergence of a series. ratio test suppose we have the series p an. de ne. I.e., absolutely convergent series are convergent.

Absolute Vs Conditional Convergence By Solomon Xie Calculus Basics In this section we are going to take a look at a test that we can use to see if a series is absolutely convergent or not. recall that if a series is absolutely convergent then we will also know that it's convergent and so we will often use it to simply determine the convergence of a series. ratio test suppose we have the series p an. de ne. I.e., absolutely convergent series are convergent. 10.9 determining absolute or conditional convergence: next lesson. packet. calc 10.9 packet.pdf: file size: 248 kb: file type: pdf: download file. want to save money on printing? support us and buy the calculus workbook with all the packets in one nice spiral bound book. solution manuals are also available. To decide whether it converges absolutely, we look at the series of absolute values, x∞ k=1 1 √ k. this is a p series with p = 1 2, and we know that diverges. so, x∞ k=1 (−1)k √ k converges conditionally . (b) x∞ k=1 sink k!. solution. this series is not an alternating series, so we should not even try the alternating series test. One unique thing about series with positive and negative terms (including alternating series) is the question of absolute or conditional convergence. once convergence of the series is established, then determining the convergence of the absolute value of the series tells you whether it converges absolutely or conditionally. There are two typical tools which can be used: absolute convergence (which implies convergence) and leibniz’s theorem. let us begin with introducing the notion of abso lute convergence. it can be seen that it is similar to absolute convergence of improper integrals. definition 1. we say that the series ∑1 ∑ n=1 an converges absolutely if.
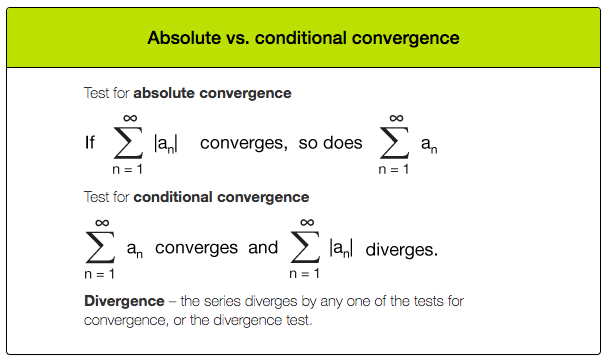
Absolute Vs Conditional Convergence By Solomon Xie Calculus Basics 10.9 determining absolute or conditional convergence: next lesson. packet. calc 10.9 packet.pdf: file size: 248 kb: file type: pdf: download file. want to save money on printing? support us and buy the calculus workbook with all the packets in one nice spiral bound book. solution manuals are also available. To decide whether it converges absolutely, we look at the series of absolute values, x∞ k=1 1 √ k. this is a p series with p = 1 2, and we know that diverges. so, x∞ k=1 (−1)k √ k converges conditionally . (b) x∞ k=1 sink k!. solution. this series is not an alternating series, so we should not even try the alternating series test. One unique thing about series with positive and negative terms (including alternating series) is the question of absolute or conditional convergence. once convergence of the series is established, then determining the convergence of the absolute value of the series tells you whether it converges absolutely or conditionally. There are two typical tools which can be used: absolute convergence (which implies convergence) and leibniz’s theorem. let us begin with introducing the notion of abso lute convergence. it can be seen that it is similar to absolute convergence of improper integrals. definition 1. we say that the series ∑1 ∑ n=1 an converges absolutely if.

Absolute Vs Conditional Convergence By Solomon Xie Calculus Basics One unique thing about series with positive and negative terms (including alternating series) is the question of absolute or conditional convergence. once convergence of the series is established, then determining the convergence of the absolute value of the series tells you whether it converges absolutely or conditionally. There are two typical tools which can be used: absolute convergence (which implies convergence) and leibniz’s theorem. let us begin with introducing the notion of abso lute convergence. it can be seen that it is similar to absolute convergence of improper integrals. definition 1. we say that the series ∑1 ∑ n=1 an converges absolutely if.

Ppt Absolute Vs Conditional Convergence Powerpoint Presentation
