
Abstract Algebra Pdf Group Mathematics Group Theory In abstract algebra, the symmetric group defined over any set is the group whose elements are all the bijections from the set to itself, and whose group operation is the composition of functions. in particular, the finite symmetric group defined over a finite set of symbols consists of the permutations that can be performed on the symbols. [1] . This videos defines the symmetric group and discusses the relationship of the symmetric group to the symmetries of an equilateral triangle and the symmetries.
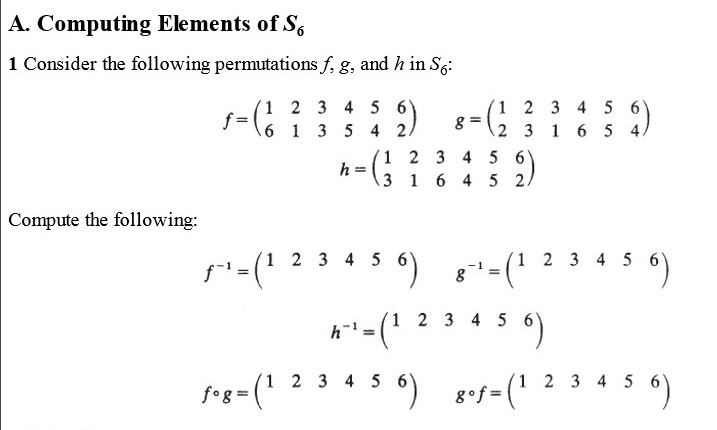
Solved Group Theory Abstract Algebra Please Solve Only Chegg There are two concepts which are very similar literally in abstract algebra: symmetric group and symmetry group. by definition, the symmetric group on a set is the group consisting of all bijections of the set (all one to one and onto functions) from the set to itself with function composition as the group operation. A symmetric group is the collection of all n! permutations of n objects. we will study permutations, and how to write them concisely in cycle notation. cayley’s theorem tells us that every nite group is isomorphic to a collection of permutations (i.e., asubgroupof a symmetric group). sec 2.3 symmetric and alternating groups abstract algebra i. Sn with compositions forms a group; this group is called a symmetric group. sn is a finite group of order n! and are permutation groups consisting of all possible permutations of n objects. identity permutation is denoted as e. we will denote a permutation by σ = [1 2 3 ⋯ n σ(1) σ(2) σ(3) ⋯ σ(n)] ∈ sn. let σ = [1 2 3 4 5 2 4 3 5 1] ∈ s5 and. Let x be a set. then the symmetric group on x is the group of all bijections. with functional composition as operation. it is denoted by sx . are each other's inverses; thus, they are bijections. furthermore, these assignments respect the group operations of sx and sy, showing these two groups to be isomorphic.

Solved 1 In Abstract Algebra Mostly Groups Rings And Chegg Sn with compositions forms a group; this group is called a symmetric group. sn is a finite group of order n! and are permutation groups consisting of all possible permutations of n objects. identity permutation is denoted as e. we will denote a permutation by σ = [1 2 3 ⋯ n σ(1) σ(2) σ(3) ⋯ σ(n)] ∈ sn. let σ = [1 2 3 4 5 2 4 3 5 1] ∈ s5 and. Let x be a set. then the symmetric group on x is the group of all bijections. with functional composition as operation. it is denoted by sx . are each other's inverses; thus, they are bijections. furthermore, these assignments respect the group operations of sx and sy, showing these two groups to be isomorphic. In general, the symmetric group on n n objects is the set of permutations that rearranges the n n objects. the group operation is composition of permutations. let’s be a little more formal. a permutation of a set a a is a function σ: a → a σ: a → a that is both one to one and onto. The symmetric group of degree n, denoted s n , is the group whose elements are p.29 the permutations on the set f1;:::;ng, and the binary operation is function composition . Motivation: cayley's theorem says that every nite group is isomorphic to a collection of permutations (i.e., a subgroup of a symmetric group). a group is cyclic if it can be generated by a single element. a possible generator is counterclockwise rotation by 2 =n, where n is the number of \arms.". Introduction (0:00) definition of symmetric group (0:18) cauchy’s notation (5:52) s n is non abelian for n at least 3 (9:50) cycle notation (11:21) disjoint cycles (18:02) cycle decomposition.

Abstract Algebra Part 2 Group Theory Example 2 4 Let G Z 12 Let S In general, the symmetric group on n n objects is the set of permutations that rearranges the n n objects. the group operation is composition of permutations. let’s be a little more formal. a permutation of a set a a is a function σ: a → a σ: a → a that is both one to one and onto. The symmetric group of degree n, denoted s n , is the group whose elements are p.29 the permutations on the set f1;:::;ng, and the binary operation is function composition . Motivation: cayley's theorem says that every nite group is isomorphic to a collection of permutations (i.e., a subgroup of a symmetric group). a group is cyclic if it can be generated by a single element. a possible generator is counterclockwise rotation by 2 =n, where n is the number of \arms.". Introduction (0:00) definition of symmetric group (0:18) cauchy’s notation (5:52) s n is non abelian for n at least 3 (9:50) cycle notation (11:21) disjoint cycles (18:02) cycle decomposition.

Symmetric Group Pdf Abstract Algebra Algebra Motivation: cayley's theorem says that every nite group is isomorphic to a collection of permutations (i.e., a subgroup of a symmetric group). a group is cyclic if it can be generated by a single element. a possible generator is counterclockwise rotation by 2 =n, where n is the number of \arms.". Introduction (0:00) definition of symmetric group (0:18) cauchy’s notation (5:52) s n is non abelian for n at least 3 (9:50) cycle notation (11:21) disjoint cycles (18:02) cycle decomposition.
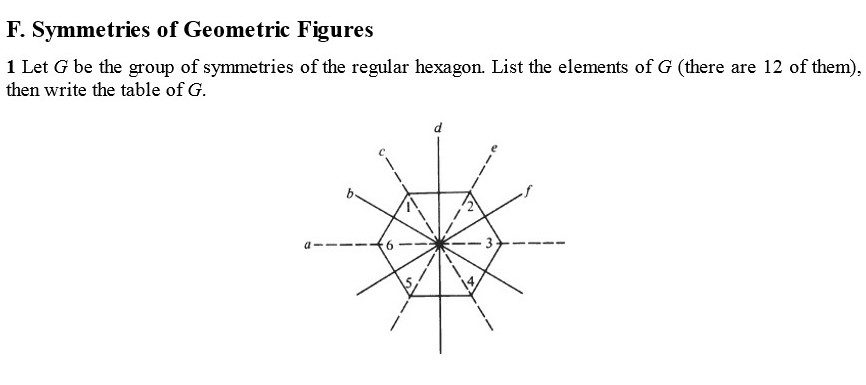
Solved Group Theory Abstract Algebra Would You Please Chegg
