
Solved Find The Nth Term Of The Arithmetic Progression That Chegg Arithmetic progression (ap) is a sequence of numbers in order that the common difference of any two successive numbers is a constant value. learn with arithmetic sequence formulas and solved examples. In this article, we will discuss the introduction to arithmetic progression (ap), general terms, and various formulas in ap, such as the sum of n terms of an ap, nth term of an ap and so on in detail. a sequence is a finite or infinite list of numbers following a specific pattern.

Solved 9 The First Term Of An Arithmetic Progression Ap Chegg Arithmetic progressions (ap) are fundamental sequences in mathematics where each term after the first is obtained by adding a constant difference to the previous term. understanding aps is crucial for solving problems related to sequences and series in class 10 mathematics. In this article, let us discuss how to find the nth term of an ap with many solved examples. assume that a 1, a 2, a 3,… be an arithmetic progression (ap), in which first term a 1 is equal to “a” and the common difference is taken as “d”, then the second term, third term, etc can be calculated as follows: second term, a 2 = a d. Formula for n th term in arithmetic progression. the formula to find the n th term in ap is a n = a (n 1)×d, where (a n) represents the nth term, 'a' denotes the first term, 'd' is the common difference, 'n' signifies the position of the term in the sequence. Let the first term of the arithmetic progression be ‘a’. then, the 8th term is given by a 7 * 3 = a 21, and the 15th term is a 14 * 3 = a 42. solving this equation, we get a = 13. now, the 10th term is a 9 * 3 = 13 27 = 40. therefore, the correct answer is not listed in the options. the correct answer is 40.
2 Find Arithmetic Progression I The 20 Th Term Of The Ap 9 13 17 21 Formula for n th term in arithmetic progression. the formula to find the n th term in ap is a n = a (n 1)×d, where (a n) represents the nth term, 'a' denotes the first term, 'd' is the common difference, 'n' signifies the position of the term in the sequence. Let the first term of the arithmetic progression be ‘a’. then, the 8th term is given by a 7 * 3 = a 21, and the 15th term is a 14 * 3 = a 42. solving this equation, we get a = 13. now, the 10th term is a 9 * 3 = 13 27 = 40. therefore, the correct answer is not listed in the options. the correct answer is 40. This expression provides the explicit formula for the \(n\) th term of an arithmetic progression. sum of n terms of a sequence in ap. the sum of the first \(n\) terms of an arithmetic progression, denoted \(s n\), is given by \(s n = \frac{n}{2}(2a (n 1)d)\), where \(a\) is the first term and \(d\) is the common difference of the progression. Answer: the 47th term is the th term of the ap. in real life scenarios, the nth term formula is used to predict outcomes based on regular patterns, such as financial growth or population changes. it is used to model patterns in nature, such as the arrangement of leaves or the growth of sequences. → general term the nth term of an ap : the nth term of an a.p is given by t n = a (n – 1)d, where a is the first term, d is the common difference and n is the number of terms. an arithmetic progression may have finite or infinite terms. infinite a.p. : 1, 2, 3, 4, 5, 6, 7, ……….

5 2 2 The Nth Term Of An Arithmetic Progression Spm Additional This expression provides the explicit formula for the \(n\) th term of an arithmetic progression. sum of n terms of a sequence in ap. the sum of the first \(n\) terms of an arithmetic progression, denoted \(s n\), is given by \(s n = \frac{n}{2}(2a (n 1)d)\), where \(a\) is the first term and \(d\) is the common difference of the progression. Answer: the 47th term is the th term of the ap. in real life scenarios, the nth term formula is used to predict outcomes based on regular patterns, such as financial growth or population changes. it is used to model patterns in nature, such as the arrangement of leaves or the growth of sequences. → general term the nth term of an ap : the nth term of an a.p is given by t n = a (n – 1)d, where a is the first term, d is the common difference and n is the number of terms. an arithmetic progression may have finite or infinite terms. infinite a.p. : 1, 2, 3, 4, 5, 6, 7, ……….
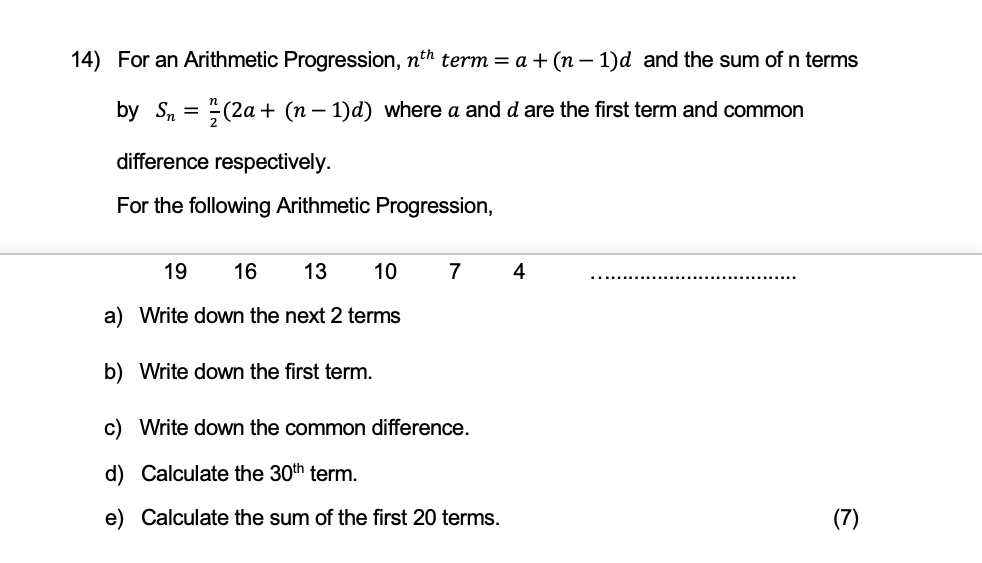
Solved 14 For An Arithmetic Progression Nth Term A N Chegg → general term the nth term of an ap : the nth term of an a.p is given by t n = a (n – 1)d, where a is the first term, d is the common difference and n is the number of terms. an arithmetic progression may have finite or infinite terms. infinite a.p. : 1, 2, 3, 4, 5, 6, 7, ……….
