
Pdf On Cubic Equations With Zero Sums Of Cubic Roots Of Roots Finding the sum and product of the roots of a cubic equations: an equation in which at least one term is raised to the power of 3 but no term is raised to any higher power is called a cubic equation. the general form of a cubic equation is ax 3 bx 2 cx d = 0 where a, b, c and d are constants and a ≠ 0. Put simply: a root is the x value where the y value equals zero. if we have a general polynomial like this: then: it works on linear, quadratic, cubic and higher! it can sometimes help us solve things. how does this magic work? let's discover we can take a polynomial, such as: and then factor it like this: f (x) = a (x−p) (x−q) (x−r).

Cubes And Cube Roots Pdf Volume Factorization How can i find sums and products of related roots? a) given a polynomial equation of order 5 (a quintic); , make 5 conjectures linking the coefficients a, b, c, d, e, f to its roots . b) test your conjectures on the example: which has roots . unlock more, it's free!. •explain why cubic equations possess either one real root or three real roots •use synthetic division to locate roots when one root is known •find approximate solutions by drawing a graph. In algebra, a cubic equation in one variable is an equation of the form in which a is not zero. the solutions of this equation are called roots of the cubic function defined by the left hand side of the equation. The ability to determine the number of real roots of a cubic equation using the sums and products method is a valuable skill in mathematics and its applications. by following the steps outlined in this guide, you can confidently analyze cubic equations and understand their real root count, contributing to problem solving across various domains.

Roots Of Cubic Equations In algebra, a cubic equation in one variable is an equation of the form in which a is not zero. the solutions of this equation are called roots of the cubic function defined by the left hand side of the equation. The ability to determine the number of real roots of a cubic equation using the sums and products method is a valuable skill in mathematics and its applications. by following the steps outlined in this guide, you can confidently analyze cubic equations and understand their real root count, contributing to problem solving across various domains. I have an equation, $x^3 x^2 x 2$, with three distinct roots, $p$, $q$ and $r$. what is the value of $p^3 q^3 r^3$? i'm not sure how to do this. using vieta's formula, we know that: $pqr= 2$ $pq. This is how we find the sum and product of roots. a cubic expression is an algebraic equation of 3rd degree. a standard form of a cubic function is: f (x) = ax3 bx2 cx1 d. and the cubic equation contains the form of ax3 bx2 cx d = 0, in which a, b and c are the coefficients and d is the constant. $\begingroup$ you could use this to solve for the individual roots using the cubic function formula. or you could just factor by grouping: $s^3 3s^2 6s 18 = s^2(s 3) 6(s 3) = (s 3)(s^2 6),$ whose roots are found from $s 3 = 0$ and $s^2 6 = 0.$ $\endgroup$ –. Cubic equation calculator solves cubic equations or 3rd degree polynomials. uses the cubic formula to solve third order polynomials for real and complex solutions.
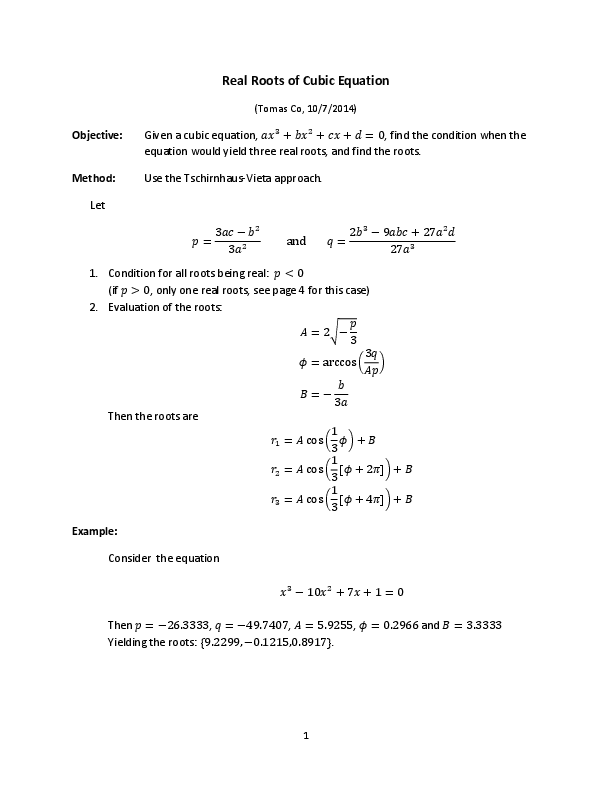
Pdf Real Roots Of Cubic Equation I have an equation, $x^3 x^2 x 2$, with three distinct roots, $p$, $q$ and $r$. what is the value of $p^3 q^3 r^3$? i'm not sure how to do this. using vieta's formula, we know that: $pqr= 2$ $pq. This is how we find the sum and product of roots. a cubic expression is an algebraic equation of 3rd degree. a standard form of a cubic function is: f (x) = ax3 bx2 cx1 d. and the cubic equation contains the form of ax3 bx2 cx d = 0, in which a, b and c are the coefficients and d is the constant. $\begingroup$ you could use this to solve for the individual roots using the cubic function formula. or you could just factor by grouping: $s^3 3s^2 6s 18 = s^2(s 3) 6(s 3) = (s 3)(s^2 6),$ whose roots are found from $s 3 = 0$ and $s^2 6 = 0.$ $\endgroup$ –. Cubic equation calculator solves cubic equations or 3rd degree polynomials. uses the cubic formula to solve third order polynomials for real and complex solutions.
