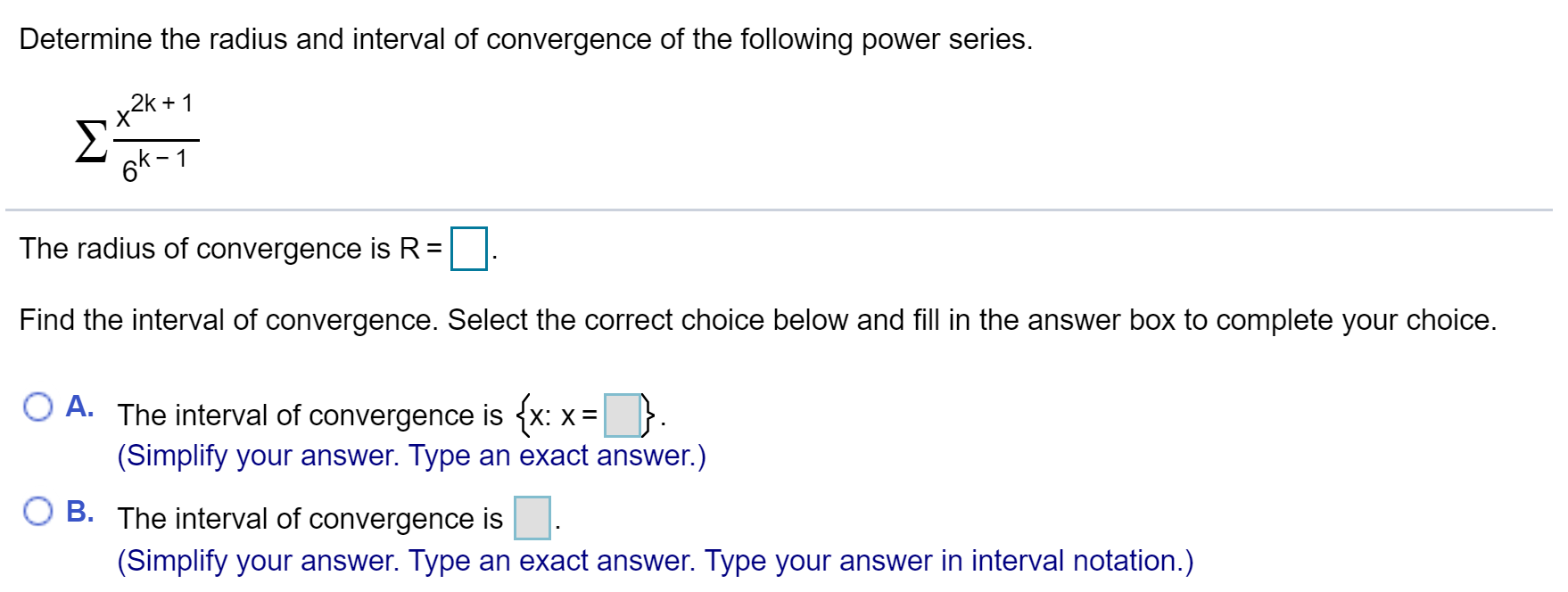
Solved Determine The Radius And Interval Of Convergence Of Chegg Free online interval of convergence calculator find power series interval of convergence step by step. In this section we will give the definition of the power series as well as the definition of the radius of convergence and interval of convergence for a power series. we will also illustrate how the ratio test and root test can be used to determine the radius and interval of convergence for a power series.
Solved Interval And Radius Of Convergence Determine The Radius Of Determine the radius and interval of convergence for each of the following power series. sum {n=0}^inftyfrac{2^n(x 3)^n}{sqrt{n 3}} mylo o'moore . answered question. 2021 03 08. Solution to calculus and analysis question: determine the radius and interval of convergence for each of the following power series.\sum {n=0}^\infty\frac {2^. For each of the following power series, find the radius of convergence and determine the exact interval of convergence. (a) sigma n^2 x^n (b) sigma (x n)^n (c) sigma (2^n n^2) x^n (d) sigma (n^3 3^n) x^n (e) sigma (2^n n!) x^n (f) sigma (1 (n 1)^2 2^n) x^n (g) sigma (3^n n middot 4^n) x^n (h) sigma (( 1)^n n^2 middot 4^n) x^n repeat exercise 23.1. Finding the radius of convergence to find the radius of convergence, r, you use the ratio test. step 1: let ! an=cn"x#a ( ) n and ! an 1=cn 1"x#a ( ) n 1. step 2: simplify the ratio ! an 1 an = cn1"x#a ( ) n 1 cn"x#a ( ) n = cn 1 cn "x#a ( ). step 3: compute the limit of the absolute value of this ratio as n → ∞. step 4: interpret the.

Solved Determine The Radius Of Convergence And Interval Of Chegg For each of the following power series, find the radius of convergence and determine the exact interval of convergence. (a) sigma n^2 x^n (b) sigma (x n)^n (c) sigma (2^n n^2) x^n (d) sigma (n^3 3^n) x^n (e) sigma (2^n n!) x^n (f) sigma (1 (n 1)^2 2^n) x^n (g) sigma (3^n n middot 4^n) x^n (h) sigma (( 1)^n n^2 middot 4^n) x^n repeat exercise 23.1. Finding the radius of convergence to find the radius of convergence, r, you use the ratio test. step 1: let ! an=cn"x#a ( ) n and ! an 1=cn 1"x#a ( ) n 1. step 2: simplify the ratio ! an 1 an = cn1"x#a ( ) n 1 cn"x#a ( ) n = cn 1 cn "x#a ( ). step 3: compute the limit of the absolute value of this ratio as n → ∞. step 4: interpret the. The calculator will try to find the radius and the interval of convergence of the given power series. enter a power series: if you need a binomial coefficient `c(n,k)=((n),(k))`, type binomial(n,k) . The interval of convergence can be calculated once you know the radius of convergence. first you solve the inequality | x − a | < r for x and then you check each endpoint individually. To find the interval of convergence, we simply solve |x 4|<3 for . to do this, we just take away the absolute value brackets and add r to the left side of the inequality, like this:. Definition: [interval and radius of convergence] given a power series of the form p1 n=0 a n(x )n, the set i = fx 0 2r j x1 n=0 a n(x 0 a)n convergesg is an interval centered at x = a which we call the interval of convergence for the power series. let r = (lub(fjx 0 ajjx 0 2ig) if i is bounded; 1 if i is not bounded: then r is called the.
