
Distribution Of The A A 1 A 2 Ratio B τ 1 And C τ 2 Values An example of data following a normal distribution is adult people’s heights, where intermediate heights are those around which most of the population falls. 5. exponential distribution. the exponential distribution is used to model the time between events in a process usually modeled by a poisson distribution. smaller values are usually more. To make a symmetrical distribution, set the mean of a to 2, and the mean of b to 0. then the ratios have the distribution shown in figure 4. as we move the mean of a closer to 0, the "valley" between the two peaks gets smaller and smaller. when the mean of a equals 1, the top is flat like a plateau.

Solved Let C1 X 2 2 X ã ë ë 2 0 F X C2 C 3 2 X ã ë ë 1 3 Consider random variables r and s where s either has no mass at 0 (discrete) or has support [0; 1). let g = g(r; s) = r=s. find approximations for eg and var(g) using taylor expansions of g(). where r is a remainder of smaller order than the terms in the equation. If $1 \leq a < b$, the projections down lines through the origin from $(1,a)$ and $(1,b)$ to the upper boundary of $u$ are to the points $(1 a,1)$ and $(1 b,1)$. the resulting area of the triangle is $\frac{1}{2}(\frac{1}{a} \frac{1}{b})(1 0)$. One way to do this is to rescale: let $y = t x 1$, so $x 0 x 1 > t$ is equivalent to $x 0 > y$. now $x 0$ and $y$ are independent exponential random variables with rate parameters $\alpha$ and $\alpha t$ respectively. It is called the f distribution, named after sir ronald fisher, an english statistician. the f statistic is a ratio (a fraction). there are two sets of degrees of freedom; one for the numerator and one for the denominator.

Solved The Distribution With Cxx 1 1 X If X 0 Chegg One way to do this is to rescale: let $y = t x 1$, so $x 0 x 1 > t$ is equivalent to $x 0 > y$. now $x 0$ and $y$ are independent exponential random variables with rate parameters $\alpha$ and $\alpha t$ respectively. It is called the f distribution, named after sir ronald fisher, an english statistician. the f statistic is a ratio (a fraction). there are two sets of degrees of freedom; one for the numerator and one for the denominator. For possible values of $q$ we have $q \in [\frac{\sqrt{2 2\cos(\pi 2\delta)}}{\mu \nu}, \frac{2}{\mu \nu}]$. on the page en. .org wiki ratio distribution, the ratio distribution is derived using (in my notation) $f z$ and $f v$. finding antiderivatives when $|y|$ is introduced turns out to be quite challenging, so i. Given two distributions y and x with joint probability density function f (x,y), let u=y x be the ratio distribution. then the distribution function of u is d (u) = p (u<=u) (1) = p (y<=ux|x>0) p (y>=ux|x<0) (2) = int 0^inftyint 0^ (ux)f (x,y)dydx int ( infty)^0int (ux)^0f (x,y)dydx. A ratio distribution, also called a quotient distribution, is composed of the ratio of two random variables. if two random variables are x and y, then their ratio distribution is x y. the random variables are usually independent and the probability distributions are usually known.

Solved The F Distribution Is Constructed As The Ratio Of Two Random For possible values of $q$ we have $q \in [\frac{\sqrt{2 2\cos(\pi 2\delta)}}{\mu \nu}, \frac{2}{\mu \nu}]$. on the page en. .org wiki ratio distribution, the ratio distribution is derived using (in my notation) $f z$ and $f v$. finding antiderivatives when $|y|$ is introduced turns out to be quite challenging, so i. Given two distributions y and x with joint probability density function f (x,y), let u=y x be the ratio distribution. then the distribution function of u is d (u) = p (u<=u) (1) = p (y<=ux|x>0) p (y>=ux|x<0) (2) = int 0^inftyint 0^ (ux)f (x,y)dydx int ( infty)^0int (ux)^0f (x,y)dydx. A ratio distribution, also called a quotient distribution, is composed of the ratio of two random variables. if two random variables are x and y, then their ratio distribution is x y. the random variables are usually independent and the probability distributions are usually known.
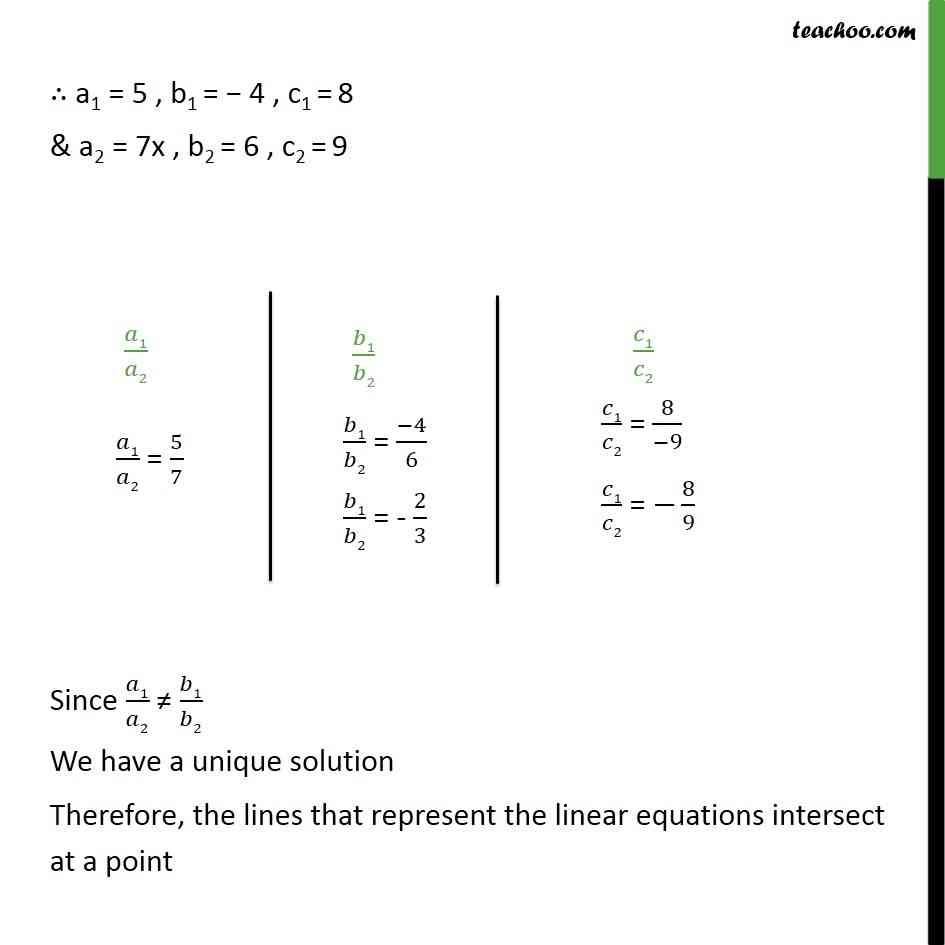
Ex 32 2 On Comparing The Ratios A1a2 B1b2 C1c2 7793 Hot Sexy Girl A ratio distribution, also called a quotient distribution, is composed of the ratio of two random variables. if two random variables are x and y, then their ratio distribution is x y. the random variables are usually independent and the probability distributions are usually known.
The Distribution Function Pt For A 1 C 1 B 2 2 X0 1 1 R
