
Solved Does Every Quadratic Equation Have Two Solutions Explain In this quick one i will show you how to obtain all the four solutions for the quantic equation here, many will only get two of the solutions.remember to lea. In mathematics, a quartic equation is one which can be expressed as a quartic function equaling zero. the general form of a quartic equation is graph of a polynomial function of degree 4, with its 4 roots and 3 critical points. where a ≠ 0. the quartic is the highest order polynomial equation that can be solved by radicals in the general case.
How To Solve Quadratic Equations The Teachers Training We will now explain how you can solve the cubic and quartic equations without the mess. given a cubic or quartic equation, we will explain how to solve it with pure thought. First, solve the cubic equation ax3 bx2 cx d where a = 1, b = −b, c = ac − 4d, d = d(4b −a2) −c2; only 1 real root is needed, call it x1. The wolfram language can solve quartic equations exactly using the built in command solve [a4 x^4 a3 x^3 a2 x^2 a1 x a0 == 0, x]. the solution can also be expressed in terms of wolfram language algebraic root objects by first issuing setoptions [roots, quartics > false]. To find all the rational and real number zeros of a higher degree polynomial. recall from the quadratic functions chapter, that every quadratic equation has two solutions. the degree of a quadratic equation is 2, thus leading us towards the notion that it has 2 solutions.

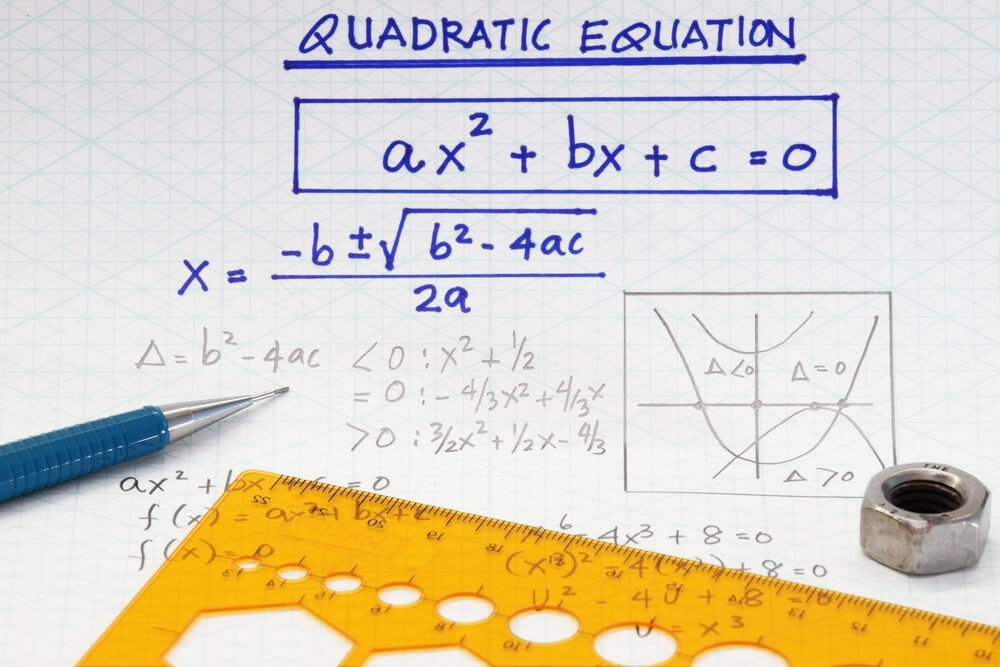
Solved When We Solve A Quadratic Equation How Many Solutions Should We The wolfram language can solve quartic equations exactly using the built in command solve [a4 x^4 a3 x^3 a2 x^2 a1 x a0 == 0, x]. the solution can also be expressed in terms of wolfram language algebraic root objects by first issuing setoptions [roots, quartics > false]. To find all the rational and real number zeros of a higher degree polynomial. recall from the quadratic functions chapter, that every quadratic equation has two solutions. the degree of a quadratic equation is 2, thus leading us towards the notion that it has 2 solutions. Use the quadratic formula to solve for the remaining two zeroes (often the complex zeroes). we will go through each step to show how they are used to solve our example quartic polynomial. 1.) calculate the number of solutions, both real and complex. this is the simplest part of the solution. This is the key to knowing how many solutions we have: if b2 4 ac is positive (>0) then we have 2 solutions. if b2 4 ac is 0 then we have only one solution as the formula is reduced to x = [ b ± 0] 2 a. so x = b 2 a, giving only one solution. lastly, if b2 4 ac is less than 0 we have no solutions. example:. If we plot the graph y = x2 px q, the solutions of the quadratic equation are where y = 0, that is, where the curve cuts the horizontal axis. the three cases i covered above—no real solutions, one real solution, two real solutions—are shown in figures cq 1, cq 2, and cq 3. If b2 −4ac>0 b 2 − 4 a c> 0 then we will get two real solutions to the quadratic equation. if b2 −4ac =0 b 2 − 4 a c = 0 then we will get a double root to the quadratic equation.
