Figure 9 Two Lines Crossing Creating Angles 1 2 3 And 4 Using The 1 identify that ∠1 and ∠4 are vertical angles, so we can use the vertical angles theorem to prove ∠1 ≅ ∠4. To determine which angle has a measure equal to 11 0 ∘, we can analyze the angles formed by the two intersecting lines. when two lines intersect, they create four angles: ∠1, ∠2, ∠3, and ∠4. a key property of intersecting lines is that vertical angles are equal.
Figure 9 Two Lines Crossing Creating Angles 1 2 3 And 4 Using The Given that a diagram shows two intersecting lines that create four angles: angles 1, 2, 3, and 4, arranged in a clockwise manner. let's evaluate each statement: a) m∠2 m∠3 = 180°: this statement is true. angles 2 and 3 are adjacent angles on a straight line, and when the lines intersect, they form a straight angle, which measures 180°. Using the subtraction property of equality, angle 2 can be subtracted from both sides of the equation, proving that the measures of angle 1 and 3 are equal. therefore, angle 1 is congruent to angle 3. if a transversal intersects two parallel lines, then corresponding angles are congruent. When two non parallel lines cross, they create an angle between them. if the lines are perpendicular, they form a 90 degree angle. otherwise, they create an acute, obtuse or other type of angle. Given: ∠1, ∠2, ∠3, and ∠4 are formed by two intersecting lines. prove: ∠1 and ∠3 are congruent. the figure shows two intersecting lines. one line rises from left to right, and the other line falls from left to right. the four angles formed, moving clockwise, are labeled as angles 1, 2, 3, and 4.
Figure 9 Two Lines Crossing Creating Angles 1 2 3 And 4 Wing Table When two non parallel lines cross, they create an angle between them. if the lines are perpendicular, they form a 90 degree angle. otherwise, they create an acute, obtuse or other type of angle. Given: ∠1, ∠2, ∠3, and ∠4 are formed by two intersecting lines. prove: ∠1 and ∠3 are congruent. the figure shows two intersecting lines. one line rises from left to right, and the other line falls from left to right. the four angles formed, moving clockwise, are labeled as angles 1, 2, 3, and 4. Two lines crossing creating angles 1, 2, 3, and 4. using the image above, complete the following table by dragging and dropping the correct pieces into the right place 1 ∠ 3 ∠ 2 4 substitution simplify vertical angles theorem transitive property division property of equality subtraction property of equality multiplication property of. Two lines crossing creating angles 1, 2, 3, and 4. How can the properties of linear pairs and vertical angles help to determine the angle measures created by the intersecting lines? explain., 2 lines intersect. a line with points t, r, w intersects a line with point s, r, v at point r. 4 angles are created. Lines jk and lm are intersected by transversal jl; the intersection of jk and jl creates angles 2, 4, 3, and 1 clockwise beginning at the top right; the intersection of lm and jl creates angles 6, 8, 7 and 5 clockwise beginning at the top right.

Chapter 9 Lines And Angles Pdf Two lines crossing creating angles 1, 2, 3, and 4. using the image above, complete the following table by dragging and dropping the correct pieces into the right place 1 ∠ 3 ∠ 2 4 substitution simplify vertical angles theorem transitive property division property of equality subtraction property of equality multiplication property of. Two lines crossing creating angles 1, 2, 3, and 4. How can the properties of linear pairs and vertical angles help to determine the angle measures created by the intersecting lines? explain., 2 lines intersect. a line with points t, r, w intersects a line with point s, r, v at point r. 4 angles are created. Lines jk and lm are intersected by transversal jl; the intersection of jk and jl creates angles 2, 4, 3, and 1 clockwise beginning at the top right; the intersection of lm and jl creates angles 6, 8, 7 and 5 clockwise beginning at the top right.
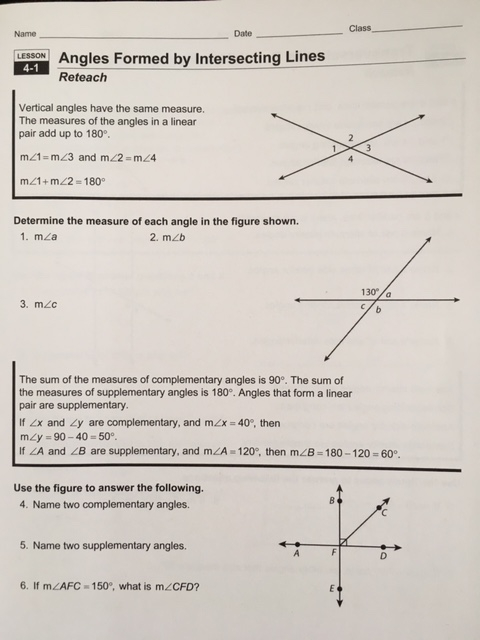
Lesson 4 1 Angles Formed By Intersecting Lines Answer Key Fill And How can the properties of linear pairs and vertical angles help to determine the angle measures created by the intersecting lines? explain., 2 lines intersect. a line with points t, r, w intersects a line with point s, r, v at point r. 4 angles are created. Lines jk and lm are intersected by transversal jl; the intersection of jk and jl creates angles 2, 4, 3, and 1 clockwise beginning at the top right; the intersection of lm and jl creates angles 6, 8, 7 and 5 clockwise beginning at the top right.
