
Linear Equations Has Infinite Solutions Tessshebaylo There are a few ways to tell when a linear system in two variables has infinite solutions: solve the system – if you solve the system and get an equation that is always true, regardless of variable value (such as 1 = 1), then there are infinite solutions. We can find whether a homogeneous linear system has a unique solution (trivial) or an infinite number of solutions (nontrivial) by using the determinant of the coefficient matrix. if a is the coefficient matrix of the system then:.

System Of Linear Equations With Infinite Solutions Diy Projects A consistent linear system of equations will have exactly one solution if and only if there is a leading 1 for each variable in the system. if a consistent linear system of equations has a free variable, it has infinite solutions. if a consistent linear system has more variables than leading 1s, then the system will have infinite solutions. A powerful tool for finding solutions to systems of equations and constraints. wolfram|alpha is capable of solving a wide variety of systems of equations. it can solve systems of linear equations or systems involving nonlinear equations, and it can search specifically for integer solutions or solutions over another domain. As you can see, the final row of the row reduced matrix consists of 0. this means that for any value of z, there will be a unique solution of x and y, therefore this system of linear equations has infinite solutions. let’s use python and see what answer we get. We can make two equations (d =distance in km, t =time in minutes) so we have a system of equations (that are linear): we can solve it on a graph: do you see how the horse starts at 6 minutes, but then runs faster? it seems you get caught after 10 minutes you only got 2 km away. run faster next time.
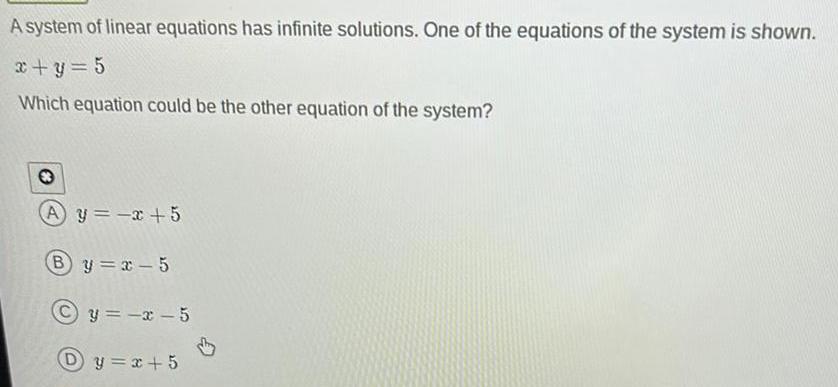
Answered A System Of Linear Equations Has Infinite Solutions One Of As you can see, the final row of the row reduced matrix consists of 0. this means that for any value of z, there will be a unique solution of x and y, therefore this system of linear equations has infinite solutions. let’s use python and see what answer we get. We can make two equations (d =distance in km, t =time in minutes) so we have a system of equations (that are linear): we can solve it on a graph: do you see how the horse starts at 6 minutes, but then runs faster? it seems you get caught after 10 minutes you only got 2 km away. run faster next time. Equations with infinite solutions. to solve systems of an equation in two or three variables, first, we need to determine whether the equation is dependent, independent, consistent, or inconsistent. if a pair of the linear equations have unique or infinite solutions, then the system of equation is said to be a consistent pair of linear. Independent: the system has exactly one unique solution. dependent: the system has infinitely many solutions. a consistent system of linear equations can have either: unique solution: this occurs when the system has exactly one solution. this is typically seen when the equations represent lines or planes that intersect at a single point. However, when a system has an infinite number of solutions, the solution is often expressed in the parametric form. this can be accomplished by assigning an arbitrary constant, t, to one of the variables, and then solving for the remaining variables. Let's look at a system of equations that has infinite solutions: 22x 11y=4414x 7y=28. try simplifying the top equation. what do you notice? 2x y=42x y=4. these are same equation! they'll both be exactly the same line on a graph. in other words, they'll overlap completely. this is easier to tell because both equations are in standard form.
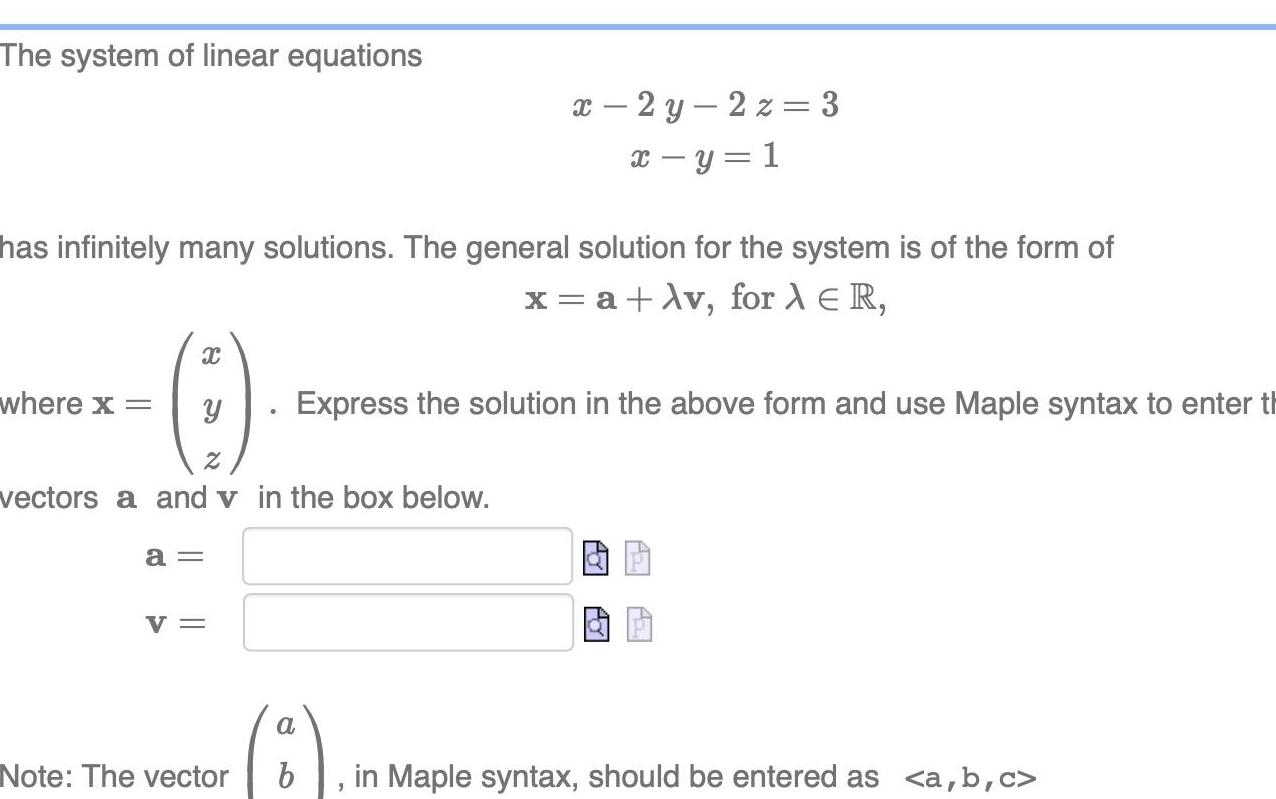
Answered The System Of Linear Equations Has Infinitely Many Solutions Equations with infinite solutions. to solve systems of an equation in two or three variables, first, we need to determine whether the equation is dependent, independent, consistent, or inconsistent. if a pair of the linear equations have unique or infinite solutions, then the system of equation is said to be a consistent pair of linear. Independent: the system has exactly one unique solution. dependent: the system has infinitely many solutions. a consistent system of linear equations can have either: unique solution: this occurs when the system has exactly one solution. this is typically seen when the equations represent lines or planes that intersect at a single point. However, when a system has an infinite number of solutions, the solution is often expressed in the parametric form. this can be accomplished by assigning an arbitrary constant, t, to one of the variables, and then solving for the remaining variables. Let's look at a system of equations that has infinite solutions: 22x 11y=4414x 7y=28. try simplifying the top equation. what do you notice? 2x y=42x y=4. these are same equation! they'll both be exactly the same line on a graph. in other words, they'll overlap completely. this is easier to tell because both equations are in standard form.
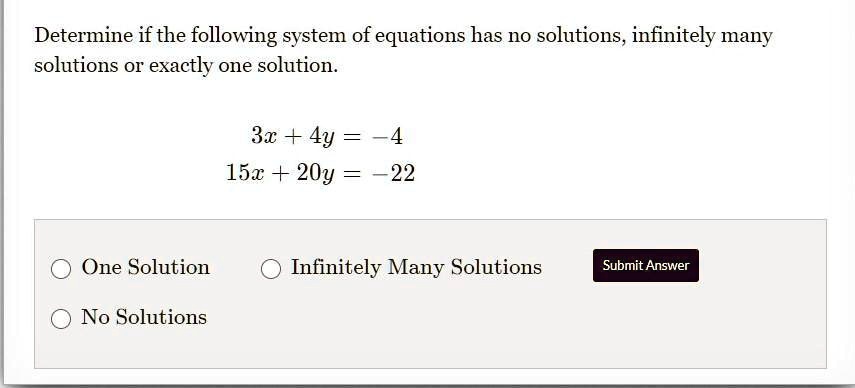
Solved Determine If The Following System Of Equations Has No However, when a system has an infinite number of solutions, the solution is often expressed in the parametric form. this can be accomplished by assigning an arbitrary constant, t, to one of the variables, and then solving for the remaining variables. Let's look at a system of equations that has infinite solutions: 22x 11y=4414x 7y=28. try simplifying the top equation. what do you notice? 2x y=42x y=4. these are same equation! they'll both be exactly the same line on a graph. in other words, they'll overlap completely. this is easier to tell because both equations are in standard form.
