
Geometric Proof Algebaic Identities Stock Vector Royalty Free Manipulating geometric shapes to carry out algebraic operations and to prove identities may be used in today's classrooms to pro vide mathematical understanding and en richment. the most elementary proof using this process involves the well known identity (a b)2 = a2 lab b2. in figure la we see a square with area (a b)2. Proving various algebraic identities like (a b)2, (a b)2, (a b c)2, a2 b2, (a b)2, (x a)(x b), (x−a)(x−b), a(b c), (b c)a using geometry.

Geometric Proof Algebraic Identities Stock Vector Royalty Free The best known geometric proof of a summation identity is probably the proof, illustrated in fig. 1, for the identity 1 3 5 7 (2n l)=n2. in this note, we exhibit a similarly striking geometric proof of the famous summation identity 13 23 33 43 n3(1 2 3 4 n)2. fi. 1. geomtric illustration of the identity 5 geometric. We give a geometric proof of the binomial identity for all natural n and real a,b. this work was inspired by the book [1], where the binomial identity for n = 3 and a,b > 0 is proved by breaking a cube c of size (a b) × (a b) × (a b) into eight rectangular boxes and counting their volumes as follows. In this article we present a geometric argument − one that does not involve induction − to establish the binomial identity for all natural and all real . n a, b in doing so, for a given natural , we first expand the above argument to prove identity (1) and then provide a geometric counting argument to show that n (a − b)n = ∑ n i = 0. Sparked by a conversation this past weekend about the usefulness of the half angle identities, i constructed geometric proofs for $latex \sin(x 2)$ and $latex \cos(x 2)$. since i've never seen these anywhere before, i thought i'd share.

Geometrical Proofs Mr Mathematics In this article we present a geometric argument − one that does not involve induction − to establish the binomial identity for all natural and all real . n a, b in doing so, for a given natural , we first expand the above argument to prove identity (1) and then provide a geometric counting argument to show that n (a − b)n = ∑ n i = 0. Sparked by a conversation this past weekend about the usefulness of the half angle identities, i constructed geometric proofs for $latex \sin(x 2)$ and $latex \cos(x 2)$. since i've never seen these anywhere before, i thought i'd share. How do you write proof in geometry? what are geometric proofs? learn to frame the structure of proof with the help of solved examples and interactive questions. Geometrical proof of identities. by using this concept of multiplication of monomials, let us try to prove the identities geometrically, which are very much useful in solving algebraic problems. 1. identity 1: (x a)(x b) = x 2 x(a b) ab. consider four regions. one region is square shaped with dimension 3 × 3 (grey). A geometric proof of an algebraic identity. published online by cambridge university press: 15 september 2017. an abstract is not available for this content so a preview has been provided. please use the get access link above for information on how to access this content. Some methods of proof of traditional algebra problems using geometric methods are explored. the techniques used come from the original greek approaches to these mathematical questions. (mp).
Intro To Geometric Proof 2 Labelled Diagram How do you write proof in geometry? what are geometric proofs? learn to frame the structure of proof with the help of solved examples and interactive questions. Geometrical proof of identities. by using this concept of multiplication of monomials, let us try to prove the identities geometrically, which are very much useful in solving algebraic problems. 1. identity 1: (x a)(x b) = x 2 x(a b) ab. consider four regions. one region is square shaped with dimension 3 × 3 (grey). A geometric proof of an algebraic identity. published online by cambridge university press: 15 september 2017. an abstract is not available for this content so a preview has been provided. please use the get access link above for information on how to access this content. Some methods of proof of traditional algebra problems using geometric methods are explored. the techniques used come from the original greek approaches to these mathematical questions. (mp).
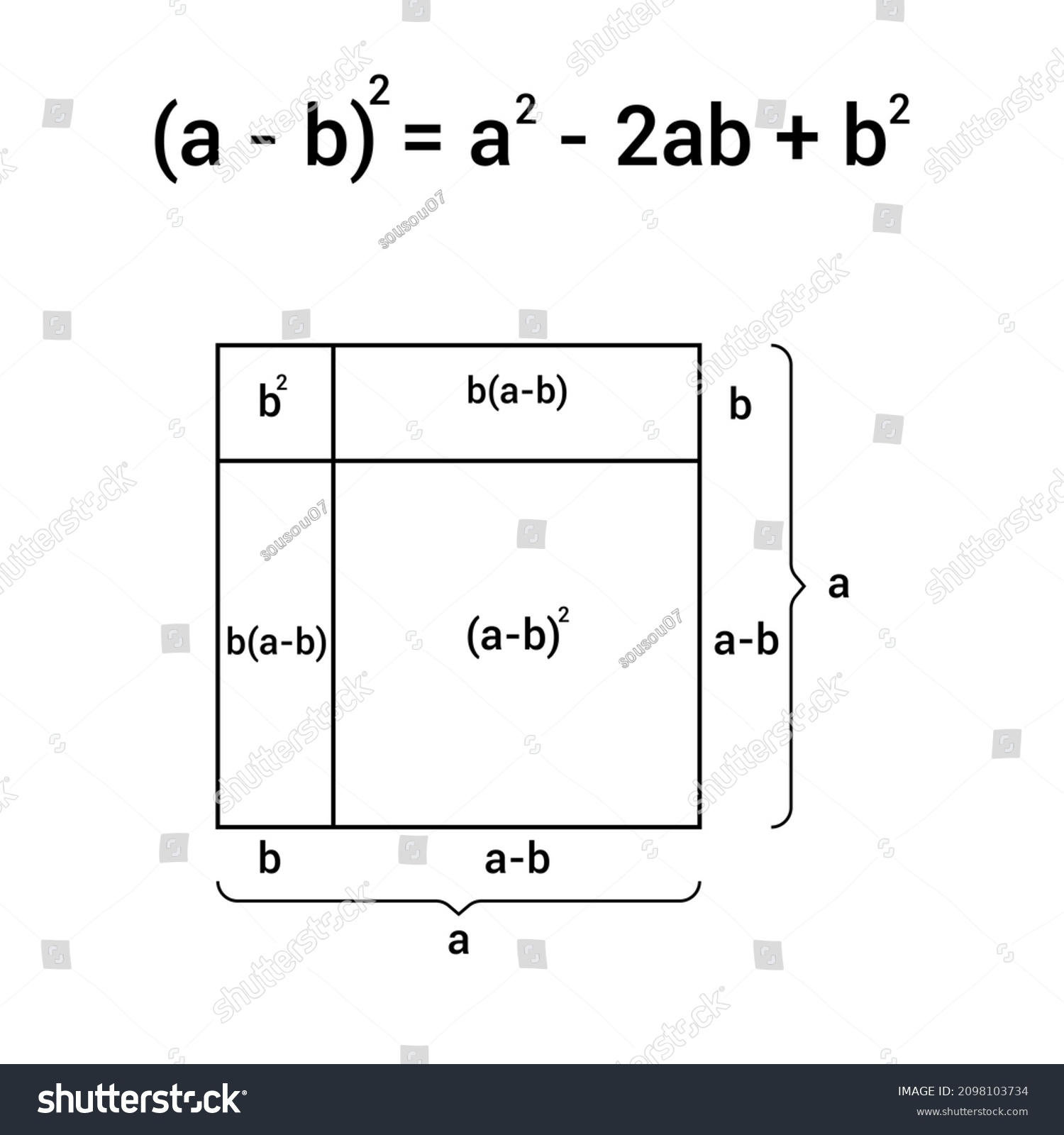
Geometric Proof Of Algebaic Identities Royalty Free Stock Vector A geometric proof of an algebraic identity. published online by cambridge university press: 15 september 2017. an abstract is not available for this content so a preview has been provided. please use the get access link above for information on how to access this content. Some methods of proof of traditional algebra problems using geometric methods are explored. the techniques used come from the original greek approaches to these mathematical questions. (mp).
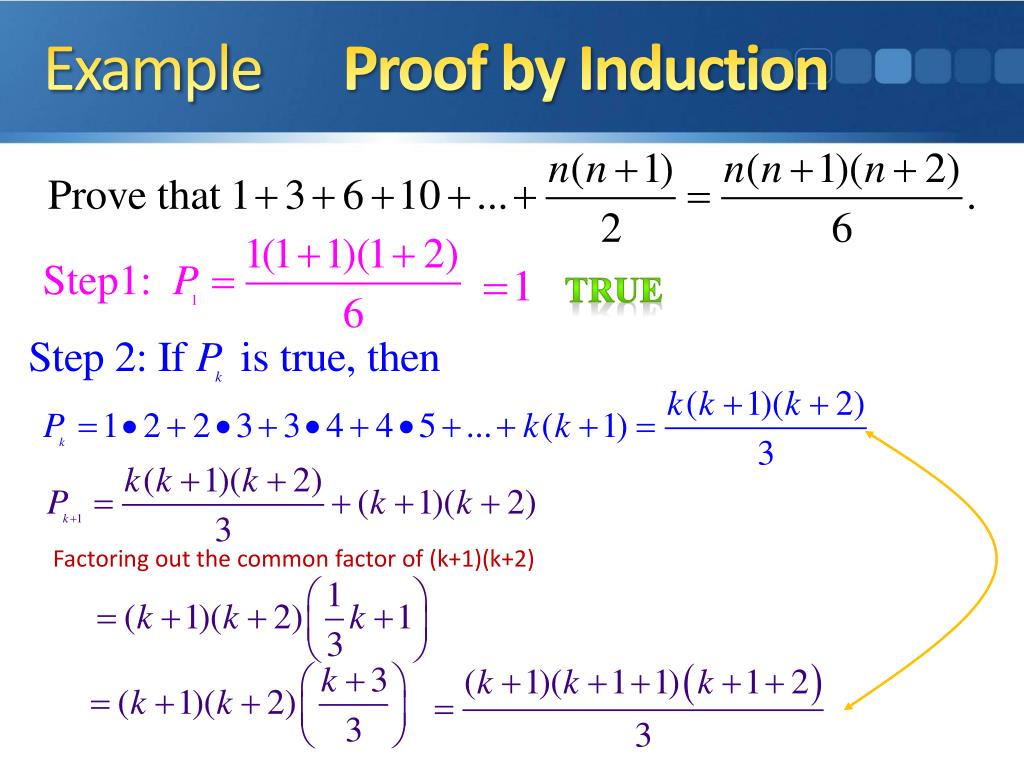
Mathematical Induction Proof Hot Sex Picture
