
Homework And Exercises Problem 12 65 Griffiths Electrodynamics 4 Ed Problem from introduction to electrodynamics, 4th edition, by david j. griffiths, pearson education, inc. A thick spherical shell (inner radius a and outer radius b ) is made of dielectric material with the polarization vec ( p ) ( v e c ( r ) ) = k r hat ( v e c ( r ) ) where k is a constant and r is the distance from the center.
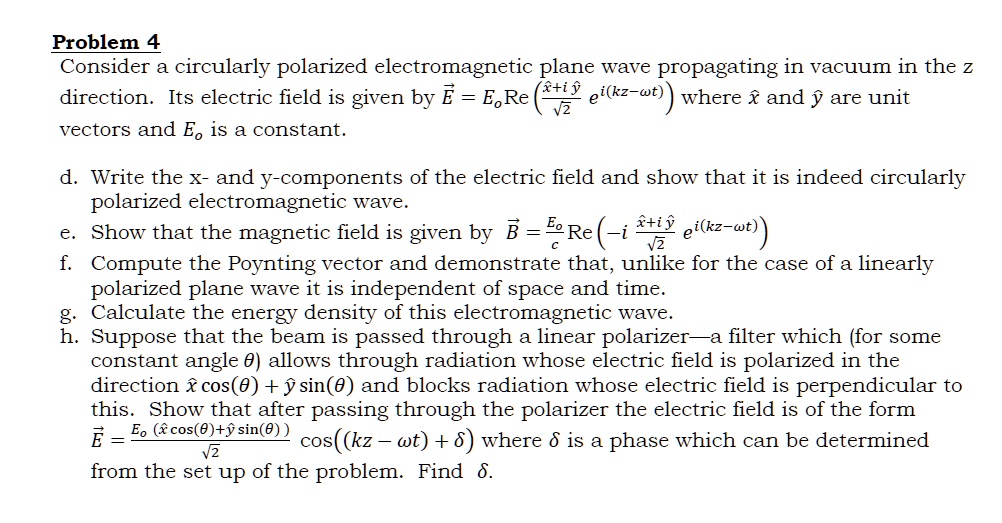
Solved Problem 4 Consider Circularly Polarized Electromagnetic Plane Pr. 4.15 griffiths. a thick spherical shell is made of dielectric material with a frozen in polarization. where k is a constant and r is the distance from the center. there is no free charge. a. b. 66. find e in three regions by two methods. locate all the bound charges ; and use gauss law. ii) find d and then get e from it. a. b. 67 sol. i) 68. Problem 4.15: " a thick spherical shell (inner radius a, outer radius b) is made of dielectric material with a "frozen in" polarization p(r)=k r in r direction, where k is a constant and r is the distance from the center. there is no free charge in the problem. find the electric field in all three regions by two different methods: a) locate all. Figure 2: electric field lines for problem 3(b). the polarization is uniform and vertical between the bound charges (red and blue blobs). c) griffiths eq. (4.18) is e~ in = −p ~ (3 0). it gives the average field inside a sphere due to all the charges within that sphere, assuming that the sphere. Although we can work out the field due to a uniformly polarized sphere using the techniques in the last post, it is also possible to do this using this integral directly. for a uniformly polarized sphere, p(r0) is constant over the volume of the sphere, so we can take it outside the integral. v(r)= 1 4ˇ 0 p z 1 jr r0j2 (r r0) jr r0j d3r0 (2).

Solved Griffiths Electrodynamics 4 E Chapter Two Problems Chegg Figure 2: electric field lines for problem 3(b). the polarization is uniform and vertical between the bound charges (red and blue blobs). c) griffiths eq. (4.18) is e~ in = −p ~ (3 0). it gives the average field inside a sphere due to all the charges within that sphere, assuming that the sphere. Although we can work out the field due to a uniformly polarized sphere using the techniques in the last post, it is also possible to do this using this integral directly. for a uniformly polarized sphere, p(r0) is constant over the volume of the sphere, so we can take it outside the integral. v(r)= 1 4ˇ 0 p z 1 jr r0j2 (r r0) jr r0j d3r0 (2). [1.] problem 4.11 from griffiths a cylinder of length l and radius a has a permanent polarization parallel to its axis. find the bound charge. sketch the electric field for the following cases: (i) l ˛ a, (ii) l ˝ a, and (iii) l ≈ a. figure 1: diagram of problem 4.11 bound charge there are two types of bound charge, surface and volume. The field of a polarized object: ex. 4.2 (griffiths, 3rd ed. ): find the electric field of a sphere of radius 𝑅𝑅, if it is uniformly polarized 𝐏𝐏𝐫𝐫′ = 𝑃𝑃 𝐳𝐳 . 𝜌𝜌𝑏𝑏= −𝛁𝛁′⋅𝐏𝐏𝐫𝐫′ = 0 𝜎𝜎𝑏𝑏= 𝐏𝐏𝐫𝐫′ ⋅𝐧𝐧 ′. Deduce the fields of a hollow shell (and also of a “solid” sphere) of radius uniform surface (or volume) polarization density, either electric or magnetic. A thick spherical shell (inner radius a, outer radius b) is made of dielectric material with a "frozen in" polarization. p (r) = k r r ^ where a constant and is the distance from the center (fig. 4.18). (there is no free charge in the problem.) find the electric field in all three regions by two different methods: figure 4.18.

Solved The Electric Field Of An Elliptically Polarized Plane Chegg [1.] problem 4.11 from griffiths a cylinder of length l and radius a has a permanent polarization parallel to its axis. find the bound charge. sketch the electric field for the following cases: (i) l ˛ a, (ii) l ˝ a, and (iii) l ≈ a. figure 1: diagram of problem 4.11 bound charge there are two types of bound charge, surface and volume. The field of a polarized object: ex. 4.2 (griffiths, 3rd ed. ): find the electric field of a sphere of radius 𝑅𝑅, if it is uniformly polarized 𝐏𝐏𝐫𝐫′ = 𝑃𝑃 𝐳𝐳 . 𝜌𝜌𝑏𝑏= −𝛁𝛁′⋅𝐏𝐏𝐫𝐫′ = 0 𝜎𝜎𝑏𝑏= 𝐏𝐏𝐫𝐫′ ⋅𝐧𝐧 ′. Deduce the fields of a hollow shell (and also of a “solid” sphere) of radius uniform surface (or volume) polarization density, either electric or magnetic. A thick spherical shell (inner radius a, outer radius b) is made of dielectric material with a "frozen in" polarization. p (r) = k r r ^ where a constant and is the distance from the center (fig. 4.18). (there is no free charge in the problem.) find the electric field in all three regions by two different methods: figure 4.18.

Solved 7 14 The Electric Field Of An Elliptically Polarized Chegg Deduce the fields of a hollow shell (and also of a “solid” sphere) of radius uniform surface (or volume) polarization density, either electric or magnetic. A thick spherical shell (inner radius a, outer radius b) is made of dielectric material with a "frozen in" polarization. p (r) = k r r ^ where a constant and is the distance from the center (fig. 4.18). (there is no free charge in the problem.) find the electric field in all three regions by two different methods: figure 4.18.
