Horrible Limit With Factorials Need To Use Stirling Formula In mathematics, stirling's approximation (or stirling's formula) is an asymptotic approximation for factorials. it is a good approximation, leading to accurate results even for small values of n {\displaystyle n} . Use stirling's approximation to evaluate the probability $\lim {n\to \infty}\binom{2n}{n}\left(\frac{1}{4}\right)^n$.

Factorials Stirling S Formula We are aware of calculating factorials using loops or recursion, but if we are asked to calculate factorial without using any loop or recursion. yes, this is possible through a well known approximation algorithm known as stirling approximation. examples: input : n = 6 output : 720. input : n = 2 output : 2. Stirling's approximation gives an approximate value for the factorial function n! or the gamma function gamma(n) for n>>1. the approximation can most simply be derived for n an integer by approximating the sum over the terms of the factorial with an integral, so that lnn! = ln1 ln2 lnn (1) = sum (k=1)^(n)lnk (2) approx int 1^nlnxdx (3. In this supplement, we prove stirling’s approximation to the factorial. it is a remarkably righteous piece of analysis. recall that n! = n×(n−1)×(n−2)×···× 2×1. this is well approximated by s(n) = √ 2π √ nnn e−n = √ 2πnn (1 2) e−n. stirling’s approximation is often stated as n! ∼ s(n),. Stirling formula or stirling approximation, named after scottish mathematician james stirling, is a formula used to find the approximate value of large factorials (written n!; eg; 3! = 3 x 2 x 1) that make use of mathematical constant e (the base of the natural logarithm) and π.

Solved 4 20pt Stirling S Formula Which Gives Chegg In this supplement, we prove stirling’s approximation to the factorial. it is a remarkably righteous piece of analysis. recall that n! = n×(n−1)×(n−2)×···× 2×1. this is well approximated by s(n) = √ 2π √ nnn e−n = √ 2πnn (1 2) e−n. stirling’s approximation is often stated as n! ∼ s(n),. Stirling formula or stirling approximation, named after scottish mathematician james stirling, is a formula used to find the approximate value of large factorials (written n!; eg; 3! = 3 x 2 x 1) that make use of mathematical constant e (the base of the natural logarithm) and π. The large factorials are approximated through the use of stirling’s formula: n! ≃ √ 2π nn 1 2e−n. the proof of the stirling’s formula can be found in many texts, such as [1], [2], and [3]. in this short note stirling’s formula is derived as an application of the central limit theorem. thus, this proof can be introduced in a. Stirling’s approximation, in combination with laplace’s method used in this article can be used to craft a general methods for proving special cases of the central limit theorem. we will take up this fun exercise in another post. Stirling’s formula provides an approximation to n! which is relatively easy to compute and is sufficient for most purposes. using it, one can evaluate log n! to better and better accuracy as n becomes large, provided that one can evaluate log n as accurately as needed.

Solved 4 20pt Stirling S Formula Which Gives Chegg The large factorials are approximated through the use of stirling’s formula: n! ≃ √ 2π nn 1 2e−n. the proof of the stirling’s formula can be found in many texts, such as [1], [2], and [3]. in this short note stirling’s formula is derived as an application of the central limit theorem. thus, this proof can be introduced in a. Stirling’s approximation, in combination with laplace’s method used in this article can be used to craft a general methods for proving special cases of the central limit theorem. we will take up this fun exercise in another post. Stirling’s formula provides an approximation to n! which is relatively easy to compute and is sufficient for most purposes. using it, one can evaluate log n! to better and better accuracy as n becomes large, provided that one can evaluate log n as accurately as needed.

Solved 40 Stirling S Approximation For Large Factorials Is Chegg Stirling’s formula provides an approximation to n! which is relatively easy to compute and is sufficient for most purposes. using it, one can evaluate log n! to better and better accuracy as n becomes large, provided that one can evaluate log n as accurately as needed.
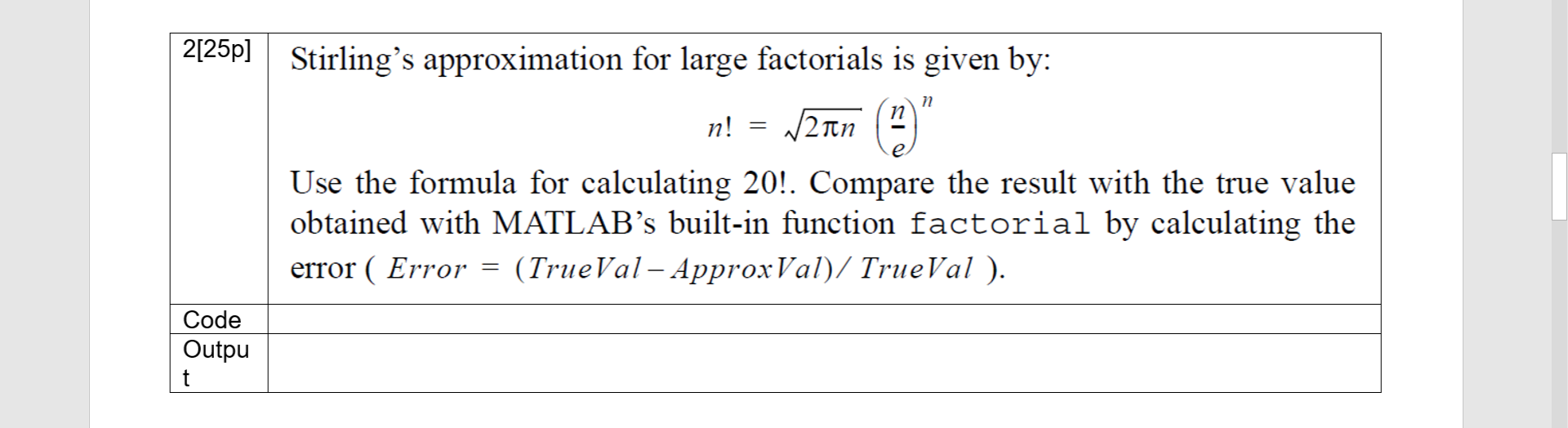
Solved N 2 25 Stirling S Approximation For Large Factorials Chegg
