
Ixl Find The Sum Of An Arithmetic Series Algebra 2 Practice Learn the general form of the arithmetic series formula and the difference between an arithmetic sequence and an arithmetic series. discover the partial sum notation and how to use it to calculate the sum of n terms. This algebra video tutorial explains how to find the sum of an arithmetic series using 2 formulas. sequences free formula sheet: bit.ly 4eau2kq more. sequences and.

Find The Sum Of The Arithmetic Series Sum K 1 12 Cameramath Given a geometric series, whose first term is a a and with a constant ratio of r r ∑n k=1 a ∗rk−1, ∑ k = 1 n a ∗ r k − 1, we can write out the terms of the series in a similar way that we did for the arithmetic series. so for a finite geometric series, we can use this formula to find the sum. To sum up the terms of this arithmetic sequence: a (a d) (a 2d) (a 3d) use this formula: what is that funny symbol? it is called sigma notation. Σ (called sigma) means "sum up" and below and above it are shown the starting and ending values: it says "sum up n where n goes from 1 to 4. answer= 10. here is how to use it:. We can use the first formula to find sum of n terms, if we don't have the last term of the given arithmetic series. we can use the second formula to find sum of n terms, if we have the last term of the given arithmetic series. problem 1 : find the sum of first 75 positive integers. solution : 1 2 3 75. There are two common formulas for calculating this sum: the sum (s) of an arithmetic series can be calculated using the following formula: $$s = \frac {n} {2} (a l)$$s = 2n(a l) where: alternatively, you can calculate the sum using the first term (a) and the common difference (d): $$s = \frac {n} {2} [2a (n 1)d]$$s = 2n[2a (n−1)d].

Sum Of Arithmetic Series We can use the first formula to find sum of n terms, if we don't have the last term of the given arithmetic series. we can use the second formula to find sum of n terms, if we have the last term of the given arithmetic series. problem 1 : find the sum of first 75 positive integers. solution : 1 2 3 75. There are two common formulas for calculating this sum: the sum (s) of an arithmetic series can be calculated using the following formula: $$s = \frac {n} {2} (a l)$$s = 2n(a l) where: alternatively, you can calculate the sum using the first term (a) and the common difference (d): $$s = \frac {n} {2} [2a (n 1)d]$$s = 2n[2a (n−1)d]. Learn how to find the sum of arithmetic series. derive a formula for the sum. use summation notation. The sum of the first n terms of a series is called "the n th partial sum", and is often denoted as "s n ". find the 35 th partial sum, s 35 , of the arithmetic sequence with terms a n = (1 2) n 1. Identify whether the given sum is an arithmetic series or not. for a finite arithmetic sequence with n terms and general formula a n=a 1 (n−1)d, where a 1 is the first term and d the common difference, the sum of all terms s n can be calculated using the following formula. How to: given terms of an arithmetic series, find the sum of the first [latex]n[ latex] terms. identify [latex]{a} {1}[ latex] and [latex]{a} {n}[ latex]. determine [latex]n[ latex].
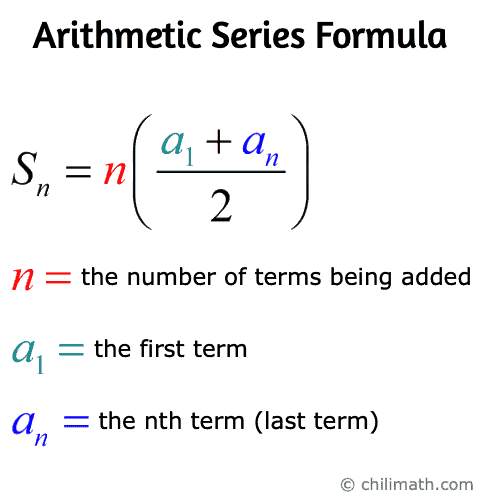
Arithmetic Series Formula Chilimath Learn how to find the sum of arithmetic series. derive a formula for the sum. use summation notation. The sum of the first n terms of a series is called "the n th partial sum", and is often denoted as "s n ". find the 35 th partial sum, s 35 , of the arithmetic sequence with terms a n = (1 2) n 1. Identify whether the given sum is an arithmetic series or not. for a finite arithmetic sequence with n terms and general formula a n=a 1 (n−1)d, where a 1 is the first term and d the common difference, the sum of all terms s n can be calculated using the following formula. How to: given terms of an arithmetic series, find the sum of the first [latex]n[ latex] terms. identify [latex]{a} {1}[ latex] and [latex]{a} {n}[ latex]. determine [latex]n[ latex].
