
Solved Find A Basis For The Following Spaces A A Line Chegg If s = fv1; : : : ; vng is a basis for a vector space v and t = fw1; : : : ; wmg is a linearly independent set of vectors in v , then m n. proof. the idea is to start with the set s and replace vectors in s one at a time with vectors from t , such that after each replacement we still have a basis for v . In this video we show that if s and t are bases for a vector space v, then s and t have the same number of vectors in them. watch and learn!here is the link.

Solved 4 Given A Set S Of Vectors In A Vector Space V Chegg So, we have two sets: $s 1 = \left\lbrace v 1, v 2, , v n \right\rbrace$ and $s 2 = \left\lbrace w 1, w 2, , w m \right\rbrace$ in which we claim that $m \neq n$ and both are bases for the vector space. first, consider set $s 1$ to be the basis for the vector space and let $s 2$ be a set of m linearly independent vectors (since we claim. Suppose v is a vector space. if v has a basis with n elements then all bases have n elements. proof.suppose s = {v 1,v 2, ,v n} and t = {u 1,u 2, ,u m} are two bases of v. since, the basiss has n elements, and t is linealry independent, by the thoerem above m cannot be bigger than n. so, m ≤ n. by switching the roles of s and t, we have n. Let s be a subset of a vector space v. definition. the span of the set s is the smallest subspace w ⊂ v that contains s. if s is not empty then w = span(s) consists of all linear combinations r1v1 r2v2 ··· rkvk such that v1, ,vk ∈ s and r1, ,rk ∈ r. we say that the set s spans the subspace w or that s is a spanning set for w. Proof. 1. let s be a basis of a vector space v. then by the definition of bases v=span(s), so every vector in v is equal to a linear combination of vectors from s. it remains to prove that this linear combination is unique. by contradiction, suppose that there exists a vector a in v which is equal to two different linear combinations of vectors.
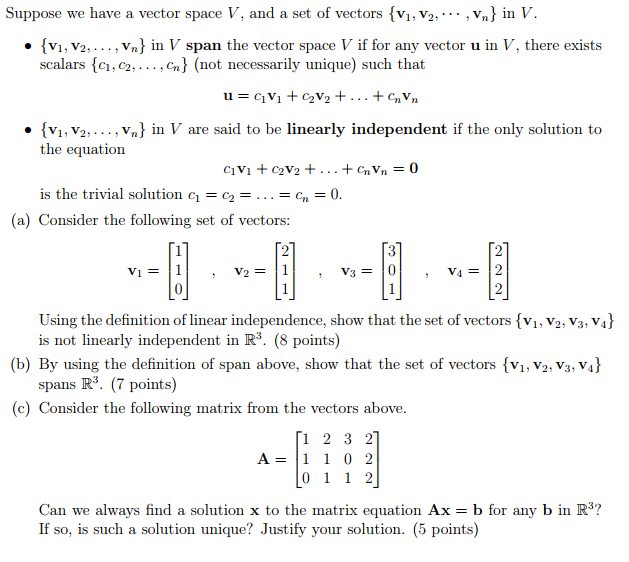
Solved Suppose We Have A Vector Space V And A Set Of Chegg Let s be a subset of a vector space v. definition. the span of the set s is the smallest subspace w ⊂ v that contains s. if s is not empty then w = span(s) consists of all linear combinations r1v1 r2v2 ··· rkvk such that v1, ,vk ∈ s and r1, ,rk ∈ r. we say that the set s spans the subspace w or that s is a spanning set for w. Proof. 1. let s be a basis of a vector space v. then by the definition of bases v=span(s), so every vector in v is equal to a linear combination of vectors from s. it remains to prove that this linear combination is unique. by contradiction, suppose that there exists a vector a in v which is equal to two different linear combinations of vectors. De nition 4.2. a collection of vectors in v which is both linearly inde pendent and spans v is called a basis of v. notice that we have not required that a basis be a nite set. usually, however, we will deal with vector spaces that have a nite basis. one of the questions we will investigate is whether a nite dimensional vector space has a basis. A set of vectors s = {# v: 1, ··· , ⃗v: n} is a basis if s spans v and is linearly independent. equivalently, each ⃗v ∈ v can be written uniquely as ⃗v = a: 1: ⃗v: 1 ··· a: n: ⃗v: n, where the a: i: are called the coordinates of ⃗v in the basis s. » the standard basis for r. 2: is 1 0, . 0 1 in general, when we write a. If s = {v 1, v 2, , v n} is a basis for a vector space v and t = {w 1, w 2, , w k} is a linearly independent set of vectors in v, then k < n. remark: if s and t are both bases for v then k = n. this says that every basis has the same number of vectors. hence the dimension is will defined. Theorem 2 if a vector space v has a finite basis, then all bases for v are finite and have the same number of elements. definition. the dimension of a vector space v , denoted dim v , is the number of elements in any of its bases. examples. problem. x 2z = 0 in r3. z) = (−2s, t, s) = t(0, 1, 0) s(−2, 0, 1).

Solved 11 Prove That If V Is A Vector Space Generated De nition 4.2. a collection of vectors in v which is both linearly inde pendent and spans v is called a basis of v. notice that we have not required that a basis be a nite set. usually, however, we will deal with vector spaces that have a nite basis. one of the questions we will investigate is whether a nite dimensional vector space has a basis. A set of vectors s = {# v: 1, ··· , ⃗v: n} is a basis if s spans v and is linearly independent. equivalently, each ⃗v ∈ v can be written uniquely as ⃗v = a: 1: ⃗v: 1 ··· a: n: ⃗v: n, where the a: i: are called the coordinates of ⃗v in the basis s. » the standard basis for r. 2: is 1 0, . 0 1 in general, when we write a. If s = {v 1, v 2, , v n} is a basis for a vector space v and t = {w 1, w 2, , w k} is a linearly independent set of vectors in v, then k < n. remark: if s and t are both bases for v then k = n. this says that every basis has the same number of vectors. hence the dimension is will defined. Theorem 2 if a vector space v has a finite basis, then all bases for v are finite and have the same number of elements. definition. the dimension of a vector space v , denoted dim v , is the number of elements in any of its bases. examples. problem. x 2z = 0 in r3. z) = (−2s, t, s) = t(0, 1, 0) s(−2, 0, 1).

Solved A Vector Space V And A Subset S Are Given Determine Chegg If s = {v 1, v 2, , v n} is a basis for a vector space v and t = {w 1, w 2, , w k} is a linearly independent set of vectors in v, then k < n. remark: if s and t are both bases for v then k = n. this says that every basis has the same number of vectors. hence the dimension is will defined. Theorem 2 if a vector space v has a finite basis, then all bases for v are finite and have the same number of elements. definition. the dimension of a vector space v , denoted dim v , is the number of elements in any of its bases. examples. problem. x 2z = 0 in r3. z) = (−2s, t, s) = t(0, 1, 0) s(−2, 0, 1).

Solved Question 5 If A Set Of Vectors In A Vector Space V Is Chegg
