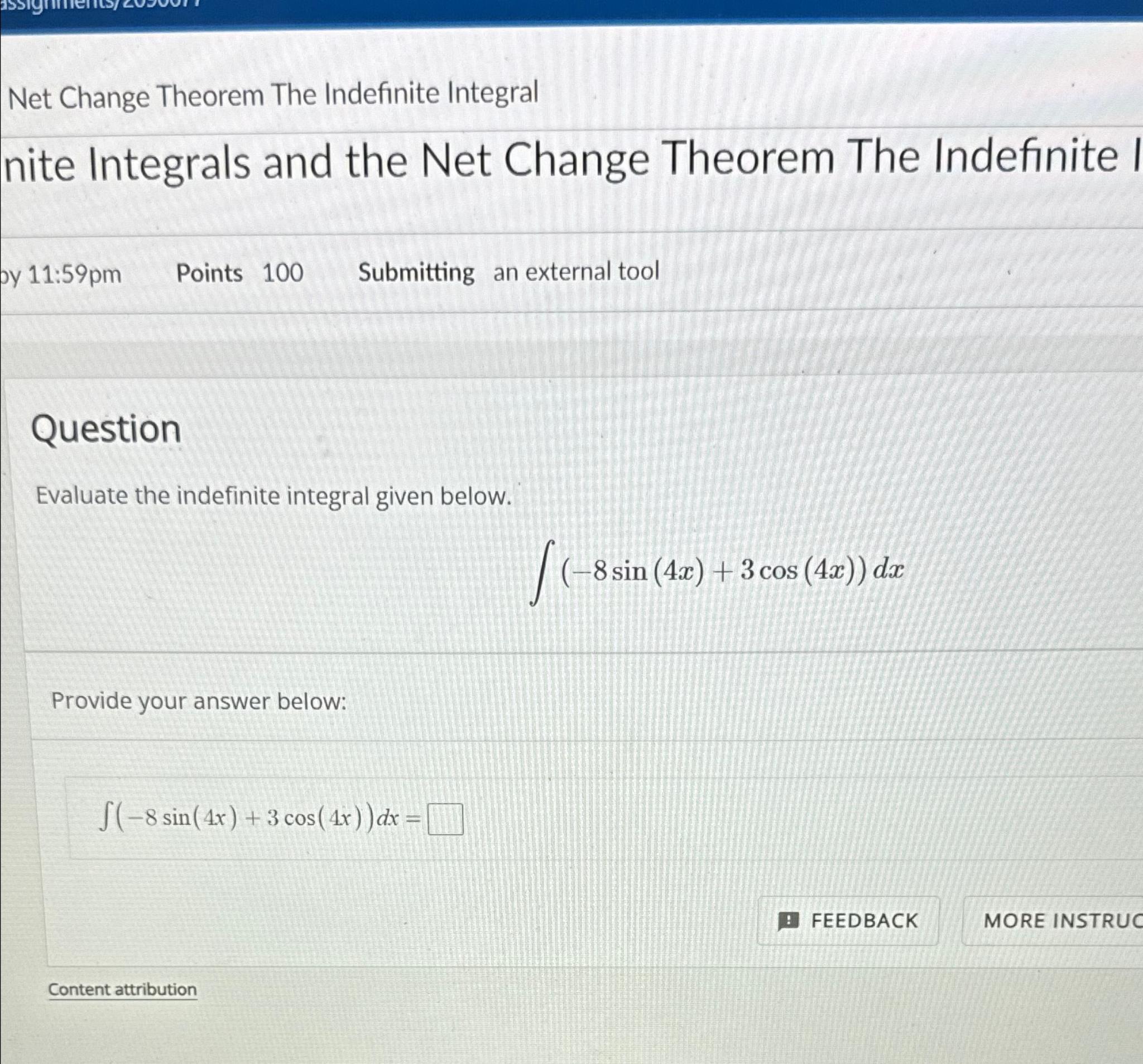
Solved Net Change Theorem The Indefinite Integralnite Chegg The net change theorem states that when a quantity changes, the final value equals the initial value plus the integral of the rate of change. net change can be a positive number, a negative number, or zero. Use the power rule to integrate the function ∫4 1 t√ (1 t) dt. ∫ 1 4 t (1 t) d t. find the definite integral of f(x) = x2 − 3x f (x) = x 2 − 3 x over the interval [1, 3]. [1, 3]. the net change theorem considers the integral of a rate of change.

Indefinite Integrals And The Net Change Theorem Pdf Integral Velocity The net change in the value of f over an interval [a,b] is the difference f(b) f(a). theorem (net change theorem) . the integral of the rate of change of a function is the net change in that. A rock that was thrown upward has a velocity function of v(t) t? 64 8 meters per second. find (a) the total displacement of the rock over the first five seconds it travels (b) the total distance traveled the rock over the first five seconds oy f uh chep db. 6h 4 hf ip fae at oo ( tet g20 u4)? 4 ww. The net change theorem, which is a restatement of the ftc ii from a different perspective, says that the net change in a function is the definite integral of its derivative. $$\int a^b f'(x)\, dx = f(b) f(a)$$. Net change theorem. part 2 of the fundamental theorem says that if f is continuous on [a,b], then zb a f(x)dx = f(b)−f(a) where f is any antiderivative of f. this means that f′ = f, so the equation can be rewritten as zb a f′(x)dx = f(b) −f(a).
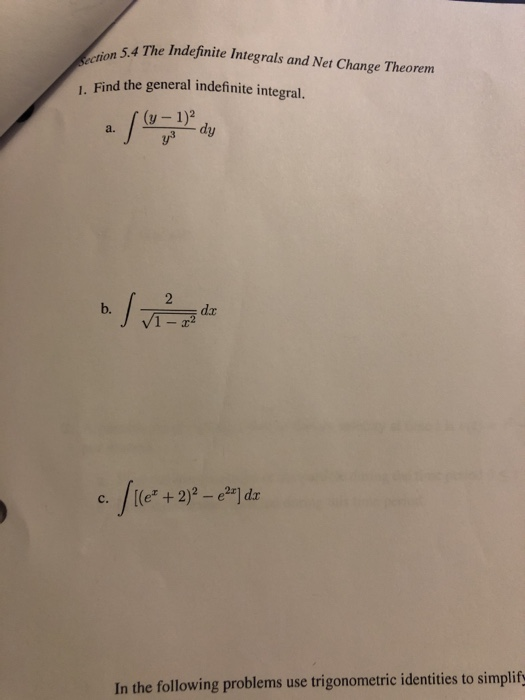
Solved 5 4 The Indefinite Integrals And Net Change Theorem Chegg The net change theorem, which is a restatement of the ftc ii from a different perspective, says that the net change in a function is the definite integral of its derivative. $$\int a^b f'(x)\, dx = f(b) f(a)$$. Net change theorem. part 2 of the fundamental theorem says that if f is continuous on [a,b], then zb a f(x)dx = f(b)−f(a) where f is any antiderivative of f. this means that f′ = f, so the equation can be rewritten as zb a f′(x)dx = f(b) −f(a). Integral of f′(x) from a to b is equal to the net change of f(x) over [a,b]. more precisely, the integral of a rate of change is the net change i.e. z b a f′(x)dx = f(b)− f(a). this can be interpreted in many different ways depending upon what f(x) is measuring. for example, if v(t) is the velocity of a moving object, then since s′(t. This says that the definite integral of the rate of change of a quantity gives the net change in the quantity. the text calls this fact the net change theorem. for \(f(t)\) a velocity, displacement is net change. Indefinite integrals and the net change theorem what is an indefinite integral? an indefinite integral of a function f is an anti derivative of f, and is denoted r f(x)dx. that is: z f(x)dx = f(x) means f0(x) = f(x) convention #1: we always write all anti derivatives. e.g. z xdx = 1 2 x2 c convention #2: the formula for an anti derivative. The definite integral of the rate of change of f equals the net change in f: ∫ ( ) ex 1: suppose the population of a country grows at a constant rate of 50,000 people per year.
