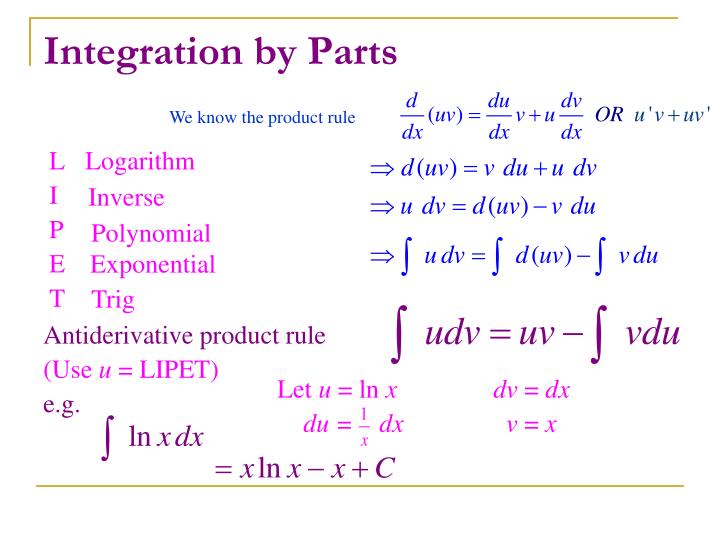
Integration By Parts Learn how to use integration by parts to evaluate integrals of the form ∫ udv = uv −∫ vdu. see the derivation of the formula, the steps to choose u and v, and examples with solutions. Learn how to find the integral of a product of functions using integration by parts, a technique derived from the product rule of differentiation. see the formula, examples, applications, and generalizations for various types of integrals.

Integration By Parts Pdf Learn how to use integration by parts, a technique based on the product rule for differentiation, to solve integrals involving two functions. see examples, formulas, exercises and analysis of the method. Integration by parts or partial integration, is a technique used in calculus to evaluate the integral of a product of two functions. the formula for partial integration is given by: ∫ u dv = uv – ∫ v du. where u and v are differentiable functions of x. Learn how to use integration by parts to perform indefinite or definite integration of products of functions. see examples, formulas, references and related topics. Learn how to use the integration by parts formula to evaluate integrals of the form ˆ f(x)g(x) dx, where f(x) and g(x) are differentiable functions. see examples, tips, tricks and practice problems with solutions.

Integration By Parts Learn how to use integration by parts to perform indefinite or definite integration of products of functions. see examples, formulas, references and related topics. Learn how to use the integration by parts formula to evaluate integrals of the form ˆ f(x)g(x) dx, where f(x) and g(x) are differentiable functions. see examples, tips, tricks and practice problems with solutions. Learn how to integrate products of two functions by parts using the formula, ilate rule and examples. find out how to apply integration by parts with limits and solve problems involving trigonometric, logarithmic and exponential functions. Integration by parts is the technique used to find the integral of the product of two types of functions. the popular integration by parts formula is, ∫ u dv = uv ∫ v du. learn more about the derivation, applications, and examples of integration by parts formula. In this comprehensive tutorial, we explore two powerful methods in calculus: integration by parts and bernoulli's rule. first, we dive into the technique of.

Integration By Parts Learn how to integrate products of two functions by parts using the formula, ilate rule and examples. find out how to apply integration by parts with limits and solve problems involving trigonometric, logarithmic and exponential functions. Integration by parts is the technique used to find the integral of the product of two types of functions. the popular integration by parts formula is, ∫ u dv = uv ∫ v du. learn more about the derivation, applications, and examples of integration by parts formula. In this comprehensive tutorial, we explore two powerful methods in calculus: integration by parts and bernoulli's rule. first, we dive into the technique of.

Integration By Parts Mr Mathematics In this comprehensive tutorial, we explore two powerful methods in calculus: integration by parts and bernoulli's rule. first, we dive into the technique of.
Integration By Parts
