
Infinite Series Tests Review Pdf Infinity Summation The study of series and convergence is a fundamental and elegant branch of mathematics. in this course, we will explore the beauty and power of infinite sums, discovering their applications and. Infinite sequences and series tests for convergence and divergence – a summary theorems on algebraic operations on series: let ∑an and ∑bn be any two series. 1. if ∑an and ∑bn both converge, then ∑(an ±bn) must converge. 2. if ∑an converges, and c is a real number, then ∑can must converge.

Solved Infinite Series Tests For Convergence Of Series Chegg Math 480: introductory mathematical analysisconvergence tests for infinite seriesnovember 27, 2018this is a lecture on "convergence tests for infinite series. To prove convergence, the comparison series must converge and be a larger series. if the series has a form similar to that of a p series or geometric series. in particular, if is a rational function or is algebraic (features roots of polynomials), then the series should be compared to a p series. In this section we give a general set of guidelines for determining which test to use in determining if an infinite series will converge or diverge. note as well that there really isn’t one set of guidelines that will always work and so you always need to be flexible in following this set of guidelines. The root test for convergence. given a series ∑ a n of positive terms (that is, a n > 0) such that the root has a limit r as n → ∞, • if r < 1, then ∑ a n converges • if r > 1, then ∑ a n diverges • if r = 1, then ∑ a n could converge or diverge. (this test works since tells us that the series is comparable to a geometric.

Solved Topic Infinite Series Convergence And Divergence Chegg In this section we give a general set of guidelines for determining which test to use in determining if an infinite series will converge or diverge. note as well that there really isn’t one set of guidelines that will always work and so you always need to be flexible in following this set of guidelines. The root test for convergence. given a series ∑ a n of positive terms (that is, a n > 0) such that the root has a limit r as n → ∞, • if r < 1, then ∑ a n converges • if r > 1, then ∑ a n diverges • if r = 1, then ∑ a n could converge or diverge. (this test works since tells us that the series is comparable to a geometric. In this tutorial, we review some of the most common tests for the convergence of an infinite series $$ \sum {k=0}^{\infty} a k = a 0 a 1 a 2 \cdots $$ the proofs or these tests are interesting, so we urge you to look them up in your calculus text. If is positive and decreasing, then if 矫꾡 nn the integral tt →∞ lim diverges, ∫ 1 tt ff ( xx)dd xx < 0, then let the series converges. ff ( 灚筢) = 矫꾡 nn . if the series diverges as well. let 摩ネ> 0 . it then∑ converges if . if n and k = 1, ∞ is the nn= harmonic kk nn1 pp series, which ۾껡> diverges. 1. Description: we continue studying the convergence of infinite series, defining absolute convergence and proving the comparison test: the test of all tests. we also prove properties of p series. speaker: casey rodriguez. Ans. some common convergence tests used to determine the convergence of an infinite series are the ratio test, the root test, the integral test, the comparison test, and the alternating series test. these tests help analyze the behavior of the series and determine if it converges or diverges.

Solution Tests For The Convergence Of An Infinite Series Studypool In this tutorial, we review some of the most common tests for the convergence of an infinite series $$ \sum {k=0}^{\infty} a k = a 0 a 1 a 2 \cdots $$ the proofs or these tests are interesting, so we urge you to look them up in your calculus text. If is positive and decreasing, then if 矫꾡 nn the integral tt →∞ lim diverges, ∫ 1 tt ff ( xx)dd xx < 0, then let the series converges. ff ( 灚筢) = 矫꾡 nn . if the series diverges as well. let 摩ネ> 0 . it then∑ converges if . if n and k = 1, ∞ is the nn= harmonic kk nn1 pp series, which ۾껡> diverges. 1. Description: we continue studying the convergence of infinite series, defining absolute convergence and proving the comparison test: the test of all tests. we also prove properties of p series. speaker: casey rodriguez. Ans. some common convergence tests used to determine the convergence of an infinite series are the ratio test, the root test, the integral test, the comparison test, and the alternating series test. these tests help analyze the behavior of the series and determine if it converges or diverges.
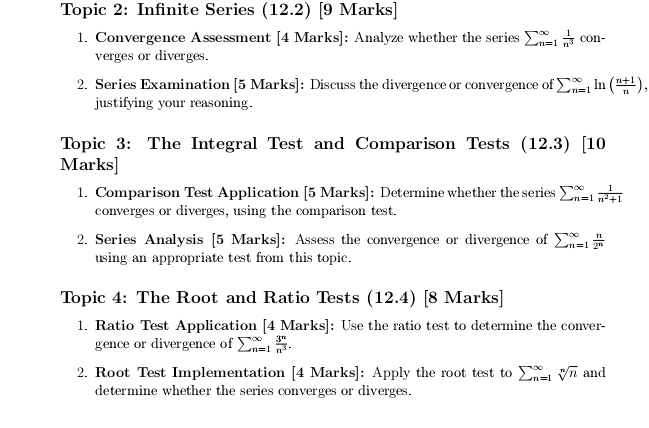
Solved Topic 2 Infinite Series 12 2 9 ï Marks Convergence Chegg Description: we continue studying the convergence of infinite series, defining absolute convergence and proving the comparison test: the test of all tests. we also prove properties of p series. speaker: casey rodriguez. Ans. some common convergence tests used to determine the convergence of an infinite series are the ratio test, the root test, the integral test, the comparison test, and the alternating series test. these tests help analyze the behavior of the series and determine if it converges or diverges.
