
Irrational Numbers Pdf So what does an irrational number look like? an irrational number is a nonterminating, nonrepeating decimal. 2. a few examples of irrational numbers are π , 2 , and 3 . (in fact, the square root of any prime number is irrational. many other square roots are irrational as well.) the values of π , 2 , and 3 are shown below to 50 decimal places. Irrational numbers are numbers that people cannot normally write the number in their standard, fraction, and decimal form. people can easily prove if a number is irrational by trying to write the said number in its standard form and contrasting it to a set of criteria that describes irrational numbers.

Rational And Irrational Numbers Pdf Numbers Rational Number Create a diagram to show how these number systems are related: irrational numbers; rational numbers; integers; whole numbers; and natural numbers. write each number. A number that can not be written as a fraction (ratio) with an integer on top and an integer on the bottom is an irrational number. examples of irrational numbers: • a square root of a number √(x), is irrational if the number x is not a perfect square. √(8), √(10), √(24), and √(40) are all irrational numbers since 8, 10, 24. List of irrational numbers. the famous irrational numbers consist of pi, euler’s number, golden ratio. many square roots and cube roots numbers are also irrational, but not all of them. for example, √3 is an irrational number but √4 is a rational number. because 4 is a perfect square, such as 4 = 2 x 2 and √4 = 2, which is a rational. Arguments for statements involving irrational numbers necessitates the use of the method of proof by contradiction. we shall give a few simple examples of this type of arguments, each concerned with a statement involving irrational.

Irrational Numbers Examples Format Pdf List of irrational numbers. the famous irrational numbers consist of pi, euler’s number, golden ratio. many square roots and cube roots numbers are also irrational, but not all of them. for example, √3 is an irrational number but √4 is a rational number. because 4 is a perfect square, such as 4 = 2 x 2 and √4 = 2, which is a rational. Arguments for statements involving irrational numbers necessitates the use of the method of proof by contradiction. we shall give a few simple examples of this type of arguments, each concerned with a statement involving irrational. Examples of rational numbers are 7=2 and 13=5. any integer is a rational number because m= m=1. a real number is one which can be expressed as decimal expansion; for example 0:0000:::, 1:0000::: and 0:33333:::. you probably know that every number with a periodic decimal expansion is rational. for example, 0:33333:::= 1 irrational numbers. Learning target: understand the concept of irrational numbers. success criteria: • i can classify real numbers as rational or irrational. • i can approximate irrational numbers. Decide whether the following numbers, sums or products yield a number that is rational or irrational. simplify if necessary and explain for each problem why it is a rational or irrational number. 1. We want in this article to find out what departures, if any, are present for irrational numbers and their products. to do so we look at 500 digit long expansions of the constants and exp(1). a measure of the departure from a uniform distribution of integers in these irrationals will be measured by the observed departure from m=4.5.

Irrational Numbers Examples Pdf Examples Examples of rational numbers are 7=2 and 13=5. any integer is a rational number because m= m=1. a real number is one which can be expressed as decimal expansion; for example 0:0000:::, 1:0000::: and 0:33333:::. you probably know that every number with a periodic decimal expansion is rational. for example, 0:33333:::= 1 irrational numbers. Learning target: understand the concept of irrational numbers. success criteria: • i can classify real numbers as rational or irrational. • i can approximate irrational numbers. Decide whether the following numbers, sums or products yield a number that is rational or irrational. simplify if necessary and explain for each problem why it is a rational or irrational number. 1. We want in this article to find out what departures, if any, are present for irrational numbers and their products. to do so we look at 500 digit long expansions of the constants and exp(1). a measure of the departure from a uniform distribution of integers in these irrationals will be measured by the observed departure from m=4.5.
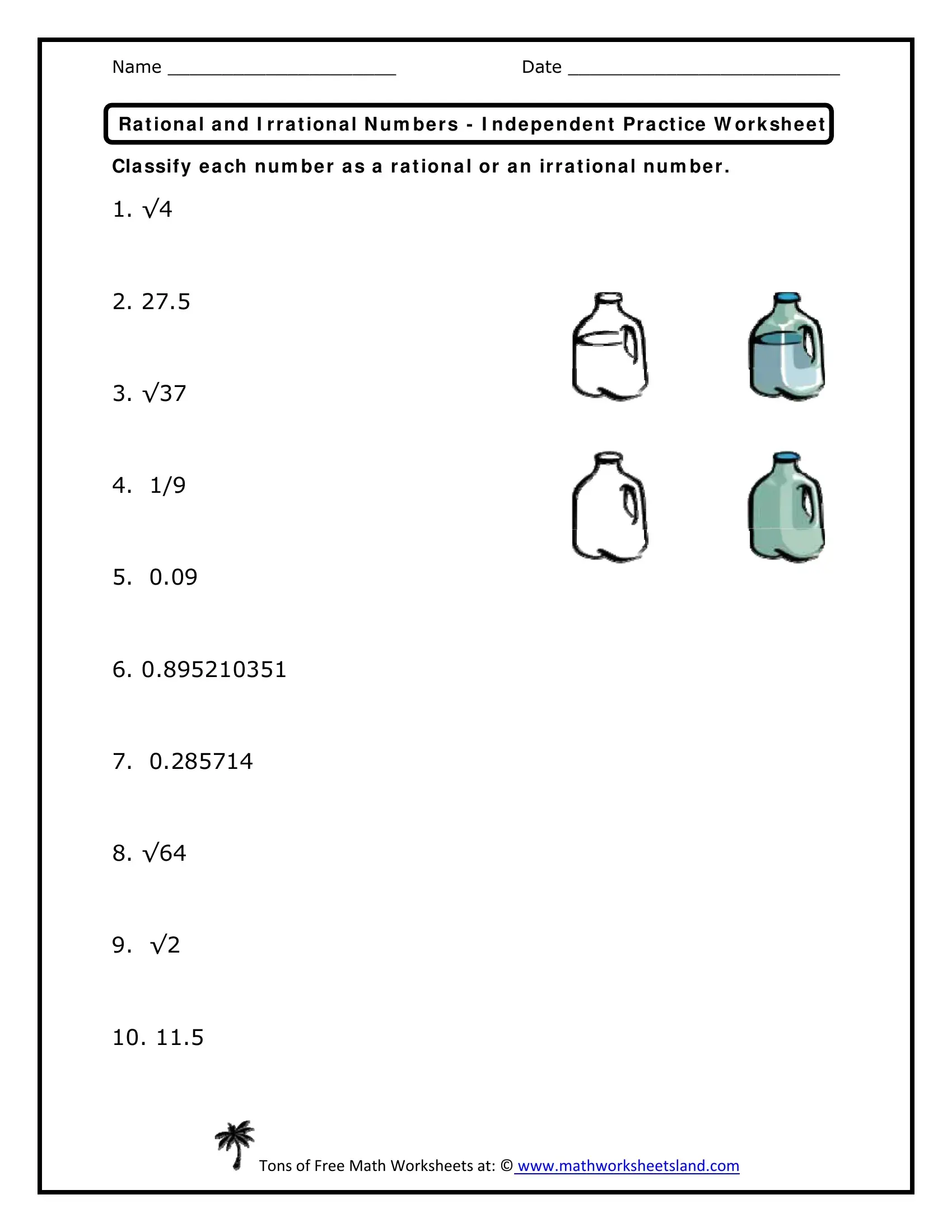
Rational Irrational Numbers Pdf Form Formspal Decide whether the following numbers, sums or products yield a number that is rational or irrational. simplify if necessary and explain for each problem why it is a rational or irrational number. 1. We want in this article to find out what departures, if any, are present for irrational numbers and their products. to do so we look at 500 digit long expansions of the constants and exp(1). a measure of the departure from a uniform distribution of integers in these irrationals will be measured by the observed departure from m=4.5.
Understanding Irrational Numbers Worksheets Helping With Math
