
Irrational Numbers Pdf Can you think of any numbers that are not rational numbers? 1. the technical definition of an irrational number is that it is a “real number which is not a rational number.” so what does an irrational number look like? an irrational number is a nonterminating, nonrepeating decimal. 2. a few examples of irrational numbers are π , 2 , and 3 . Central results on irrational numbers, and is not aimed at providing an exhaustive treatment of the problems with which it deals. the term "irrational numbers," a usage inherited from ancient greece which is not too felicitous in view of the everyday meaning of the word "irrational," is employed in the title in a generic sense to include such.

Rational And Irrational Numbers Presentation In Beige Handdrawn Style Irrational numbers are prevailing on the number line. in fact, if you choose a number randomly from [0,1], the probability that the number is irrational turns out to be 1. this is an interesting result in probability theory. in this session, instead of measure theory, we are going to see the set of irrational numbers. Rational numbers are those which can be represented as a ratio of two integers — i.e., the set {a b: a,b ∈ z, b 6= 0 } — and the irrational numbers are those which cannot be written as the quotient of two integers. we will, in essence, show that the set of irrational numbers is not empty. Lecture 2: irrational numbers we have worked on some irrationality proofs on the blackboard: theorem: p 3 is irrational. proof: p 3 = p=qimplies 3 = p 2=q2 or 3q2 = p. if we make a prime factorization, then on the left hand side contains an odd number of factors 3, while the right hand side contains an even number of factors 3. this is not. Download irrational numbers pdf. 1. rationals and irrationals. 2. simple irrationalities. 3. certain algebraic numbers. 4. the approximation of irrationals by rationals. 5. continued fractions. 6. further diophantine approximations. 7. algebraic and transcendental numbers. 8. normal numbers. 9. the generalized lindemann theorem. 10.
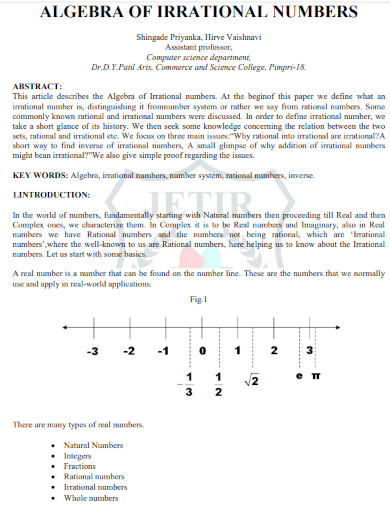
Irrational Numbers Examples Format Pdf Lecture 2: irrational numbers we have worked on some irrationality proofs on the blackboard: theorem: p 3 is irrational. proof: p 3 = p=qimplies 3 = p 2=q2 or 3q2 = p. if we make a prime factorization, then on the left hand side contains an odd number of factors 3, while the right hand side contains an even number of factors 3. this is not. Download irrational numbers pdf. 1. rationals and irrationals. 2. simple irrationalities. 3. certain algebraic numbers. 4. the approximation of irrationals by rationals. 5. continued fractions. 6. further diophantine approximations. 7. algebraic and transcendental numbers. 8. normal numbers. 9. the generalized lindemann theorem. 10. Irrational numbers sequences and series by henry parker manning, pa.d. assistant professor of pure mathematics in brown university first edition first thousand new york john wiley & sons. A number that can not be written as a fraction (ratio) with an integer on top and an integer on the bottom is an irrational number. examples of irrational numbers: • a square root of a number √(x), is irrational if the number x is not a perfect square. √(8), √(10), √(24), and √(40) are all irrational numbers since 8, 10, 24. Any square root that doesn’t come out to a whole number is an irrational number. if a number is either rational or irrational, it’s classified as a real number. there are non real numbers, but most students don’t encounter those until high school. for this class, we will always be working in the real number system. Arguments for statements involving irrational numbers necessitates the use of the method of proof by contradiction. we shall give a few simple examples of this type of arguments, each concerned with a statement involving irrational.
Irrational Numbers In Mathematics I Answer 4 U Irrational numbers sequences and series by henry parker manning, pa.d. assistant professor of pure mathematics in brown university first edition first thousand new york john wiley & sons. A number that can not be written as a fraction (ratio) with an integer on top and an integer on the bottom is an irrational number. examples of irrational numbers: • a square root of a number √(x), is irrational if the number x is not a perfect square. √(8), √(10), √(24), and √(40) are all irrational numbers since 8, 10, 24. Any square root that doesn’t come out to a whole number is an irrational number. if a number is either rational or irrational, it’s classified as a real number. there are non real numbers, but most students don’t encounter those until high school. for this class, we will always be working in the real number system. Arguments for statements involving irrational numbers necessitates the use of the method of proof by contradiction. we shall give a few simple examples of this type of arguments, each concerned with a statement involving irrational.
