Let A 1 2 3 4 5 6 B 2 4 6 8 Find A B And B A

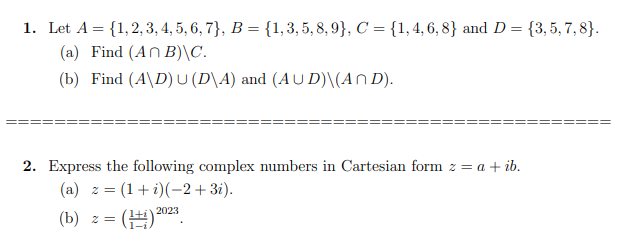
Solved 1 Let A 1 2 3 4 5 6 7 B 1 3 5 8 9 C 1 4 6 8 Chegg Example 18 let a = { 1, 2, 3, 4, 5, 6}, b = { 2, 4, 6, 8 }. find a – b and b – a. a – b = a – (a ∩ b) a ∩ b = {1, 2, 3, 4, 5, 6} ∩ {2, 4, 6, 8} = {2, 4, 6} a – b = a – (a ∩ b) = {1, 2, 3, 4, 5, 6} – {2, 4, 6} = {1, 3, 5} b – a = b – (b ∩ a) = b – (a ∩ b) = {2,. Let a = {1,2,3,4,5,6}, b = {2,4,6,8}. find a−b and b−a. let a = {1,2,3,4} and b ={2,4,6,8}. then find a−b. let a = {1, 2, 3, 4, 5, 6} and b = {2, 4, 6, 8}. then the sets a∪b and a∩b are. if a = {1,2,3,4,5,6} and b = {2,4,6,8}, then a b = ? let a = {2,4,6,8} and b ={6,8,10,12}. find a∪b.

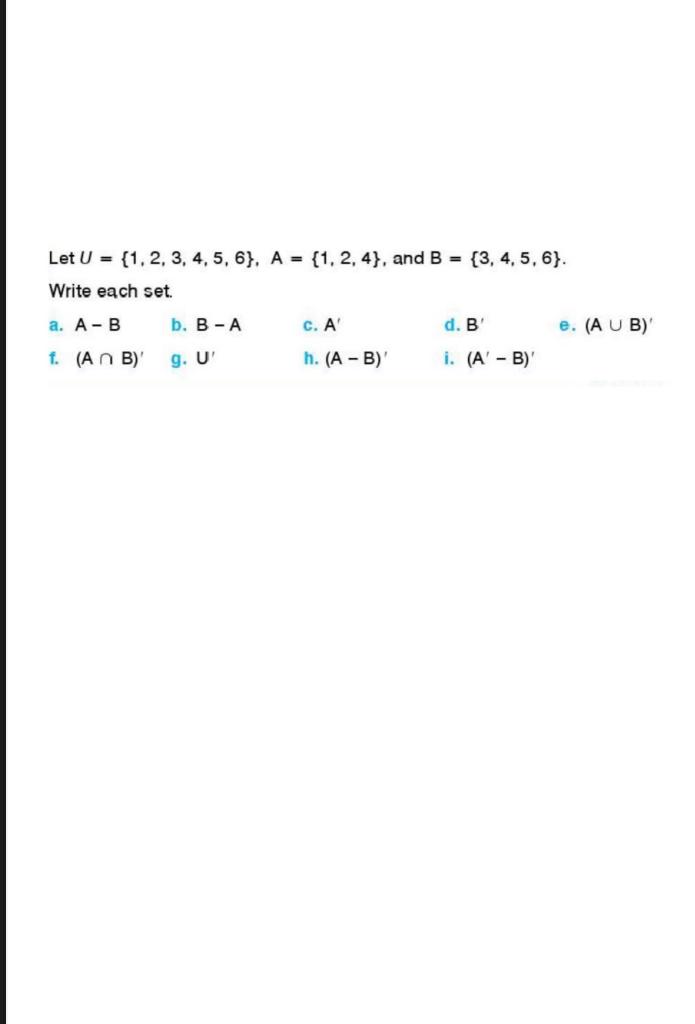
Solved Let U 1 2 3 4 5 6 A 1 2 4 And B 3 4 5 6 Write Chegg Find `a b` and `b a`. Given : let a = {1,2,3,4,5,6} , b = {2,4,6,8}. to find : a b and b a ? solution : the following steps will give you a b or b a, 1. look elements of both set a and b carefully. 2. cancel all the elements which is found similar in both set. 3. after cancellation of similar elements of both sets, write down the uncancelled elements of set a or set b. One to one function: a function f:a → b is one to one if every element of the range b corresponds to exactly one element of the domain a of f. important: for a function f:a → b to be one to one, the range must have more number of elements than the domain. calculation:. Given a = {1, 2, 3}, b = {3, 4}, c ={4, 5, 6}, find (a × b) ∩ (b × c ). if a = {1, 2, 3}, b = {4}, c = {5}, then verify that: (i) a × ( b ∪ c ) = ( a × b ) ∪ ( a × c ).

8 Let A 1 2 3 4 5 B 3 4 5 6 7 And C Chegg One to one function: a function f:a → b is one to one if every element of the range b corresponds to exactly one element of the domain a of f. important: for a function f:a → b to be one to one, the range must have more number of elements than the domain. calculation:. Given a = {1, 2, 3}, b = {3, 4}, c ={4, 5, 6}, find (a × b) ∩ (b × c ). if a = {1, 2, 3}, b = {4}, c = {5}, then verify that: (i) a × ( b ∪ c ) = ( a × b ) ∪ ( a × c ). If u = {2, 3, 5, 7, 9} is the universal set and a = {3, 7}, b = {2, 5, 7, 9}, then prove that: (i) (a ∪ b)’ = a’ ∩ b’. Let u = { 1, 2, 3, 4, 5, 6, 7, 8, 9 }, a = { 1, 2, 3, 4}, b = { 2, 4, 6, 8 } and c = { 3, 4, 5, 6 }. find (i) a′ (ii) b′ (iii) (a ∪ c)′ (iv) (a ∪ b)′ (v) (a′)′ (vi) (b – c)′. grade. Let r be the relation on a defined by {(a, b): a, b ∈ a, b is exactly divisible by a}. (iii) find the range of r r = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (6, 6)} range of r = set of second elements of relation = {1, 2, 3, 4, 6}. Ex 1.5, 1 (method 1) let u = {1, 2, 3, 4, 5, 6, 7, 8, 9}, a = {1, 2, 3, 4}, b = {2, 4, 6, 8} and c = {3, 4, 5, 6}. find (a ∪ b)’ a ∪ b = {1, 2, 3, 4} ∪ {2, 4, 6, 8} = {1, 2, 3, 4, 6, 8} (a ∪ b)’ = u – (a ∪ b) = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {1,2,3,4,6,8} = {5, 7, 9} ∪ union combination of two sets ex 1.5, 1 (method 2) let.

Solved 1 Let A 1 2 3 4 5 6 7 8 B 2 4 6 8 Chegg If u = {2, 3, 5, 7, 9} is the universal set and a = {3, 7}, b = {2, 5, 7, 9}, then prove that: (i) (a ∪ b)’ = a’ ∩ b’. Let u = { 1, 2, 3, 4, 5, 6, 7, 8, 9 }, a = { 1, 2, 3, 4}, b = { 2, 4, 6, 8 } and c = { 3, 4, 5, 6 }. find (i) a′ (ii) b′ (iii) (a ∪ c)′ (iv) (a ∪ b)′ (v) (a′)′ (vi) (b – c)′. grade. Let r be the relation on a defined by {(a, b): a, b ∈ a, b is exactly divisible by a}. (iii) find the range of r r = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (6, 6)} range of r = set of second elements of relation = {1, 2, 3, 4, 6}. Ex 1.5, 1 (method 1) let u = {1, 2, 3, 4, 5, 6, 7, 8, 9}, a = {1, 2, 3, 4}, b = {2, 4, 6, 8} and c = {3, 4, 5, 6}. find (a ∪ b)’ a ∪ b = {1, 2, 3, 4} ∪ {2, 4, 6, 8} = {1, 2, 3, 4, 6, 8} (a ∪ b)’ = u – (a ∪ b) = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {1,2,3,4,6,8} = {5, 7, 9} ∪ union combination of two sets ex 1.5, 1 (method 2) let.

Solved Let U 1 2 3 4 5 6 7 8 9 A 1 3 4 6 Chegg Let r be the relation on a defined by {(a, b): a, b ∈ a, b is exactly divisible by a}. (iii) find the range of r r = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (6, 6)} range of r = set of second elements of relation = {1, 2, 3, 4, 6}. Ex 1.5, 1 (method 1) let u = {1, 2, 3, 4, 5, 6, 7, 8, 9}, a = {1, 2, 3, 4}, b = {2, 4, 6, 8} and c = {3, 4, 5, 6}. find (a ∪ b)’ a ∪ b = {1, 2, 3, 4} ∪ {2, 4, 6, 8} = {1, 2, 3, 4, 6, 8} (a ∪ b)’ = u – (a ∪ b) = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {1,2,3,4,6,8} = {5, 7, 9} ∪ union combination of two sets ex 1.5, 1 (method 2) let.