
Measure Theory Pdf In these notes we discuss the structure of lebesgue measurable subsets of r from sev eral di erent points of view. along the way, we will see several alternative character izations of measurability which might help to make the concept seem more intuitive. we begin by discussing the measures of open sets. first, recall the following de nition. Elementary measure m(e) is defined to be the sum of these disjoint boxes, and is independent of partition. proof idea. let each endpoint of the elementary set boxes define a new mesh. note, measure defined by discretisation can fail to obey desired properties. consider, for instance, q ∩ [0, 1] and q ∩ ([0, 1] 2).

Real Analysis Show That Inner Measure Is The Same As Outer Measure On 10. measurable cardinals the theory of large cardinals owes its origin to the basic problem of measure theory, the measure problem of h. lebesgue. the measure problem let s be an infinite set. a (nontrivial σ additive probabilistic) measure on s is a real valued function µ on p(s) such that: (i) µ(∅)=0andµ(s)=1; (ii) if x ⊂y,thenµ(x. Definition 1.5. a measurable space (x,a) is a non empty set xequipped with a σ algebra a on x. it is useful to compare the definition of a σ algebra with that of a topology in definition 1.1. there are two significant differences. first, the complement of a measurable set is measurable, but the complement of an open set is not, in general,. Lecture notes taken from the measure and integration lecture given by dr. francesca da lio during spring semester 2021 at eth z ̈urich. with a focus on the theorems and their proofs, these notes have fewer examples than given in the lecture, but the proofs will be more explicit. the exam will be a 20 minute . To that end, we define for any measure µthe collection of measurable sets. we show that the µ measurable sets form a natural algebraic class (theorem 10) and that µ is countably additive on this class (theorem 6).
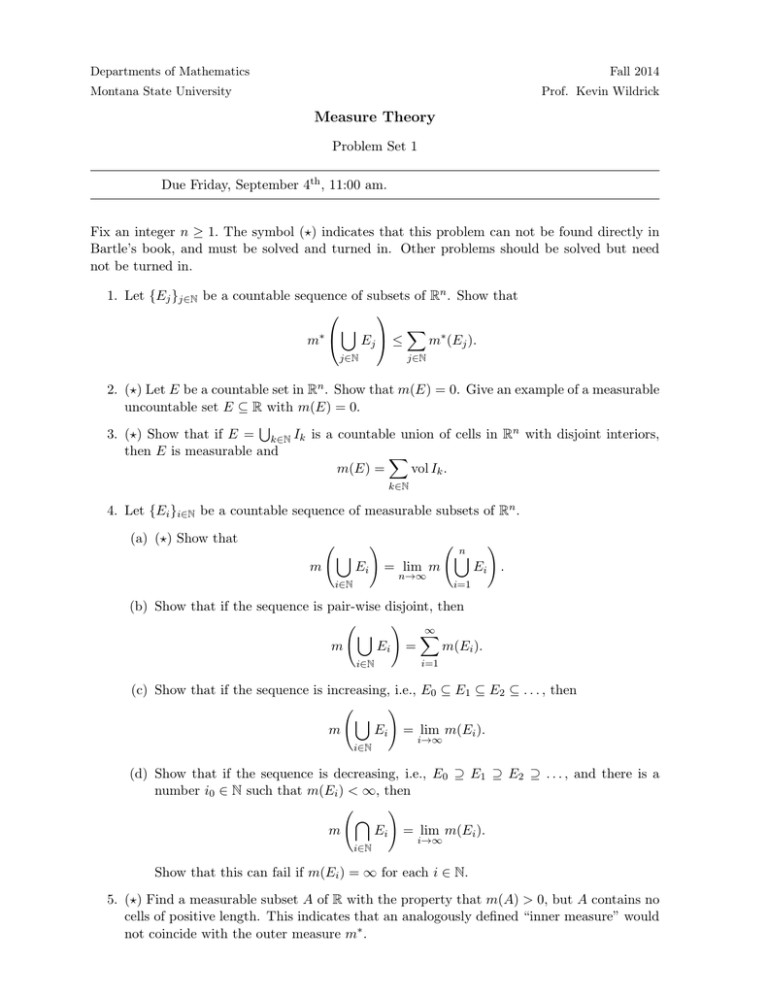
Measure Theory Lecture notes taken from the measure and integration lecture given by dr. francesca da lio during spring semester 2021 at eth z ̈urich. with a focus on the theorems and their proofs, these notes have fewer examples than given in the lecture, but the proofs will be more explicit. the exam will be a 20 minute . To that end, we define for any measure µthe collection of measurable sets. we show that the µ measurable sets form a natural algebraic class (theorem 10) and that µ is countably additive on this class (theorem 6). There is no universal and objective definition of what is a measurable subset of a general space $x$. the general concept of a measurable subset has its origins in the problem of measure in euclidean space: problem of measure: given an object $a\subset\mathbb r^n$, how does one assign a measure $m(a)\in[0,\infty]$ to $a$?. Definition of lebesgue measurable for sets with finite outer measure remove restriction of finite outer measure (r^n, l, lambda) is a measure space, i.e., l is a sigma algebra, and lambda is a measure. Measure theory, 2010 greg hjorth july 8, 2010 contents 1 set theoretical background 2 2 review of topology, metric spaces, and compactness 6 3 the concept of a measure 13 4 the general notion of integration and measurable function 25 5 convergence theorems 32 6 radon nikodym and conditional expectation 38 7 standard borel spaces 44. Measure theory: notes by anwar khan partial contents. these are handwritten notes. we are very thankful to mr. anwar khan for providing these notes. 1. algebra on x 1 2. sigma algebra i.e. ˙ algebra on x 3 3.

Solution Measure Theory Studypool There is no universal and objective definition of what is a measurable subset of a general space $x$. the general concept of a measurable subset has its origins in the problem of measure in euclidean space: problem of measure: given an object $a\subset\mathbb r^n$, how does one assign a measure $m(a)\in[0,\infty]$ to $a$?. Definition of lebesgue measurable for sets with finite outer measure remove restriction of finite outer measure (r^n, l, lambda) is a measure space, i.e., l is a sigma algebra, and lambda is a measure. Measure theory, 2010 greg hjorth july 8, 2010 contents 1 set theoretical background 2 2 review of topology, metric spaces, and compactness 6 3 the concept of a measure 13 4 the general notion of integration and measurable function 25 5 convergence theorems 32 6 radon nikodym and conditional expectation 38 7 standard borel spaces 44. Measure theory: notes by anwar khan partial contents. these are handwritten notes. we are very thankful to mr. anwar khan for providing these notes. 1. algebra on x 1 2. sigma algebra i.e. ˙ algebra on x 3 3.
