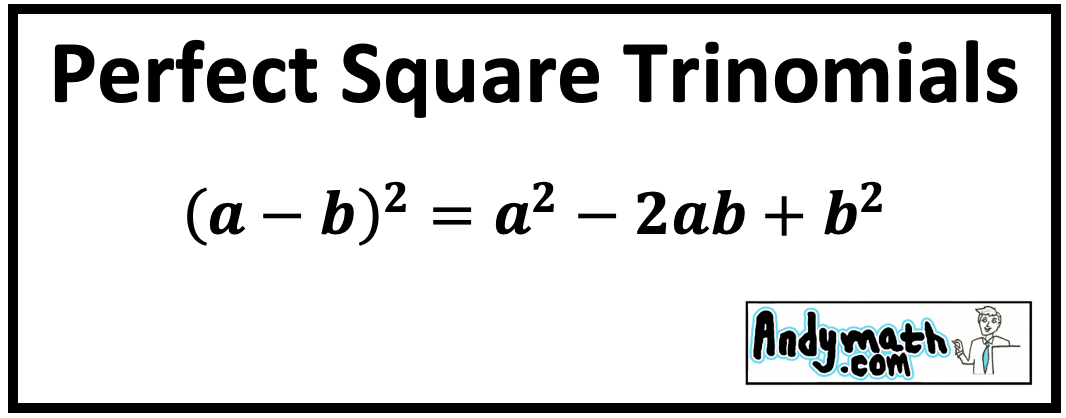
Perfect Square Trinomials Perfect square trinomial generally has the pattern of a 2 2ab b 2 or a 2 2ab b 2. given a binomial, to find the perfect square trinomial, we follow the steps given below. they are, step 1: find the square the first term of the binomial. step 2: multiply the first and the second term of the binomial with 2. Does this fit the pattern of a perfect square trinomial? yes. both x 2 and 36 are perfect squares. and 12x is twice the product of x and 6. since all signs are positive, the pattern is (a b) 2 = a 2 2ab b 2. let a = x and b = 6. answer: (x 6) 2 or (x 6)(x 6).

Factoring Perfect Square Trinomials In these lessons, we will learn how to factor perfect square trinomials. the following diagrams show the factoring and expanding of perfect square trinomials. scroll down the page for examples and solutions of factoring perfect square trinomials. in some cases recognizing some common patterns in the trinomial will help you to factor it faster. Learning to identify certain patterns in polynomials helps you factor some “special cases” of polynomials quickly. the special cases are: trinomials that are perfect squares, \(\ a^{2} 2 a b b^{2}\) and \(\ a^{2} 2 a b b^{2}\), which factor as \(\ (a b)^{2}\) and \(\ (a b)^{2}\), respectively;. How to: given a perfect square trinomial, factor it into the square of a binomial. confirm that the first and last term are perfect squares. confirm that the middle term is twice the product of [latex]ab[ latex]. write the factored form as [latex]{\left(a b\right)}^{2}[ latex] or [latex]{\left(a b\right)}^{2}[ latex]. The first term, 4x 2, is the square of 2x, and the last term, 36, is the square of 6 (or, in this case, −6, if this is a perfect square). according to the pattern for perfect square trinomials, the middle term must be:.

Showme Perfect Square Trinomials How to: given a perfect square trinomial, factor it into the square of a binomial. confirm that the first and last term are perfect squares. confirm that the middle term is twice the product of [latex]ab[ latex]. write the factored form as [latex]{\left(a b\right)}^{2}[ latex] or [latex]{\left(a b\right)}^{2}[ latex]. The first term, 4x 2, is the square of 2x, and the last term, 36, is the square of 6 (or, in this case, −6, if this is a perfect square). according to the pattern for perfect square trinomials, the middle term must be:. Answer: a perfect square trinomial has a specific pattern: `ax^2 bx c` where the first and last terms are perfect squares, and the middle term is twice the product of the square roots of the first and last terms. In this lesson you will learn how to recognize patterns for factoring polynomials. factoring by patterns means recognizing that polynomials having a certain form will always factor in a specific way. perfect square trinomials, differences of two squares, and differences and sums of two cubes can all be factored using patterns. these patterns are. Examples of factoring perfect square trinomial. example 1: factor [latex]{x^2} 8x 16[ latex] let’s examine if the given trinomial meets the requirements. the first term [latex]x^2[ latex] is a perfect square since it can be written as [latex]x^2=(x)^2[ latex]. In some special cases, it is possible to factor polynomials using the perfect square trinomial. this technique allows us to factor these types of polynomials easily and use factoring to find the roots or solutions to a problem. here, we will do a brief review of the perfect square trinomial.

Perfect Square Trinomials Worksheet Collection For Teaching Learning Answer: a perfect square trinomial has a specific pattern: `ax^2 bx c` where the first and last terms are perfect squares, and the middle term is twice the product of the square roots of the first and last terms. In this lesson you will learn how to recognize patterns for factoring polynomials. factoring by patterns means recognizing that polynomials having a certain form will always factor in a specific way. perfect square trinomials, differences of two squares, and differences and sums of two cubes can all be factored using patterns. these patterns are. Examples of factoring perfect square trinomial. example 1: factor [latex]{x^2} 8x 16[ latex] let’s examine if the given trinomial meets the requirements. the first term [latex]x^2[ latex] is a perfect square since it can be written as [latex]x^2=(x)^2[ latex]. In some special cases, it is possible to factor polynomials using the perfect square trinomial. this technique allows us to factor these types of polynomials easily and use factoring to find the roots or solutions to a problem. here, we will do a brief review of the perfect square trinomial.
