
Permutation Group Pdf Cayley’s theorem: every group is isomorphic to a group of permutations. proof: given a group we will find a 1 1 map 𝜙: →𝑆𝐺, where 𝑆𝐺 is the group of permutations of , such that 𝜙( )=𝜙( )𝜙( )for all , ∈ . then by our lemma will be isomorphic to 𝜙[ ]≤𝑆𝐺. One way to write a permutation is to show where each element goes. for example, suppose σ = 1 2 3 4 5 6 3 2 4 1 6 5 ∈ s6. i’ll refer to this as permutation notation. this means that σ(1) = 3,σ(2) = 2,σ(3) = 4,σ(4) = 1,σ(5) = 6,σ(6) = 5. thus, the identity permutation in s6 is id = 1 2 3 4 5 6 1 2 3 4 5 6 . 3.

Permutation And Combination Pdf Pdf Permutation Alphabet Permutation groups de–nition a permutation of a nonempty set a is a function s: a !a that is one to one and onto. in other words, a pemutation of a set is a rearrangement of the elements of the set. theorem let a be a nonempty set and let s a be the collection of all permutations of a. then (s a, ) is a group, where is the function. In the part of the course, we will be looking at actions of groups on various combinatorial objects. we will mostly focus on transitive groups and will look at primitive and imprimitive actions, before turning our attention to multiply transitive actions. In mathematics, a permutation group is a group g whose elements are permutations of a given set m, and whose group operation is the composition of permutations in g (which are thought of as bijective functions from the set m to itself); the relationship is often written as (g,m). Symmetric groups and their subgroups are permutation groups. historically, it was the study of permutation groups and groups of transformations that gave the foundation to group theory. a lot of work in the study of permutations was undertaken by several european mathematicians in the 18th century.

Permutation And Combination Maths Download Free Pdf I Phone In mathematics, a permutation group is a group g whose elements are permutations of a given set m, and whose group operation is the composition of permutations in g (which are thought of as bijective functions from the set m to itself); the relationship is often written as (g,m). Symmetric groups and their subgroups are permutation groups. historically, it was the study of permutation groups and groups of transformations that gave the foundation to group theory. a lot of work in the study of permutations was undertaken by several european mathematicians in the 18th century. Remark 2.7. cayley’s theorem gives one group of permutations that a given group is isomorphic to. it does not claim to give the smallest symmetric group containing a subgroup isomorphic to the group we started with. for example, we saw that d n is isomorphic to a subgroup of s zn ∼=s n, while cayley’s theorem exhibits an isomorphism between d. Permutation groups h.w. lenstra, fall 2007 math.leidenuniv.nl ˘streng permutation index solvable groups. let gbe a group. we de ne the sequence g(0) ˙ g(1) ˙ g(2) ˙ ::: of subgroups of ginductively by g(0) = gand g(i 1) = [g(i);g(i)]. the group gis called solvable if g(t) = f1g for some t2 z 0. exercise 1. Although a group is an abstract mathematical entity, it’s easier to explain group operations if we have in mind a specific representation. the set of permutations of n objects constitute a group sn. we can see that sn satisfies the group axioms as follows: (1) for any two permutations si and sj, their product sisj is also in the group.

Permutation 12 Examples Format How To Write Pdf Remark 2.7. cayley’s theorem gives one group of permutations that a given group is isomorphic to. it does not claim to give the smallest symmetric group containing a subgroup isomorphic to the group we started with. for example, we saw that d n is isomorphic to a subgroup of s zn ∼=s n, while cayley’s theorem exhibits an isomorphism between d. Permutation groups h.w. lenstra, fall 2007 math.leidenuniv.nl ˘streng permutation index solvable groups. let gbe a group. we de ne the sequence g(0) ˙ g(1) ˙ g(2) ˙ ::: of subgroups of ginductively by g(0) = gand g(i 1) = [g(i);g(i)]. the group gis called solvable if g(t) = f1g for some t2 z 0. exercise 1. Although a group is an abstract mathematical entity, it’s easier to explain group operations if we have in mind a specific representation. the set of permutations of n objects constitute a group sn. we can see that sn satisfies the group axioms as follows: (1) for any two permutations si and sj, their product sisj is also in the group.
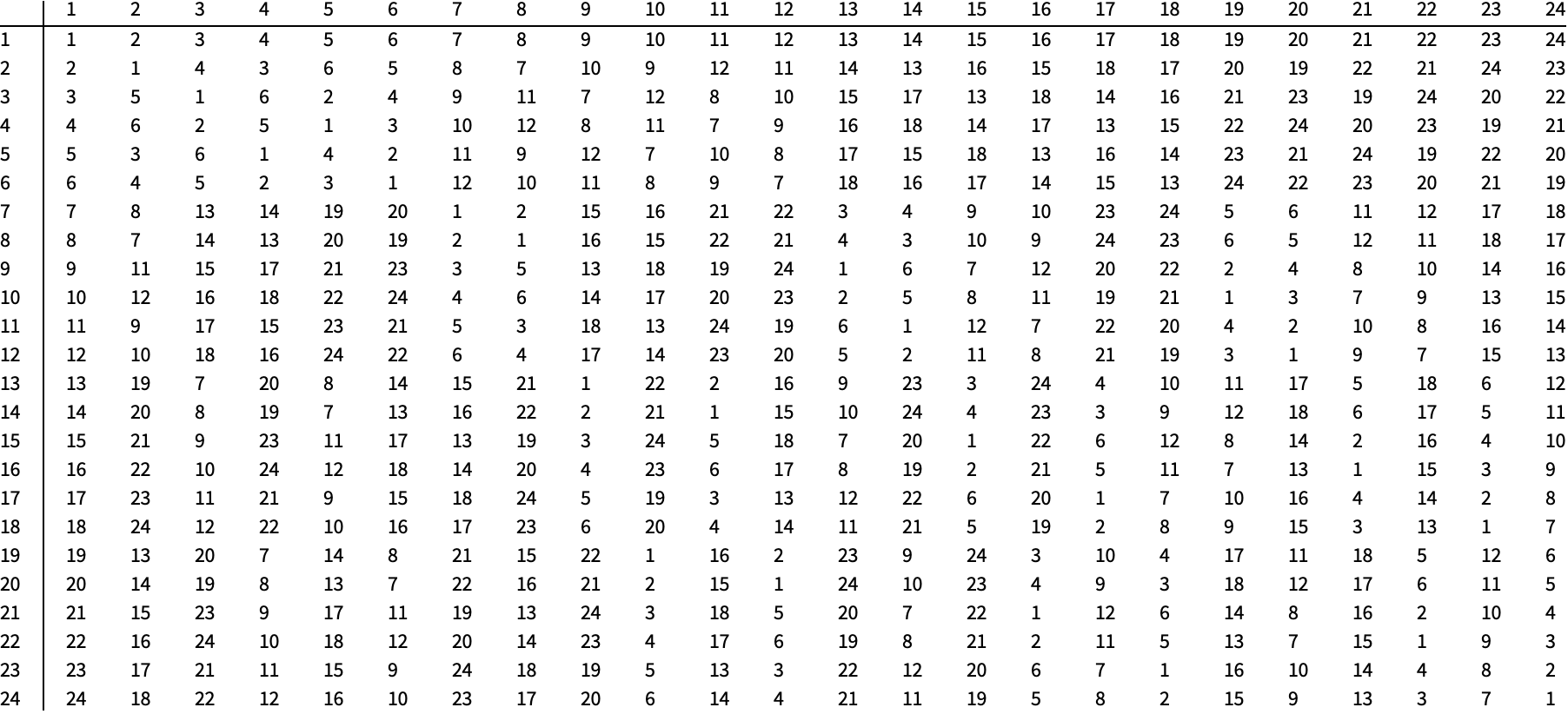
Permutation Groups Wolfram Language Documentation Although a group is an abstract mathematical entity, it’s easier to explain group operations if we have in mind a specific representation. the set of permutations of n objects constitute a group sn. we can see that sn satisfies the group axioms as follows: (1) for any two permutations si and sj, their product sisj is also in the group.
