
Permutation Part 2 Pdf Permutation Mathematics In how many ways can the letters of the word success be arranged so that all ss are together? solution : let us consider all "s" together as one unit. "c" is repeating 2 times, both "e" and "u" is repeating once. total number of letters = 5 (sss as 1) = 5! (2!) = 60. hence the total number of words = 60. question 4 : a coin is tossed 8 times,. 14.1 part ii permutations and 14.2 permutations with repetitions & circular permutations notes 1. permutation: a permutation of n differenct elements is an ordering of the elements such that one.

Practice Problems In Permutations Analyzing Products Orders Parity How many different combinations of 1st, 2 nd, and 3rd place winners can there be? this is a permutation problem, because we are looking for the number of groups of winners. consider the three positions, and how many choices there are for each position: there are 20 choices for 1st place, 19 for 2nd place, and 18 for 3rd place. multiply to get 6840. Permutations with repetition given a set of \(n\) objects such that there are \(n 1\) identical objects of type 1, \(n 2\) identical objects of type 2, \(\ldots\), and \(n k\) identical objects of type \(k\), how many distinct permutations of the objects are there?. Here you’ll learn how to solve problems for the special case where there are double objects or repetitions within a permutation situation. you’re having a party at your house this weekend and you’ve stocked up on soft drinks. Permutations with repetition math problems. solved word math problems, tests, exercises, and preparation for exams. math questions with answers and solved math homework. problems count 36.

Permutation And Combinations Problems Pdf Numbers Rowing Sport Here you’ll learn how to solve problems for the special case where there are double objects or repetitions within a permutation situation. you’re having a party at your house this weekend and you’ve stocked up on soft drinks. Permutations with repetition math problems. solved word math problems, tests, exercises, and preparation for exams. math questions with answers and solved math homework. problems count 36. Arrangements or permutations eg 1. a maths debating team consists of 4 speakers. a) in how many ways can all 4 speakers be arranged in a row for a photo? b) how many ways can the captain and vice captain be chosen? solution : 4.3.2.1 = 4! or 4p4 solution : 4.3 = 12 or 4p2. Learn how to differentiate between permutations and combinations. scenarios to help distinguish permutation type problems vs. combination type problems. try the free mathway calculator and problem solver below to practice various math topics. Repetition problems when repetition of elements is permitted, we can calculate the number of permutations using the fundamental counting principle, which essentially takes the following format: 𝑃=𝑛𝑟 example 2: suppose we want to create a four digit pin using the digits 0 6. how many different pins can be generated if:. Permutations . a pemutation is a sequence containing each element from a finite set of n elements once, and only once. permutations of the same set differ just in the order of elements. p(n) = n! permutations with repetition n 1 – # of the same elements of the first cathegory n 2 # of the same elements of the second cathegory.
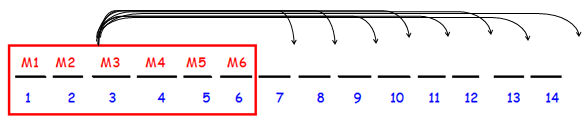
Permutation With Repetition Problems With Solutions Arrangements or permutations eg 1. a maths debating team consists of 4 speakers. a) in how many ways can all 4 speakers be arranged in a row for a photo? b) how many ways can the captain and vice captain be chosen? solution : 4.3.2.1 = 4! or 4p4 solution : 4.3 = 12 or 4p2. Learn how to differentiate between permutations and combinations. scenarios to help distinguish permutation type problems vs. combination type problems. try the free mathway calculator and problem solver below to practice various math topics. Repetition problems when repetition of elements is permitted, we can calculate the number of permutations using the fundamental counting principle, which essentially takes the following format: 𝑃=𝑛𝑟 example 2: suppose we want to create a four digit pin using the digits 0 6. how many different pins can be generated if:. Permutations . a pemutation is a sequence containing each element from a finite set of n elements once, and only once. permutations of the same set differ just in the order of elements. p(n) = n! permutations with repetition n 1 – # of the same elements of the first cathegory n 2 # of the same elements of the second cathegory.
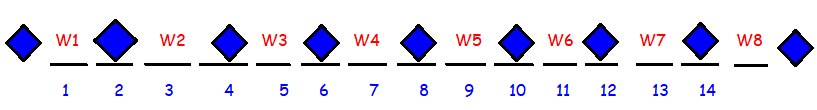
Permutation With Repetition Problems With Solutions Repetition problems when repetition of elements is permitted, we can calculate the number of permutations using the fundamental counting principle, which essentially takes the following format: 𝑃=𝑛𝑟 example 2: suppose we want to create a four digit pin using the digits 0 6. how many different pins can be generated if:. Permutations . a pemutation is a sequence containing each element from a finite set of n elements once, and only once. permutations of the same set differ just in the order of elements. p(n) = n! permutations with repetition n 1 – # of the same elements of the first cathegory n 2 # of the same elements of the second cathegory.
Solved Give An Example Permutation Without Repetition Math
