
Players Compete In A Tournament Every One Plays Everyone Else Just 8 players compete in a tournament,every one plays everyone else just once. the winner of the game gets 1, the loser 0 or, each gets $\frac{1}{2}$ if draw. the final result is that every one gets a different score and the player placing second gets the same score as the total of the four bottom players. To ask unlimited maths doubts download doubtnut from goo.gl 9wzjcw 8 players compete in a tournament, everyone plays everyone else just once. the.
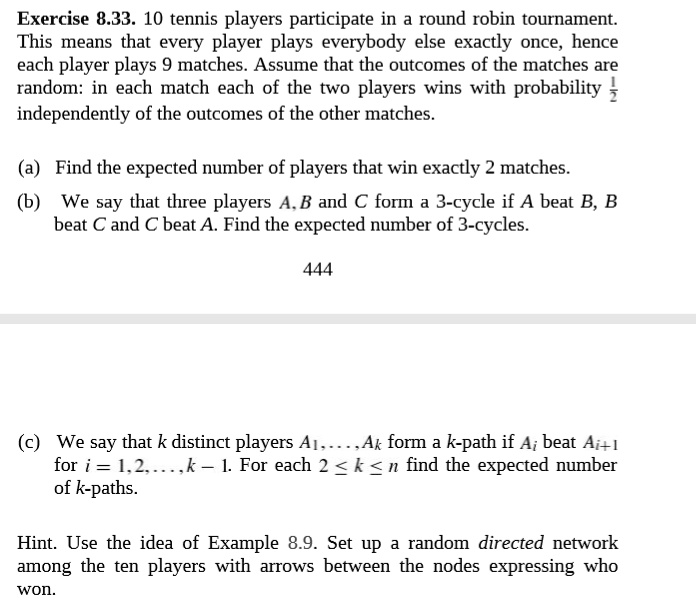
Solved Exercise 8 33 10 Tennis Players Participate In A Round Robin 8 players compete in a tournament, every one plays everyone else just once. the winner of a game gets 1, the loser 0 or each gets 1 2 if the game is drawn. the final result is that every one gets a different score and the player playing placing second gets the same as the total of four bottom players.the total score of all the players is. Everyone plays everyone else just once. the winner of a game gets 1, the loser 0, or each gets 1 2 if the game is drawn. the final result is that everyone gets a different score and the player placing second gets the same as the total of the four bottom players. Is it possible in general to seat players so that everyone plays against everyone else exactly once during the tournament? the background is that yesterday i took part in a tournament in a three player game, with 15 participants. after five rounds i'm pretty certain no one had met anyone else twice, and i was curious whether it would possible. I would like to create a 4 on 4 tournament with 8 players (4 players on a team where two teams play against each other each game), where every player plays with every other player an equal number of times. a simple example of this would be if you had a 2 on 2 tournament with 4 players then: 12 v 34. 13 v 24. 14 v 23.

Solved 12 Players Compete In A Tournament And Are Ranked Chegg Is it possible in general to seat players so that everyone plays against everyone else exactly once during the tournament? the background is that yesterday i took part in a tournament in a three player game, with 15 participants. after five rounds i'm pretty certain no one had met anyone else twice, and i was curious whether it would possible. I would like to create a 4 on 4 tournament with 8 players (4 players on a team where two teams play against each other each game), where every player plays with every other player an equal number of times. a simple example of this would be if you had a 2 on 2 tournament with 4 players then: 12 v 34. 13 v 24. 14 v 23. In a round robin tournament, everyone plays everyone else. this leads to a huge number of matches, but it also gives a guaranteed number of matches for everyone. Everyone plays everyone else just once. the winner of a game gets 1, the loser 0, or each gets 1 2 if the game is drawn. the final result is that everyone gets a different score and the player placing second gets the same as the total of the four bottom players. Out of these, four players namely abhishek, bharat, hitesh and ashutosh decide to play a game "pirate bay ii" tournament after the end of this tournament. the tournament is played on the round robin basis where each player trades with the other player once. all trades produced a winner. The problem of scheduling a tournament in which all participants compete against each other one on one in a series of rounds probably goes back to pre history. this page presents some thoughts of the author on the problem and some software to explore various ways to look at it.

Solved N Players Compete In A Tournament And Are Ranked From Chegg In a round robin tournament, everyone plays everyone else. this leads to a huge number of matches, but it also gives a guaranteed number of matches for everyone. Everyone plays everyone else just once. the winner of a game gets 1, the loser 0, or each gets 1 2 if the game is drawn. the final result is that everyone gets a different score and the player placing second gets the same as the total of the four bottom players. Out of these, four players namely abhishek, bharat, hitesh and ashutosh decide to play a game "pirate bay ii" tournament after the end of this tournament. the tournament is played on the round robin basis where each player trades with the other player once. all trades produced a winner. The problem of scheduling a tournament in which all participants compete against each other one on one in a series of rounds probably goes back to pre history. this page presents some thoughts of the author on the problem and some software to explore various ways to look at it.
