
Power Series Method Pdf Power Series Calculus Convergent power series can be added and multiplied together, and multiplied by constants using the following rules. firstly, we can add series by adding term by term,. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3.
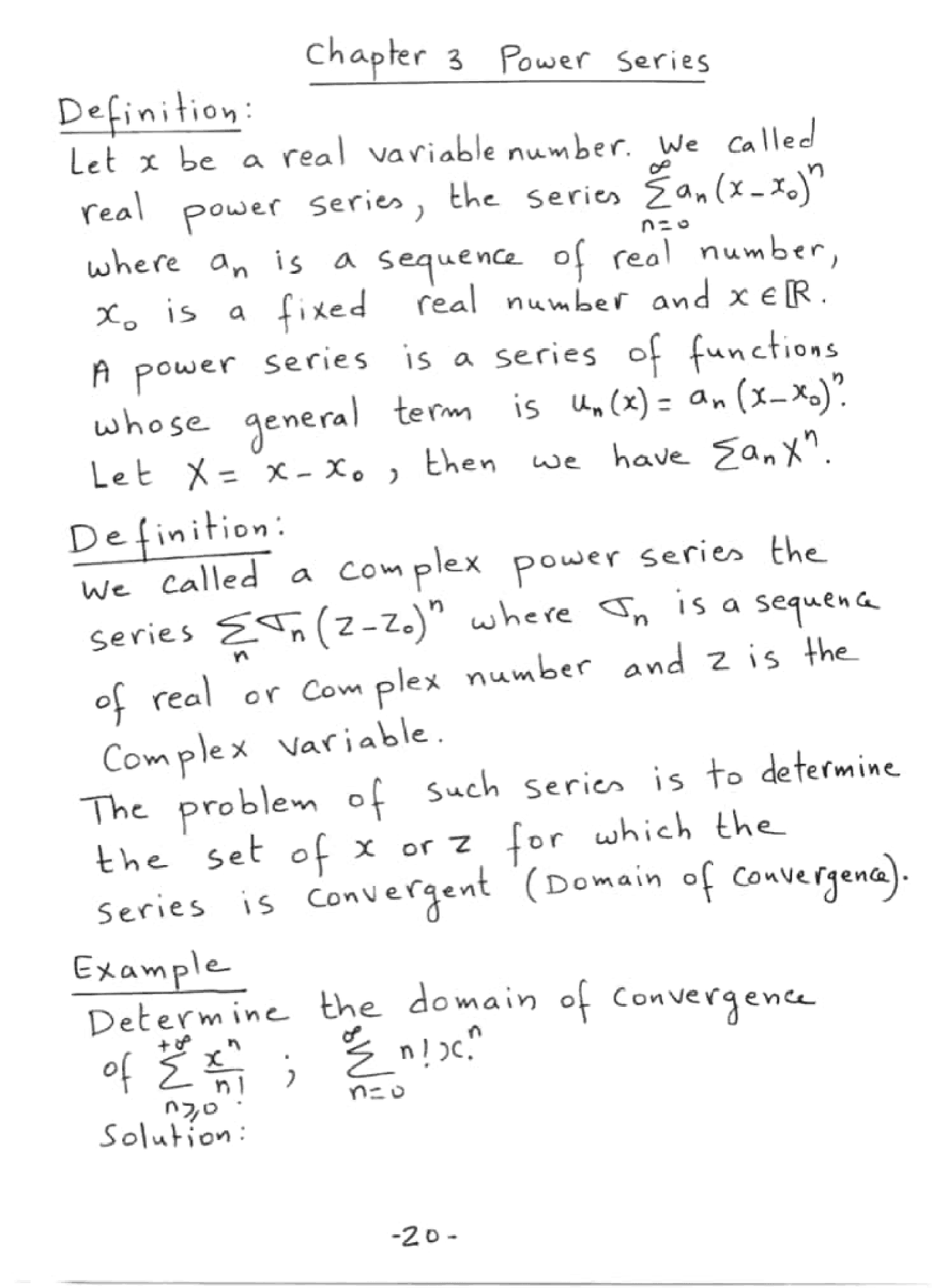
Analysis Calculus Power Series Docsity Given the power series y(x) = x∞ n=0 c n (x − x 0)n, introduce the number l = lim n→∞ |c n 1| |c n|. then, the following statements hold: (1) the power series converges in the domain |x − x 0|l < 1. (2) the power series diverges in the domain |x − x 0|l > 1. (3) the power series may or may not converge at |x − x 0|l = 1. therefore. 1. there exists r > 0 such that the series diverges for |x − a| > r, but converges for |x− a| < r. the series may or may not converge at the endpoints 2. the series converges for every x (r =. A power series (centered at 0) is a series of the form ∑∞ n=0 anx n = a 0 a1x a2x 2 ··· a nx n . where the an are some coefficients. if all but finitely many of the an are zero, then the power series is a polynomial function, but if infinitely many of the an are nonzero, then we need to consider the convergence of the power series. Lecture 30: power series a power series is a series of the form x1 n=0 c nx n = c 0 c 1x c 2x 2 c 3x 3 ::: where x is a variable, the c n’s are constants called the coe cients of the series. example x1 n=0 xn 2n = 1 x 2 x2 22 x3 23 ::: a power series may converge for some values of x and may diverge for others. example in the.
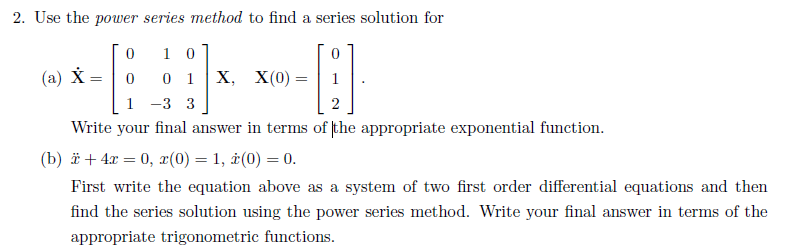
Solved 2 Use The Power Series Method To Find A Series Chegg A power series (centered at 0) is a series of the form ∑∞ n=0 anx n = a 0 a1x a2x 2 ··· a nx n . where the an are some coefficients. if all but finitely many of the an are zero, then the power series is a polynomial function, but if infinitely many of the an are nonzero, then we need to consider the convergence of the power series. Lecture 30: power series a power series is a series of the form x1 n=0 c nx n = c 0 c 1x c 2x 2 c 3x 3 ::: where x is a variable, the c n’s are constants called the coe cients of the series. example x1 n=0 xn 2n = 1 x 2 x2 22 x3 23 ::: a power series may converge for some values of x and may diverge for others. example in the. Power series solutions tsogtgerel gantumur abstract. we introduce the power series solution method for second order linear di erential equations, and illustrate it by examples. contents 1. ordinary and singular points1 2. the fundamental theorem3 3. formulation of the method4 4. examples 5 1. ordinary and singular points consider the di. Power series: convergence analysis a power series is a series of functions of the form x 7! x∞ n=0 an(x x0)n (|) where an’s are constants called coefficientsand x0 is also a constant. note. Power series method – an example. y′′ = x y. (10) this equation looks very simple but its general solution cannot be written in “closed form”. on the other hand, these solutions are very useful in practice that they were given a name and became one class of the so called “special functions”. 1. write power series expansion for y: an xn. This gives us a power series representation for the function g(x) on the interval ( 1;1). note that the function g(x) here has a larger domain than the power series.

Solved Use The Method Of Power Series To Find A Solution Of Chegg Power series solutions tsogtgerel gantumur abstract. we introduce the power series solution method for second order linear di erential equations, and illustrate it by examples. contents 1. ordinary and singular points1 2. the fundamental theorem3 3. formulation of the method4 4. examples 5 1. ordinary and singular points consider the di. Power series: convergence analysis a power series is a series of functions of the form x 7! x∞ n=0 an(x x0)n (|) where an’s are constants called coefficientsand x0 is also a constant. note. Power series method – an example. y′′ = x y. (10) this equation looks very simple but its general solution cannot be written in “closed form”. on the other hand, these solutions are very useful in practice that they were given a name and became one class of the so called “special functions”. 1. write power series expansion for y: an xn. This gives us a power series representation for the function g(x) on the interval ( 1;1). note that the function g(x) here has a larger domain than the power series.

Power Series Representations Of Functions Techniques For Finding Power series method – an example. y′′ = x y. (10) this equation looks very simple but its general solution cannot be written in “closed form”. on the other hand, these solutions are very useful in practice that they were given a name and became one class of the so called “special functions”. 1. write power series expansion for y: an xn. This gives us a power series representation for the function g(x) on the interval ( 1;1). note that the function g(x) here has a larger domain than the power series.

Calculus Finding Power Series Any Explanation Would Be Incredibly
