
Power Series Representations Of Functions Techniques For Finding To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. however, use of this formula does quickly illustrate how functions can be represented as a power series. we also discuss differentiation and integration of power series. In this section we define power series and show how to determine when a power series converges and when it diverges. we also show how to represent certain functions using power series. a series of the form. ∞ ∑ n = 0cnxn = c0 c1x c2x2 …, where x is a variable and the coefficients cn are constants, is known as a power series. the series.

9 9 Representations Of Functions By Power Series First, we will look at techniques which will allow us to obtain a series rep resentation by using known series representations. the techniques involved are substitution, di¤erentiation and integration. Power series representations of functions can be systematically derived by manipulating the geo metric series formula. the process involves the following steps: this is a foundational representation for f(x) = , valid on the interval ( 1; 1). the series for related functions. rive series for functions such as ln(1 x), tan 1 x, or similar. Substitution first, we examine how to use the power series representation of the function g(x) = 1=(1 x) on the interval ( 1;1) to derive a power series representation of other functions on an interval. example find a power series representation of the function given below and nd the interval of convergence of the series. f(x) = 1 1 x7: ii we. We calculate the power series representations centered about zero for most of the elementary functions. from these so called maclaurin series we can build many other examples through substitution and series multiplication. sections 13.4 and 13.5 are devoted to illustrating the utility of power series in mathematical calculation.

Solved Representations Of Functions As Power Series Chegg Substitution first, we examine how to use the power series representation of the function g(x) = 1=(1 x) on the interval ( 1;1) to derive a power series representation of other functions on an interval. example find a power series representation of the function given below and nd the interval of convergence of the series. f(x) = 1 1 x7: ii we. We calculate the power series representations centered about zero for most of the elementary functions. from these so called maclaurin series we can build many other examples through substitution and series multiplication. sections 13.4 and 13.5 are devoted to illustrating the utility of power series in mathematical calculation. Find a power series representation for the function and determine the radius and interval of conver gence. note: when differentiating a power series, the starting index will change if the first term is constant. when integrating a power series, the starting index does not change under any circumstances. f′(x) and n! z f(x) dx. z 3. evaluate. Find a geometric power series that represents a function. construct a power series using series operations. in this section and the next, you will study several techniques for finding a power series that represents a given function. consider the function given by f x bles the sum of a geometric series. the form of f closely resem r 1 , a r < 1. Differentiation and integration are useful techniques for finding power series representations of functions. differentiation and integration of power series works in a way very similar to handling polynomials: look at the series term by term. for instance, look at the power series. How can we represent common functions as power series, and why would we want to do so? the next two sections provide partial answers to these questions. in this section we start with a function defined by a geometric series and show how we can obtain power series representations for several related functions. and we look at a few ways in which.
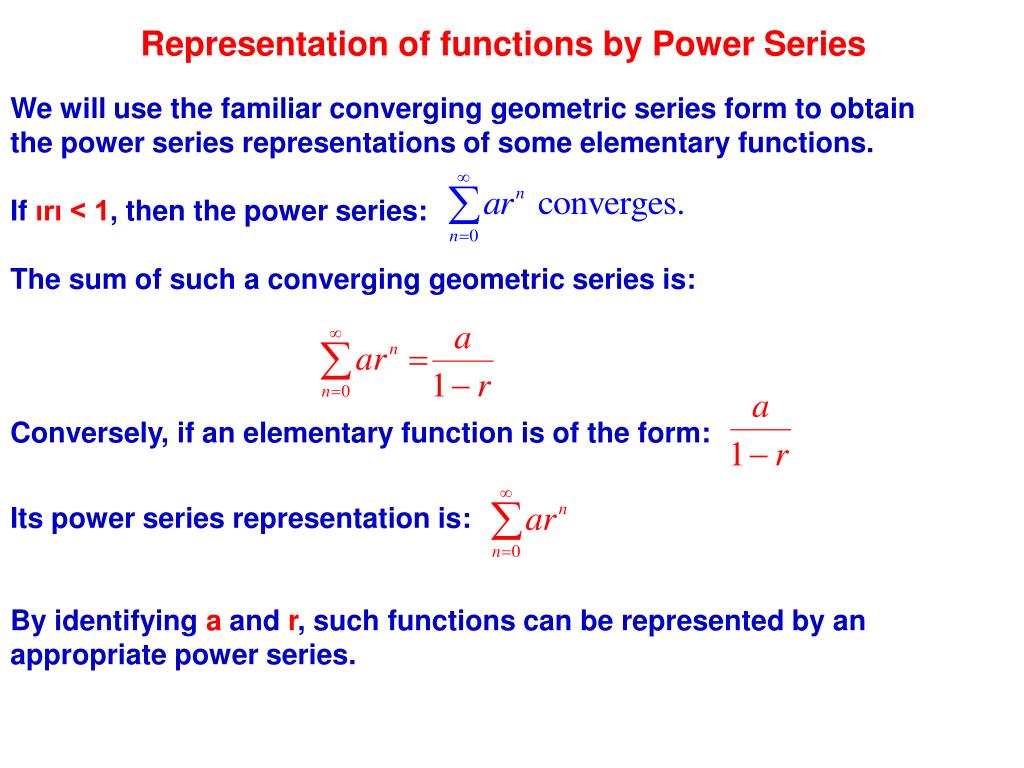
Ppt Representation Of Functions By Power Series Powerpoint Find a power series representation for the function and determine the radius and interval of conver gence. note: when differentiating a power series, the starting index will change if the first term is constant. when integrating a power series, the starting index does not change under any circumstances. f′(x) and n! z f(x) dx. z 3. evaluate. Find a geometric power series that represents a function. construct a power series using series operations. in this section and the next, you will study several techniques for finding a power series that represents a given function. consider the function given by f x bles the sum of a geometric series. the form of f closely resem r 1 , a r < 1. Differentiation and integration are useful techniques for finding power series representations of functions. differentiation and integration of power series works in a way very similar to handling polynomials: look at the series term by term. for instance, look at the power series. How can we represent common functions as power series, and why would we want to do so? the next two sections provide partial answers to these questions. in this section we start with a function defined by a geometric series and show how we can obtain power series representations for several related functions. and we look at a few ways in which.
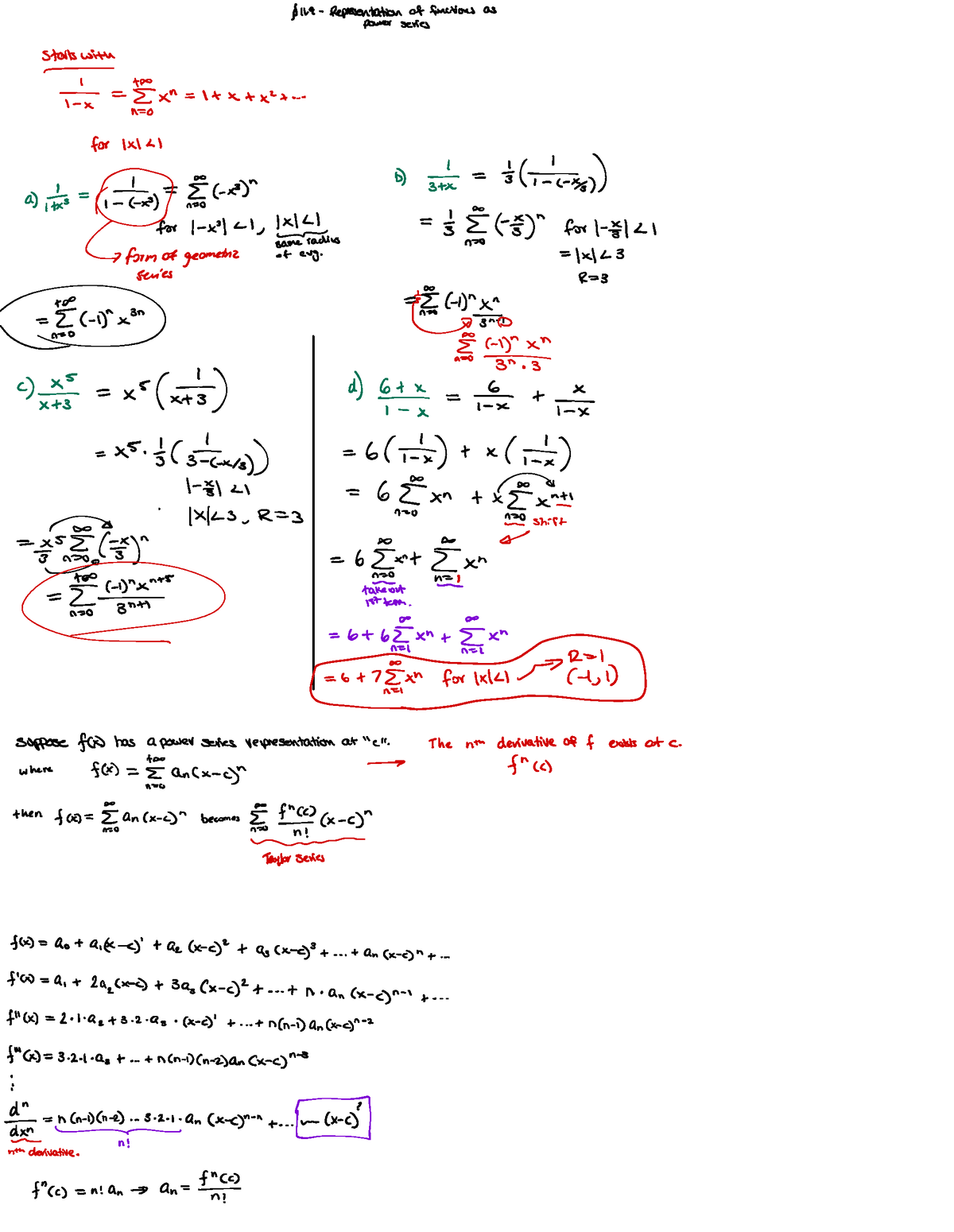
Representation Of Functions As Power Series 11 11 Representation Differentiation and integration are useful techniques for finding power series representations of functions. differentiation and integration of power series works in a way very similar to handling polynomials: look at the series term by term. for instance, look at the power series. How can we represent common functions as power series, and why would we want to do so? the next two sections provide partial answers to these questions. in this section we start with a function defined by a geometric series and show how we can obtain power series representations for several related functions. and we look at a few ways in which.
