
Problem 4 Let V1 2 1 0 1 V2 0 1 2 1 And Chegg There are 2 steps to solve this one. given vectors, v 1 = (− 2, 1, 0, − 1), v 2 = (0, 1, − 2, 1) and b = (1, 3, − 1, − 1). also w is the subspace of r 4 spanned by v 1 and v 2. not the question you’re looking for? post any question and get expert help quickly. Let v be the subspace of r4 generated by the vectors v1 = (1; 1; 0; 0); v2 = (0; 1; 1; 0); v3 = (0; 0; 1; 1) and w generated by the vectors w1 = (1; 0; 1; 0); w2 = (0; 2; 1; 1); w3 = (1; 2; 1; 2) in r4. solution. dim(v ) = 3 since v = spanfv1; v2; v3g and the vectors v1; v2; v3 are lin. independent.
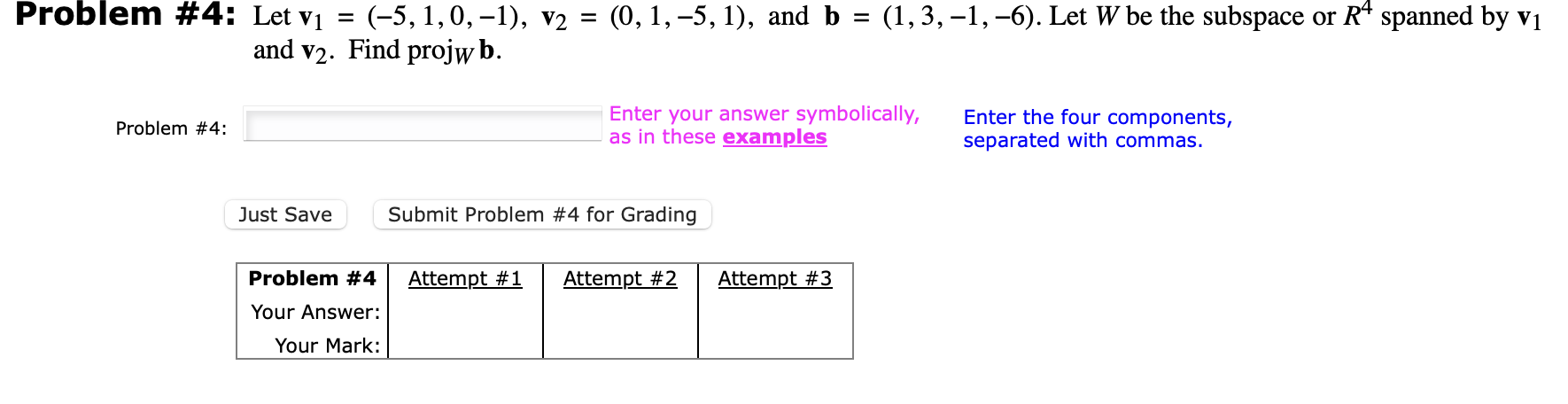
Problem 4 Let V1 5 1 0 1 V2 0 1 5 1 ï And Chegg Let v1=(2,−2,1) and v2=(2,−1,1). select all vectors below in the span of v1 and v2. the answer choices are a. (0,−1,0) b. (0,0,0) c. (2,−3,1) d. (1,−3,0). Problem \#4: let v1= (−2,1,0,−1),v2= (0,1,−2,1), and b= (1,1,−1,−4). let w be the subspace or r4 spanned by v1 and v2. find projwb. enter your answer problem \#4: symbolically, as in these enter the four components, examples separated with commas. your work has been saved!. Let x (t) and y (t) determine two quadratic bézier curves, with control points {p0, p1, p2} and {p2, p3, p4}, respectively. the curves are joined at p2 = x (1) = y (0). a. suppose the combined curve has g^1 continuity (at p2). what algebraic restriction does this condition impose on the control points?. Example 2 1. let v1 = (1;2;3);v2 = (1;0;2). (a) express u = ( 1;2; 1) as a linear combi nation of v1 and v2, we must nd scalars a1 and a2 such that u = a1v1 a2v2. thus a1 a2 = 1 2a1 0a2 = 2 3a1 2a2 = 1 this is 3 equations in the 2 unknowns a1, a2. solving for a1, a2: 0 b @ 1 1 1 2 0 2 3 2 1 1 c a r2! r2 2r1 r3! r3 3r1 0 b @ 1 1 1 0 2 4.

Let V1 4 6 7 T V2 0 1 1 T V3 0 1 2 T ï Let Chegg Let x (t) and y (t) determine two quadratic bézier curves, with control points {p0, p1, p2} and {p2, p3, p4}, respectively. the curves are joined at p2 = x (1) = y (0). a. suppose the combined curve has g^1 continuity (at p2). what algebraic restriction does this condition impose on the control points?. Example 2 1. let v1 = (1;2;3);v2 = (1;0;2). (a) express u = ( 1;2; 1) as a linear combi nation of v1 and v2, we must nd scalars a1 and a2 such that u = a1v1 a2v2. thus a1 a2 = 1 2a1 0a2 = 2 3a1 2a2 = 1 this is 3 equations in the 2 unknowns a1, a2. solving for a1, a2: 0 b @ 1 1 1 2 0 2 3 2 1 1 c a r2! r2 2r1 r3! r3 3r1 0 b @ 1 1 1 0 2 4. In r ^4 4, let v1 = (1, 1,2 , 1), v2 = (2 , 1,2, 0), v3 = (1 , 0, 2, 0), and v4 = (1 , 0, 3, 1). show that the set {v1 , v2, v3, v4} is affinely independent. in this problem, we are tasked to show that the set \ {\bold v 1, \bold v 2, \bold v 3, \bold v 4\} {v1,v2,v3,v4} is affinely independent. we are given the points. There are 4 steps to solve this one. consider a matrix a = [1 2 3 4 0 − 1 − 1 − 1 2 − 1 1 3] whose columns are vectors v 1, v 2, v 3, v 4 written as column vectors. (a) hence col (a)=column spac not the question you’re looking for? post any question and get expert help quickly. Let v 1 = 0 0 −4, v 2 = 0 −4 12, and v 3 = 6 −5 −8. do v1, v2, and v3 span r3? why or why not? yes, the vectors v1 = (0,0, 4), v2 = (0, 4,12), and v3 = (6, 5, 8) do span ℝ3. this is because any 3 vectors in ℝ3 will span the entire space, meaning that any vector in ℝ3 can be written as a linear combination of these 3 vectors. Definition. let v be a vector space. vectors v1,v2, ,vk ∈ v are called linearly dependent if they satisfy a relation r1v1 r2v2 ··· rkvk = 0, where the coefficients r1, ,rk ∈ r are not all equal to zero. otherwise the vectors v1,v2, ,vk are called linearly independent. that is, if r1v1 r2v2 ··· rkvk = 0 =⇒ r1 = ··· = rk = 0.
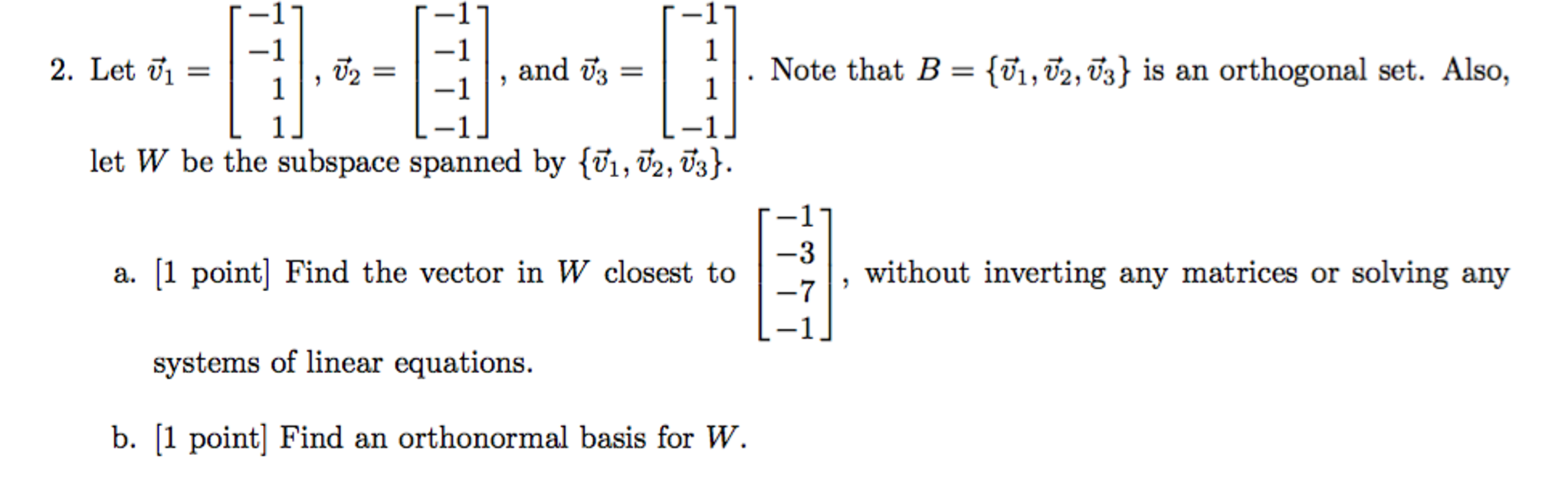
Solved Let V 1 1 1 1 1 V 2 1 1 1 1 And Chegg In r ^4 4, let v1 = (1, 1,2 , 1), v2 = (2 , 1,2, 0), v3 = (1 , 0, 2, 0), and v4 = (1 , 0, 3, 1). show that the set {v1 , v2, v3, v4} is affinely independent. in this problem, we are tasked to show that the set \ {\bold v 1, \bold v 2, \bold v 3, \bold v 4\} {v1,v2,v3,v4} is affinely independent. we are given the points. There are 4 steps to solve this one. consider a matrix a = [1 2 3 4 0 − 1 − 1 − 1 2 − 1 1 3] whose columns are vectors v 1, v 2, v 3, v 4 written as column vectors. (a) hence col (a)=column spac not the question you’re looking for? post any question and get expert help quickly. Let v 1 = 0 0 −4, v 2 = 0 −4 12, and v 3 = 6 −5 −8. do v1, v2, and v3 span r3? why or why not? yes, the vectors v1 = (0,0, 4), v2 = (0, 4,12), and v3 = (6, 5, 8) do span ℝ3. this is because any 3 vectors in ℝ3 will span the entire space, meaning that any vector in ℝ3 can be written as a linear combination of these 3 vectors. Definition. let v be a vector space. vectors v1,v2, ,vk ∈ v are called linearly dependent if they satisfy a relation r1v1 r2v2 ··· rkvk = 0, where the coefficients r1, ,rk ∈ r are not all equal to zero. otherwise the vectors v1,v2, ,vk are called linearly independent. that is, if r1v1 r2v2 ··· rkvk = 0 =⇒ r1 = ··· = rk = 0.
