
7 2 Integral Calculus 02 Solutions Pdf Integral Calculus Solutions must be written neatly on test (when applicable) and answers easy to find. evaluate the following integrals. (a) was this document helpful?. Math 2300: calculus ii project: the harmonic series, the integral test 1.let’s start with the sequence a n = 1 n. does this sequence converge or diverge? explain. solution: to determine if a sequence converges, we just take a limit: lim n!1 1 n = 0. the sequence converges to 0. 2.now consider this in nite series (called the harmonic series.
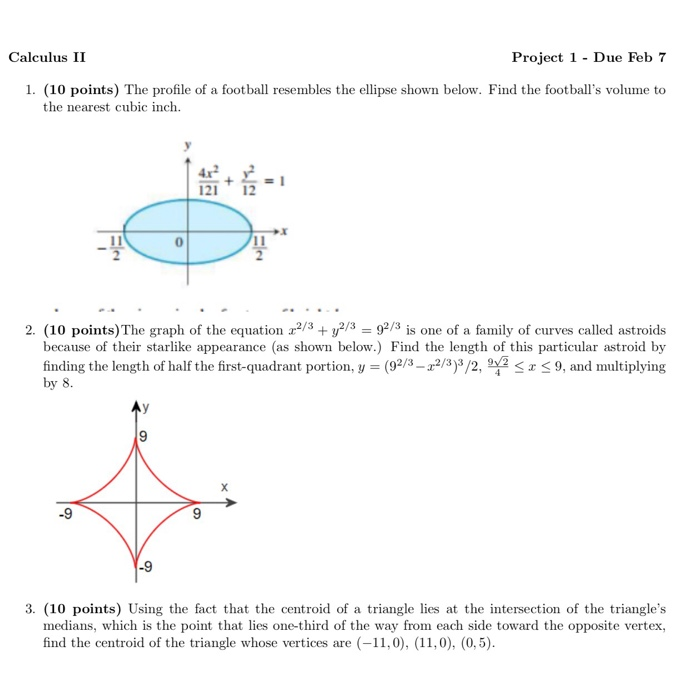
Solved Calculus Ii Project 1 Due Feb 7 1 10 Points The Chegg Laguardia community college mat 202 8 calculus ii project 2: a special technique for integration student: de la cruz, luis professor: tao, chen π 4 from the previous result, we can conclude that when x the can also be any integer and both equations will be the same. n 1 2 3 10 f ( x sin n x sinn x cos n x cos n x sin n x cos n x f. 7.2 integral calculus 02 solutions free download as pdf file (.pdf), text file (.txt) or read online for free. Here are a set of practice problems for the integration techniques chapter of the calculus ii notes. if you’d like a pdf document containing the solutions the download tab above contains links to pdf’s containing the solutions for the full book, chapter and section. Project ii: in this project, students use integrals to find the area between the graphs of two or more functions and the volume of different solids of revolution about vertical horizontal axis. both the slicing and cylindrical shells methods will be applied.

Solution Calculus 2 Integral Calculus Module 11 Part 1 Solutions Here are a set of practice problems for the integration techniques chapter of the calculus ii notes. if you’d like a pdf document containing the solutions the download tab above contains links to pdf’s containing the solutions for the full book, chapter and section. Project ii: in this project, students use integrals to find the area between the graphs of two or more functions and the volume of different solids of revolution about vertical horizontal axis. both the slicing and cylindrical shells methods will be applied. Introduction (problem statement) using a computer algebra system to find solutions to the following family of indefinite trigonometric integrals. 1. ∫ sin x cos 2x dx 2. ∫ sin 3x cos 7x dx 3. ∫ sin 8x cos 3x dx looking at the pattern of the output, guess the general formula and then prove it by checking the guess with a cas. (a) use a computer algebra system to evaluate the following integrals. dx 1x 5) x 2)(x 5) 2 (b) based on the pattern of your responses in part (a), guess the value of the integral (x kx 6 ifa . what if a b? (e) check your guess by asking your cas to evaluate the integral in part (b). Integration techniques – in this chapter we will look at several integration techniques including integration by parts, integrals involving trig functions, trig substitutions and partial fractions. we will also look at improper integrals including using the comparison test for convergence divergence of improper integrals. Puting riemann sums using xi = (xi−1 xi) 2 = midpoint of each interval as sample point. this yields the following approximation for the value of a definite integral: z b a f(x)dx ≈ xn i=1 f(xi)∆x = ∆x[f(x1) f(x2) ··· f(xn)]. example:usethemidpointrulewithn = 5toapproximate r1 0 x2 x. answer: the subintervals are [0,0.2], [0.2,0.4.
