
Calculus Iii Surface Integrals Of Vector Fields Pdf 1 surface integrals of vector fields hw problems evaluate ∬ 𝐹⃗∙𝑑𝑆⃗ 𝑆 using an upward outward pointing normal to the surface. 1. 𝐹⃗( , , )= ⃗−2 ⃗ 𝑘⃗⃗, 𝑆 is the plane =1, where r0, r0, r0. 2. Problem 2: calculate the surface integral of the vector field f = (yz, xz, xy) over the surface of the plane x y z = 1 in the first octant. solution: parameterize the surface: x = x, y = y, z = 1 x y; normal vector \mathbf{n} = \frac{\nabla(x y z 1)}{|\nabla(x y z 1)|} = \frac{(1, 1, 1)}{\sqrt{3}}.

Solution Surface Integrals Of Vector Fields Calculus 1 Exam Problems Vector calculus solutions to sample final examination #1 1. let f(x;y)=exysin(x y). (a) in what direction, starting at (0;ˇ=2), is fchanging the fastest? (b) in what directions starting at (0;ˇ=2) is fchanging at 50% of its maximum rate? (c) let c(t) be a flow line of f = rfwith c(0) = (0;ˇ=2). calculate d dt [f(c(t))] t=0: solution. This is the formula you want to use to compute surface integrals of vector fields. problem 1. evaluate. where ~f (x; y; z) = xy~i yz~j zx~k and s is the part of the paraboloid z = 4 that lies above the square in the xy plane such that 0 x 1 and 0 y has upward orientation. Example evaluate the integral a 1 1 x2 ds where s is the unit normal over the area a and a is the square 0 ≤ x ≤ 1, 0 ≤ y ≤ 1, z =0. solution in this integral, s becomes k dx dy i.e. the unit normal times the surface element. thus the integral is 1 y=0 1 x=0 k 1 x2 dx dy = 1 y=0 ktan−1 x 1 0 dy = 1 y=0 k(π 4 −0) 1 0 dy = π 4 k 1 y. Surface integrals, stokes’ theorem and gauss’ theorem used to be in the math240 syllabus until last year, so we will look at some of the questions from those old exams for practice.

Solution Surface Integrals Of Vector Fields Calculus 1 Exam Problems Example evaluate the integral a 1 1 x2 ds where s is the unit normal over the area a and a is the square 0 ≤ x ≤ 1, 0 ≤ y ≤ 1, z =0. solution in this integral, s becomes k dx dy i.e. the unit normal times the surface element. thus the integral is 1 y=0 1 x=0 k 1 x2 dx dy = 1 y=0 ktan−1 x 1 0 dy = 1 y=0 k(π 4 −0) 1 0 dy = π 4 k 1 y. Surface integrals, stokes’ theorem and gauss’ theorem used to be in the math240 syllabus until last year, so we will look at some of the questions from those old exams for practice. Here is a set of practice problems to accompany the surface integrals of vector fields section of the surface integrals chapter of the notes for paul dawkins calculus iii course at lamar university. Your matched tutor provides personalized help according to your question details. payment is made only after you have completed your 1 on 1 session and are satisfied with your session. Our expert help has broken down your problem into an easy to learn solution you can count on. see answer see answer see answer done loading question: (30 points) evaluate the surface integral of the vector field f=[x,y,z] over the surface s given by theleft hemisphere of the sphere x2 y2 z2=32 where x≤0, and the. First, let's substitute the parametric surface into the vector field: f (x, z) = − x 2, x, 1 . next, we take the dot product of f and n: f (x, z) ⋅ n = − x 2, x, 1 ⋅ − 2 x, 1, 0 = 2 x 3 x. finally, we can compute the flux integral as follows: ∬ s f ⋅ ds = ∫ 0 4 ∫ 0 1 (2 x 3 x) d z d x. 5. evaluate the integral.

Solution Surface Integrals Of Vector Fields Calculus 1 Exam Problems Here is a set of practice problems to accompany the surface integrals of vector fields section of the surface integrals chapter of the notes for paul dawkins calculus iii course at lamar university. Your matched tutor provides personalized help according to your question details. payment is made only after you have completed your 1 on 1 session and are satisfied with your session. Our expert help has broken down your problem into an easy to learn solution you can count on. see answer see answer see answer done loading question: (30 points) evaluate the surface integral of the vector field f=[x,y,z] over the surface s given by theleft hemisphere of the sphere x2 y2 z2=32 where x≤0, and the. First, let's substitute the parametric surface into the vector field: f (x, z) = − x 2, x, 1 . next, we take the dot product of f and n: f (x, z) ⋅ n = − x 2, x, 1 ⋅ − 2 x, 1, 0 = 2 x 3 x. finally, we can compute the flux integral as follows: ∬ s f ⋅ ds = ∫ 0 4 ∫ 0 1 (2 x 3 x) d z d x. 5. evaluate the integral.

Solution Conservative Vector Fields Line Integrals Calculus 1 Exam Our expert help has broken down your problem into an easy to learn solution you can count on. see answer see answer see answer done loading question: (30 points) evaluate the surface integral of the vector field f=[x,y,z] over the surface s given by theleft hemisphere of the sphere x2 y2 z2=32 where x≤0, and the. First, let's substitute the parametric surface into the vector field: f (x, z) = − x 2, x, 1 . next, we take the dot product of f and n: f (x, z) ⋅ n = − x 2, x, 1 ⋅ − 2 x, 1, 0 = 2 x 3 x. finally, we can compute the flux integral as follows: ∬ s f ⋅ ds = ∫ 0 4 ∫ 0 1 (2 x 3 x) d z d x. 5. evaluate the integral.
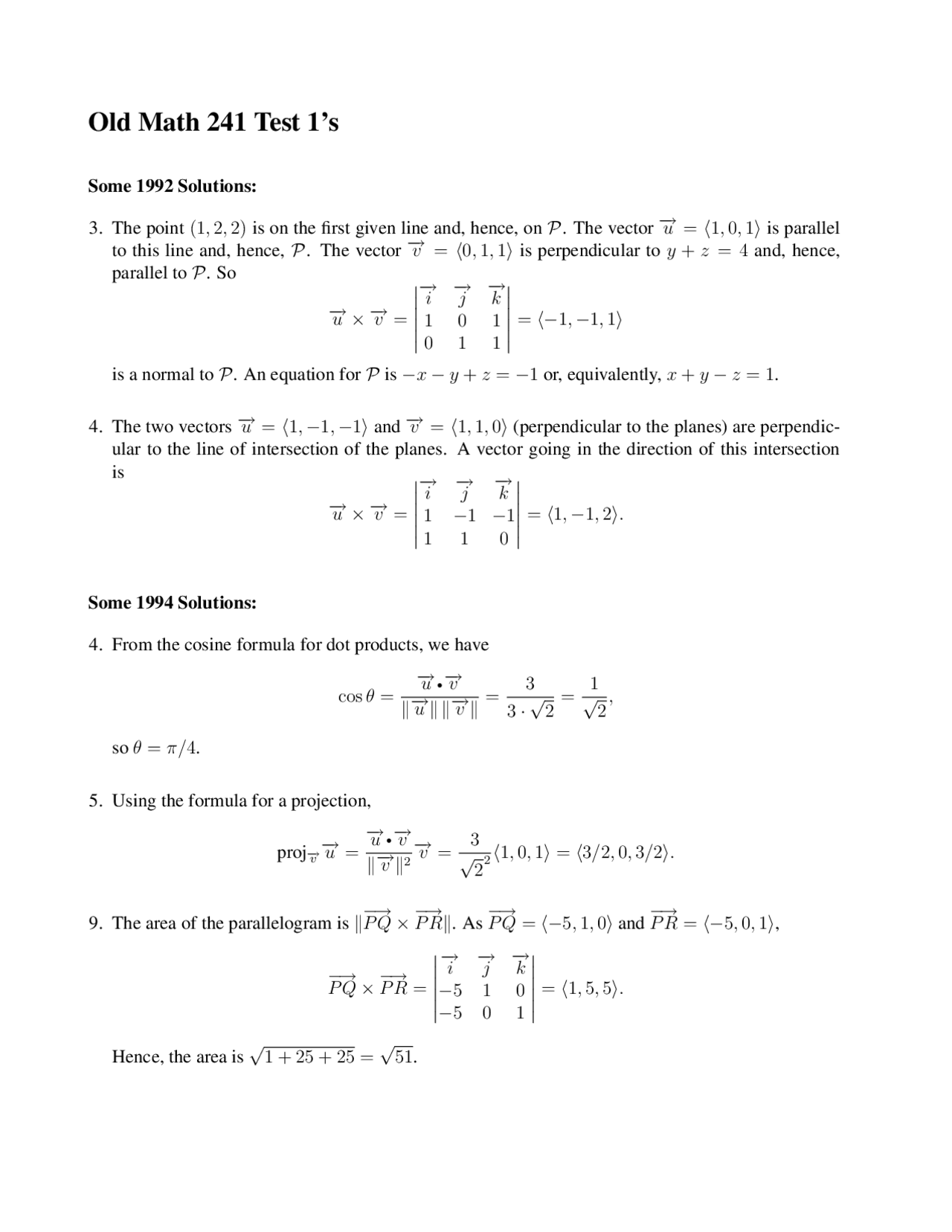
Solution To Exam 1 Vector Calculus Math 241 Docsity
