
Solved 1 A Let U 1 2 3 5 0 V 0 4 1 1 2 And Chegg 1: let u = (2, 1, − 1, 0), v = (3, 4, − 5, 0) and w = (− 4, − 1, 1, 0). find a vector q in r 4 which is orthogonal to u, v and w. there are infinitely many such vectors, but determine the collection of all such vectors. 2: let u = (cos θ sin ϕ, sin θ sin ϕ, 0), w = (r cos θ cos ϕ, r sin θ cos ϕ, − r sin ϕ) compute u ∘ v and. (b) f(2;1;0);(1;1;1);(4;3;2)g solution: denote u = (2;1;0), v = (1;1;1), w = (4;3;2). it is easy to see that w = u 2v and u;v are linearly independent (not parallel to each other), hence the set of all linear combination of u;v;w is two dimensional, i.e. a plane. (c) f(2; 3;1);( 4;6; 2);( 10;15; 5)g solution: denote u = (2; 3;1), v = ( 4;6; 2.
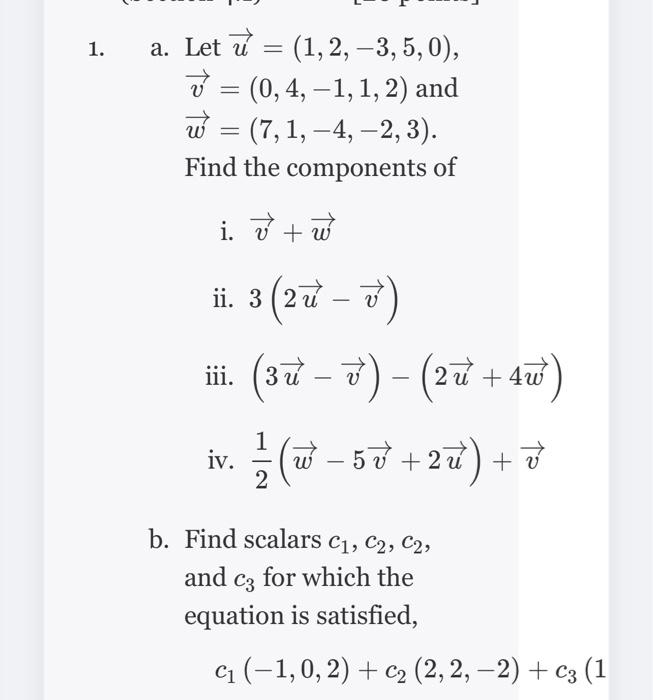
Solved A Let U 1 2 3 5 0 V 0 4 1 1 2 And Chegg Let u = (2, 1, 5, 0), v = (4, 3, 1, 1), and w = ( 6, 2, 0, 3) be vectors in r^4. find x using each equation. a. x = 2u – (v 3w) b. 3 (x w) = 2u – v x. the "step by step explanation" refers to a detailed and sequential breakdown of the solution or reasoning behind the answer. Let u = (2, − 1, 5, 0), v = (4, 3, 1, − 1), and w = (− 6, 2, 0, 3) be vectors in r 4. solve x in each of the following cases. 1. denote the vectors by ~u = (5,−2,4), ~v = (2,−3,5), and w~ = (4,5 −7). consider a~u b~v cw~ =~0. recall that the vectors are linearly independent if the only solution of the previous equation is a = b = c = 0, and linearly dependent otherwise. the equation can be written as a 5 −2 4 b 2 −3 5 c 4 5 −7 = 0 0 0 , or in matrix. Solution: let v = au 1 bu 2 cu 3. we need to solve for a,b,c. writing the equation explicitly, we have (2,5,−4,0) = a(1,3,2,1) b(2,−2,−5,4) c(2,−1,3,6). therefore (2,5,−4,0) = (a 2b 2c,3a−2b−c,2a−5b 3c,a 4b 6c) equating entry wise, we have system of linear equation a 2b 2c = 2 3a −2b −c = 5 2a −5b 3c = −4 a 4b 6c = 0.

Solved 1 Let U 2 1 1 0 V 3 4 5 0 And W 4 1 1 0 Chegg 1. denote the vectors by ~u = (5,−2,4), ~v = (2,−3,5), and w~ = (4,5 −7). consider a~u b~v cw~ =~0. recall that the vectors are linearly independent if the only solution of the previous equation is a = b = c = 0, and linearly dependent otherwise. the equation can be written as a 5 −2 4 b 2 −3 5 c 4 5 −7 = 0 0 0 , or in matrix. Solution: let v = au 1 bu 2 cu 3. we need to solve for a,b,c. writing the equation explicitly, we have (2,5,−4,0) = a(1,3,2,1) b(2,−2,−5,4) c(2,−1,3,6). therefore (2,5,−4,0) = (a 2b 2c,3a−2b−c,2a−5b 3c,a 4b 6c) equating entry wise, we have system of linear equation a 2b 2c = 2 3a −2b −c = 5 2a −5b 3c = −4 a 4b 6c = 0. Let $u = <2, 0>, v = <0, 5>,$ and $w = < 4, 3>$. find the vector $x$ that satisfies $4u v x=10x w$. in this case, vector $x$ is?. Question: exercise 1 let u = (2, 1,5,0), v = (4, 3, 1, 1), and w = ( 6,2,0, 3) be vectors in r4. solve x in each of the following cases. (a) x= 3u (v 2w) (b)2(x w) = 3u v x exercise 2 determine whether the following sets of vectors in r3 is l.i. or l.d s1 = { v1, v2, v3} = {(1, 2, 3), (0, 2, 4), ( 2, 0, 1)} s2 = { v1, v2, v3} = {0, 1. Let $u = (2, 3, 1)$, $v = (1, 3, 0)$, and $w = (2, 3, 3)$. since $(1 2)u (2 3)v (1 6)w = (0, 0, 0)$ can we conclude that the set $\{u, v, w\}$ is linearly dependent over $\mathbb{z} 7$?. There are 3 steps to solve this one. 1. let u= ( 2,0,4), v= (3, 1,6), and w= (2, 5, 5). compute (a) 3v 2u (b) ||u v w| (c) the distance between 3u and v sw (d) proju (e) u (vxw)) (1) ( 5v w)* ( (u.v)w) answer: (a) 3v 2u = (13. 3. 10) (b) ||u v wil = 70 (c) 774 (d) proju (2.

Solved Let U 2 7 1 V 3 0 4 W 0 5 8 Find A Chegg Let $u = <2, 0>, v = <0, 5>,$ and $w = < 4, 3>$. find the vector $x$ that satisfies $4u v x=10x w$. in this case, vector $x$ is?. Question: exercise 1 let u = (2, 1,5,0), v = (4, 3, 1, 1), and w = ( 6,2,0, 3) be vectors in r4. solve x in each of the following cases. (a) x= 3u (v 2w) (b)2(x w) = 3u v x exercise 2 determine whether the following sets of vectors in r3 is l.i. or l.d s1 = { v1, v2, v3} = {(1, 2, 3), (0, 2, 4), ( 2, 0, 1)} s2 = { v1, v2, v3} = {0, 1. Let $u = (2, 3, 1)$, $v = (1, 3, 0)$, and $w = (2, 3, 3)$. since $(1 2)u (2 3)v (1 6)w = (0, 0, 0)$ can we conclude that the set $\{u, v, w\}$ is linearly dependent over $\mathbb{z} 7$?. There are 3 steps to solve this one. 1. let u= ( 2,0,4), v= (3, 1,6), and w= (2, 5, 5). compute (a) 3v 2u (b) ||u v w| (c) the distance between 3u and v sw (d) proju (e) u (vxw)) (1) ( 5v w)* ( (u.v)w) answer: (a) 3v 2u = (13. 3. 10) (b) ||u v wil = 70 (c) 774 (d) proju (2.

Solved 9 Let U 4 1 V 0 5 And W 3 3 Find Chegg Let $u = (2, 3, 1)$, $v = (1, 3, 0)$, and $w = (2, 3, 3)$. since $(1 2)u (2 3)v (1 6)w = (0, 0, 0)$ can we conclude that the set $\{u, v, w\}$ is linearly dependent over $\mathbb{z} 7$?. There are 3 steps to solve this one. 1. let u= ( 2,0,4), v= (3, 1,6), and w= (2, 5, 5). compute (a) 3v 2u (b) ||u v w| (c) the distance between 3u and v sw (d) proju (e) u (vxw)) (1) ( 5v w)* ( (u.v)w) answer: (a) 3v 2u = (13. 3. 10) (b) ||u v wil = 70 (c) 774 (d) proju (2.
