
Solved 10 Let A Bв Z A Prove That If A2в јb2 Then Aв јb B Chegg (# 4.12) let a,b ∈ z. prove that if a2 2b2 ≡ 0(mod 3), then either a and b are congruent to 0 modulo 3 or neither is congruent to 0 modulo 3. solution. note that this may be divided into cases. every integer is congruent to either 0,1, or 2 modulo 3. therefore we have either a ≡ 0(mod 3), a ≡ 1(mod 3),or a ≡ 2(mod 3). likewise. There are 2 steps to solve this one. a^2 ≡1 (mod 5) . 4.15. let a,b,c,n∈z, where n≥2. prove that if a≡b(modn) and a≡c(modn), then b≡ c(modn). 4.16. let a,b∈z. prove that a2 2b2 ≡0(mod3) if and only if either a and b are both congruent to 0 modulo 3 or neither is congruent to 0 modulo 3 . 17.
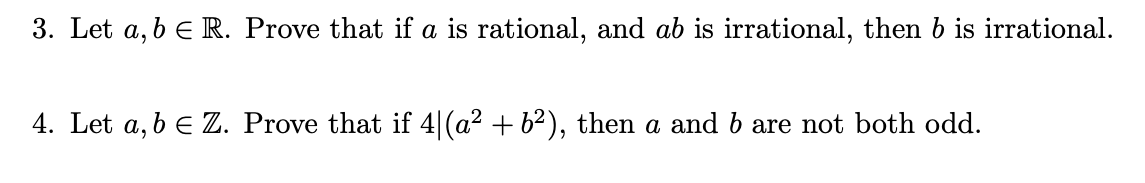
Solved 3 Let A Bв R Prove That If A Is Rational And Ab Is Chegg Q 8: suppose a,b, and c 2z , if a2 b2 = c2, then a or b is even. proof: proof by contradictiction suppose a2 b2 = c2 and a,b are odd. let a=2m 1 and b=2n 1 since they are both odd, m and n are integers. a2 b2 = (2m 1)(2n 1) i.e. a2 b2 = 4(m2 n2 n m) 2. i.e. a2 b2 = 4x 2, x is an integer. now c2 2 = 4x therefore, c is even let c = 2r. Let $a,b \in \mathbb z$. prove that if $3 \mid (a 2b)$ then $3 \mid (2a b)$ this is how i solved this: $3m = a 2b \iff a = 3m 2b$ $2a b = 2(3m 2b) b = 6m 3b = 3(2m b)$ and now, my solution seems to work. however, when instead of solving for $a$, i solve for $b$, then i get this: $2a b = 3 \frac{a m}{2}$. Consider the prime factorization of both b 2 b^2 b 2 and k a 2 ka^2 k a 2 according to the fundamental theorem of arithmetic, and it becomes obvious that the no. of positive divisors of b 2 b^2 b 2 is clearly odd, while that of k a 2 ka^2 k a 2 is only so iff all the powers of primes in the prime factorization of k k k is even, i.e. k k k is a. For this question, we need to realize that if a \b = ;, then a b = fa 2a : a =2bg= a. then, if we rewrite the question, we see that we want to prove or disprove the statement, if a and b are sets and a\b = ;, then p(a) p (b) p(a). but this statement is true. so we need to prove it. proof: let x 2p(a) p (b). then we see x 2p(a) but x =2p(b). we.

Solved 13 Let A B Z Prove That If A 0 Mod 5 And B Chegg Consider the prime factorization of both b 2 b^2 b 2 and k a 2 ka^2 k a 2 according to the fundamental theorem of arithmetic, and it becomes obvious that the no. of positive divisors of b 2 b^2 b 2 is clearly odd, while that of k a 2 ka^2 k a 2 is only so iff all the powers of primes in the prime factorization of k k k is even, i.e. k k k is a. For this question, we need to realize that if a \b = ;, then a b = fa 2a : a =2bg= a. then, if we rewrite the question, we see that we want to prove or disprove the statement, if a and b are sets and a\b = ;, then p(a) p (b) p(a). but this statement is true. so we need to prove it. proof: let x 2p(a) p (b). then we see x 2p(a) but x =2p(b). we. Determine whether or not each of the statements is true or false. prove your assertion. 1. suppose a; b; and c are sets. if a b, then a c b c. solution: this statement is true. proof. it is straightforward to show that if any of a; b; or c is the empty set, then the statement is true. so, let a; b; and c be nonempty sets such that a b. then. To prove a ∩ (b ∪ c) = (a ∩ b) ∪ (a ∩ c), first note that the statement involves three sets, a, b, and c, so there are 23 = 8 possibilities for the membership of an element in the sets. since each entry in column 7 is the same as the corresponding entry in column 8, we have shown that a ∩ (b ∪ c) = (a ∩ b) ∪ (a ∩ c) for any sets a, b, and c. Theorem: if a ⊆ b ∪ c, b ⊆ d, and c ⊆ e, then a ⊆ d ∪ e. proof: consider any sets a, b, c, d, and e where a ⊆ b ∪ c, b ⊆ d, and c ⊆ e. we will prove that a ⊆ d ∪ e. to do so, pick an arbitrary x ∈ a. we will prove that x ∈ d ∪ e. since we know x ∈ a and a ⊆ b ∪ c, we see that x ∈ b ∪ c. [ the rest of the. Proposition: for every a;b 2z, ab is even if and only if a is even or b is even. proof: let a and b be integers. first, suppose a is even or b is even. wlog, let a be even, so a = 2x for some x 2z. then ab = 2(xb), so ab is even. we conclude that, if a or b is even, then ab is even. second, suppose that both a and b are odd.

Solved Activity 1 6e Prove That в A B в Aв B Let A Bв Z Chegg Determine whether or not each of the statements is true or false. prove your assertion. 1. suppose a; b; and c are sets. if a b, then a c b c. solution: this statement is true. proof. it is straightforward to show that if any of a; b; or c is the empty set, then the statement is true. so, let a; b; and c be nonempty sets such that a b. then. To prove a ∩ (b ∪ c) = (a ∩ b) ∪ (a ∩ c), first note that the statement involves three sets, a, b, and c, so there are 23 = 8 possibilities for the membership of an element in the sets. since each entry in column 7 is the same as the corresponding entry in column 8, we have shown that a ∩ (b ∪ c) = (a ∩ b) ∪ (a ∩ c) for any sets a, b, and c. Theorem: if a ⊆ b ∪ c, b ⊆ d, and c ⊆ e, then a ⊆ d ∪ e. proof: consider any sets a, b, c, d, and e where a ⊆ b ∪ c, b ⊆ d, and c ⊆ e. we will prove that a ⊆ d ∪ e. to do so, pick an arbitrary x ∈ a. we will prove that x ∈ d ∪ e. since we know x ∈ a and a ⊆ b ∪ c, we see that x ∈ b ∪ c. [ the rest of the. Proposition: for every a;b 2z, ab is even if and only if a is even or b is even. proof: let a and b be integers. first, suppose a is even or b is even. wlog, let a be even, so a = 2x for some x 2z. then ab = 2(xb), so ab is even. we conclude that, if a or b is even, then ab is even. second, suppose that both a and b are odd.

Solved Let A B Cв Z Prove That If Aв јb And Aв ј B C Then Chegg Theorem: if a ⊆ b ∪ c, b ⊆ d, and c ⊆ e, then a ⊆ d ∪ e. proof: consider any sets a, b, c, d, and e where a ⊆ b ∪ c, b ⊆ d, and c ⊆ e. we will prove that a ⊆ d ∪ e. to do so, pick an arbitrary x ∈ a. we will prove that x ∈ d ∪ e. since we know x ∈ a and a ⊆ b ∪ c, we see that x ∈ b ∪ c. [ the rest of the. Proposition: for every a;b 2z, ab is even if and only if a is even or b is even. proof: let a and b be integers. first, suppose a is even or b is even. wlog, let a be even, so a = 2x for some x 2z. then ab = 2(xb), so ab is even. we conclude that, if a or b is even, then ab is even. second, suppose that both a and b are odd.
