
Solved 16 By Using Appropriate Identities Where Required Chegg Here’s the best way to solve it. 16. by using appropriate identities, where required, determine which of the following sets of vectors in f ( 00,00) are lin early dependent. nobraqob yhts (a) 6, 3 sinº x, 2 cosx (b) x, cos x islom (c) 1, sin x, sin 2x (d) cos 2x, sinºx, cos” x (e) (3 x)2, x2 6x, 5 (f) 0, cos' ax, sinº 37x. By using appropriate identities, where required, determine which of the following sets of vectors in are linearly dependent. (a) (b) (c) (d) (e) (f) 21. the functions and are linearly independent in because neither function is a scalar multiple of the other. confirm the linear independence using wroński's test. answer: for some x. 22.
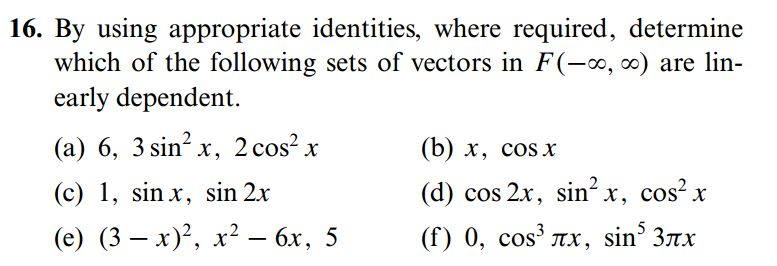
Solved 16 By Using Appropriate Identities Where Required Chegg By using appropriate identities, where required, determine if the following set of vectors in f ( infinity, infinity) is linearly dependent: 6, 3(sin2(x)), 2(cos2(x)) 6, 3 (sin 2 (x)), 2 (cos 2 (x)). Professor dean provides chegg with list of questions to flag as cheating. chegg performs automated search query of all recently asked questions to look for matches. chegg finds op's question as a match, reports op to the dean and gives him an email to rub in how much he is fucked. Using \(\cos 2x = \cos^2 x \sin^2 x\) and \(\sin^2 x \cos^2 x = 1\), substituting these identities reveals dependencies. specifically: \(\cos 2x \sin^2 x \cos^2 x = 0\), confirming dependence. Let's factorize each expression step by step using appropriate algebraic identities. here's how you can do it: (i) 4x2 9y2 16z2 12xy − 24yz − 1. arrange the terms: first, look at the way terms are arranged to see if they can be grouped conveniently.

Solved By Using Appropriate Identities Where Required Chegg Using \(\cos 2x = \cos^2 x \sin^2 x\) and \(\sin^2 x \cos^2 x = 1\), substituting these identities reveals dependencies. specifically: \(\cos 2x \sin^2 x \cos^2 x = 0\), confirming dependence. Let's factorize each expression step by step using appropriate algebraic identities. here's how you can do it: (i) 4x2 9y2 16z2 12xy − 24yz − 1. arrange the terms: first, look at the way terms are arranged to see if they can be grouped conveniently. Now, we can express the third vector as a linear combination of the first two vectors: 2cos^2 x = 2 2sin^2 x = 2 (1 sin^2 x) = 2 2 (3 sin^2 x) 3 = 6 3 sin^2 x. therefore, these vectors are linearly dependent. (b) x, cos x, sin x, sin 2x we cannot express any of these vectors as a linear combination of the others. By using appropriate identities, where required, determine which of the following sets of vectors in $f ( \infty, \infty)$ are linearly dependent. a. $6,3 \sin ^ {2} x, 2 \cos ^ {2} x$ b. $x, \cos x$ c. $1, \sin x, \sin 2 x$ d. $\cos 2 x, \sin ^ {2} x, \cos ^ {2} x$ e. $ (3 x)^ {2}, x^ {2} 6 x, 5$ f. $0, \cos ^ {3} \pi x, \sin ^ {5} 3 \pi x$ 3. There are 2 steps to solve this one. we need to check whether these vectors are linearly dependent. let's apply wronskian method. 16. by using appropriate identities, where required, determine which of the following sets of vectors in f ( o,o) are lin early dependent. Find step by step linear algebra solutions and your answer to the following textbook question: by using appropriate identities, where required, determine which of the following sets of vectors in f ( oo, oo) are linearly dependent.

Solved By Using Appropriate Identities Where Required Chegg Now, we can express the third vector as a linear combination of the first two vectors: 2cos^2 x = 2 2sin^2 x = 2 (1 sin^2 x) = 2 2 (3 sin^2 x) 3 = 6 3 sin^2 x. therefore, these vectors are linearly dependent. (b) x, cos x, sin x, sin 2x we cannot express any of these vectors as a linear combination of the others. By using appropriate identities, where required, determine which of the following sets of vectors in $f ( \infty, \infty)$ are linearly dependent. a. $6,3 \sin ^ {2} x, 2 \cos ^ {2} x$ b. $x, \cos x$ c. $1, \sin x, \sin 2 x$ d. $\cos 2 x, \sin ^ {2} x, \cos ^ {2} x$ e. $ (3 x)^ {2}, x^ {2} 6 x, 5$ f. $0, \cos ^ {3} \pi x, \sin ^ {5} 3 \pi x$ 3. There are 2 steps to solve this one. we need to check whether these vectors are linearly dependent. let's apply wronskian method. 16. by using appropriate identities, where required, determine which of the following sets of vectors in f ( o,o) are lin early dependent. Find step by step linear algebra solutions and your answer to the following textbook question: by using appropriate identities, where required, determine which of the following sets of vectors in f ( oo, oo) are linearly dependent.
