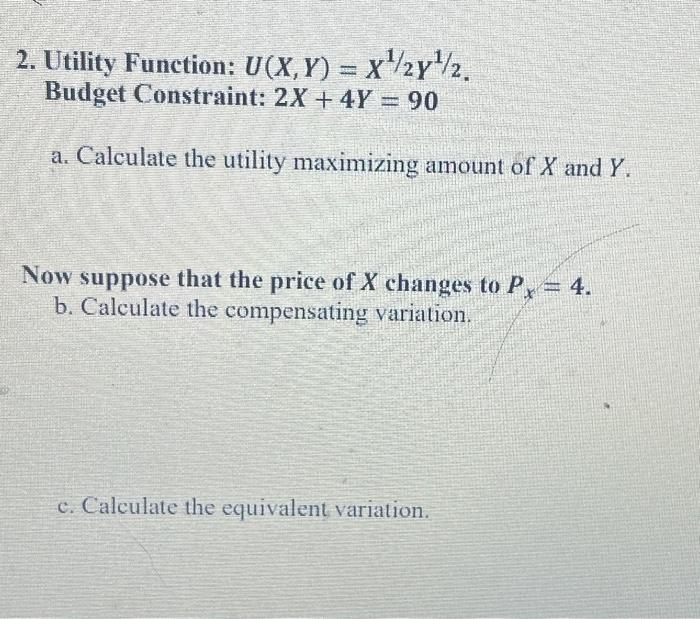
Solved 2 Utility Function U X Y X1 2y1 2 Budget Chegg Question: 2. utility function: u(x,y)=x1 2y1 2. budget constraint: 2x 4y=90 a. calculate the utility maximizing amount of x and y. now suppose that the price of x changes to px=4. b. calculate the compensating variation. c. calculate the equivalent variation. Substitute the expression of y into the utility function u(x,y). maximize the utility function which is now a function of only one of the good (good x in this case). the f.o.c yields the solution for good x. substitute the value of good x onto the budget constraint to get the solution for good y. x yy m p yx pp.

Solved 2 Utility Function U X Y X1 2y1 2 Budget Chegg U(x;y) = x y1 that is, that the cobb douglas is a special case of the ces function where ˆ = 0. hint: remember that limz!0 g(z) = elimz!0 lng(z). hint 2: you will need l’hôpital rule. solution: lim ˆ!0 u(x;y) = lim ˆ!0 ( x ˆ (1 )yˆ)1 = exp [lim ˆ!0 ln( xˆ (1 )yˆ) ˆ] = exp [lim ˆ!0 xˆ lnx (1 )yˆ lny xˆ (1 )yˆ] = exp [ lnx (1. Suppose a consumer’s preferences for two goods can be represented by the cobb douglas utility function u(x, y) = a x α y β, where a, α, and β are positive constants. a. what is mrsx, y ? b. is mrsx, y diminishing, constant, or increasing as the consumer substitutes x. for y along an indifference curve?. Utility function: u(x,y)=x 1 2 y 1 2. budget constraint: x 2y=12. if the price of good y changes to py=3, what is the compensating variation? (round to the nearest one tenth). Problem 1. utility maximization. (75 points) in this exercise, we consider a standard utility maximization problem with an unusual (for us) income. the utility function is ( )= log( ) (1− )log( ) this function is well defined for 0 and for 0 from now on, assume 0 and 0 unless otherwise stated.

Solved Consider The Utility Function U X Y X1 2y1 2 A Chegg Utility function: u(x,y)=x 1 2 y 1 2. budget constraint: x 2y=12. if the price of good y changes to py=3, what is the compensating variation? (round to the nearest one tenth). Problem 1. utility maximization. (75 points) in this exercise, we consider a standard utility maximization problem with an unusual (for us) income. the utility function is ( )= log( ) (1− )log( ) this function is well defined for 0 and for 0 from now on, assume 0 and 0 unless otherwise stated. A utility function of the form u(x 1,x 2) = f(x 1) x 2 is linear in just x 2 and is called quasi linear. e.g.u(x. u(x 1,x 2) = 2x 1 1 2 x 2. To find the equivalent variation, first calculate the initial consumption bundle using lagrange multipliers to maximize utility given the original budget constraint. to find the equivalent variation when the price of good y changes, we need to determine how much the. Exercise 2. 1 3use the utility function u(x 1,x 2)= x 1 1 2x 2 and the budget constraint m=p 1 x 1 p 2 x 2 to calculate the walrasian demand, the indirect utility function, the hicksian demand, and the expenditure function. solution. the lagrangian for the utility maximization problem is 1 2 1 3 ( , ) ( ),x x x p x p x moo 1 2 1 1 2 2 taking. Question: consider a cobb douglas utility function u(x,y) = x1 2y1 2. suppose i is income and prices for x and y are px and py respectively. a) find the demand functions and show that it is homogeneous of degree zero. b) find the indirect utility function. c) show that marshallian demand functions can be recover from indirect.

Solved Q1 Consider The Utility Function U X Y X1 2y1 2 Chegg A utility function of the form u(x 1,x 2) = f(x 1) x 2 is linear in just x 2 and is called quasi linear. e.g.u(x. u(x 1,x 2) = 2x 1 1 2 x 2. To find the equivalent variation, first calculate the initial consumption bundle using lagrange multipliers to maximize utility given the original budget constraint. to find the equivalent variation when the price of good y changes, we need to determine how much the. Exercise 2. 1 3use the utility function u(x 1,x 2)= x 1 1 2x 2 and the budget constraint m=p 1 x 1 p 2 x 2 to calculate the walrasian demand, the indirect utility function, the hicksian demand, and the expenditure function. solution. the lagrangian for the utility maximization problem is 1 2 1 3 ( , ) ( ),x x x p x p x moo 1 2 1 1 2 2 taking. Question: consider a cobb douglas utility function u(x,y) = x1 2y1 2. suppose i is income and prices for x and y are px and py respectively. a) find the demand functions and show that it is homogeneous of degree zero. b) find the indirect utility function. c) show that marshallian demand functions can be recover from indirect.
