Solved 3 Let A 1 2 3 4 5 6 And 1 2 3 4 5 6 P1 Chegg

Solved 3 Let A 1 2 3 4 5 6 And 1 2 3 4 5 6 P1 Chegg Xpgfs brings alive the x plane atmosphere combining metar reports and noaa weather data for the whole world. features: own metar interpretation engine. 8 layers of wind data with 0.5x0.5 degree resolution. 8 layers of turbulence data. Amd ryzen™ 7 9800x3d cpu nvidia gigabyte rtx 5090 64gb ram with a samsung odyssey g9 neo 49" curved monitor running a 5120 x 1440 resolution.
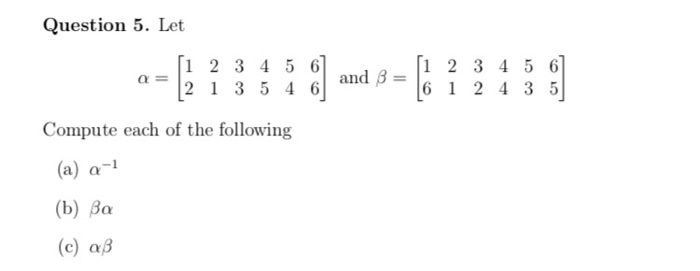
Solved Question 5 Let 1 2 3 4 5 6 2 1 3 5 4 6 And 3 1 2 3 Chegg Hello which weather plugin is the best looking one for xplane 11? iam looking for the most realistic weather plugin. Hi there, flying the 777 has been great, and the system depth and features are stunning. however, i have not been able to find much on weather radar usage in the fcom or cockpit manual, simply just an overview of certain returns and what each switch does. that said, i am confused as to what tilt. This is a flywithlua script that will prevent abrupt violent weather changes and will make the weather more accurate. it also includes cloud improvements and an option for fps improvement. Maybe i've missed something obvious, but is the weather radar non functioning in xp12?.

Solved Let A 1 1 3 4 5 6 Be A Set And Let B And C Be Chegg This is a flywithlua script that will prevent abrupt violent weather changes and will make the weather more accurate. it also includes cloud improvements and an option for fps improvement. Maybe i've missed something obvious, but is the weather radar non functioning in xp12?. Hi everyone! i don't remember seeing it mentioned anywhere; sorry if it was. are there any plans for updating the wx radar for the zibo mod in x plane 12? the current one doesn't seem realistic in x plane 12; i have even checked with a irl 737 pilot, and he confirmed. thanks for all your great wo. Hello everyone concerning the weather radar, is it simulated? i'm asking because i can't get it to work no matter which buttons i press. nothing happens. thank you for your answers. have a nice day. The plugin adds egpws terrain display feature (with peaks mode). now plugin work in two modes: integration into the navigation display (see the list of supported aircrafts) and overlay window (all other aircrafts) x plane version supported x plane version: x plane 10 32 64 bit x plane 11 64 bit x. Zhsi is a glass cockpit software suite for the zibo mod b737 800x.this program is free software: you can redistribute it and or modifyit under the terms of the gnu general public license as published bythe free software foundation, either version 3 of the license, or(at your option) any later ver.

2 Let A 2 3 4 B 4 5 6 C 5 6 7 And Let Chegg Hi everyone! i don't remember seeing it mentioned anywhere; sorry if it was. are there any plans for updating the wx radar for the zibo mod in x plane 12? the current one doesn't seem realistic in x plane 12; i have even checked with a irl 737 pilot, and he confirmed. thanks for all your great wo. Hello everyone concerning the weather radar, is it simulated? i'm asking because i can't get it to work no matter which buttons i press. nothing happens. thank you for your answers. have a nice day. The plugin adds egpws terrain display feature (with peaks mode). now plugin work in two modes: integration into the navigation display (see the list of supported aircrafts) and overlay window (all other aircrafts) x plane version supported x plane version: x plane 10 32 64 bit x plane 11 64 bit x. Zhsi is a glass cockpit software suite for the zibo mod b737 800x.this program is free software: you can redistribute it and or modifyit under the terms of the gnu general public license as published bythe free software foundation, either version 3 of the license, or(at your option) any later ver.
Comments are closed.