
Solved 41 Let V1 4 1 0 1 V2 0 1 4 1 And Chegg There are 2 steps to solve this one. we have v 1 ⋅ v 2 = (− 4) × 0 1 × 1 0 × (− 4) (− 1) × 1 = 0 1 0 − 1 = 0 . 41: let v1 =(−4,1,0,−1),v2 = (0,1,−4,1), and b=(1,8,−1,−7). let w be the subspace or r4 spanned by v1 and v2. find projw b using the euclidean inner product. Proj v1 b = (b · v1) (v1 · v1) * v1 proj v2 b = (b · v2) (v2 · v2) * v2 answer step 2: add the projections to find the projection of b onto w. proj w b = proj v1 b proj v2 b now, let's calculate the dot products and projections.
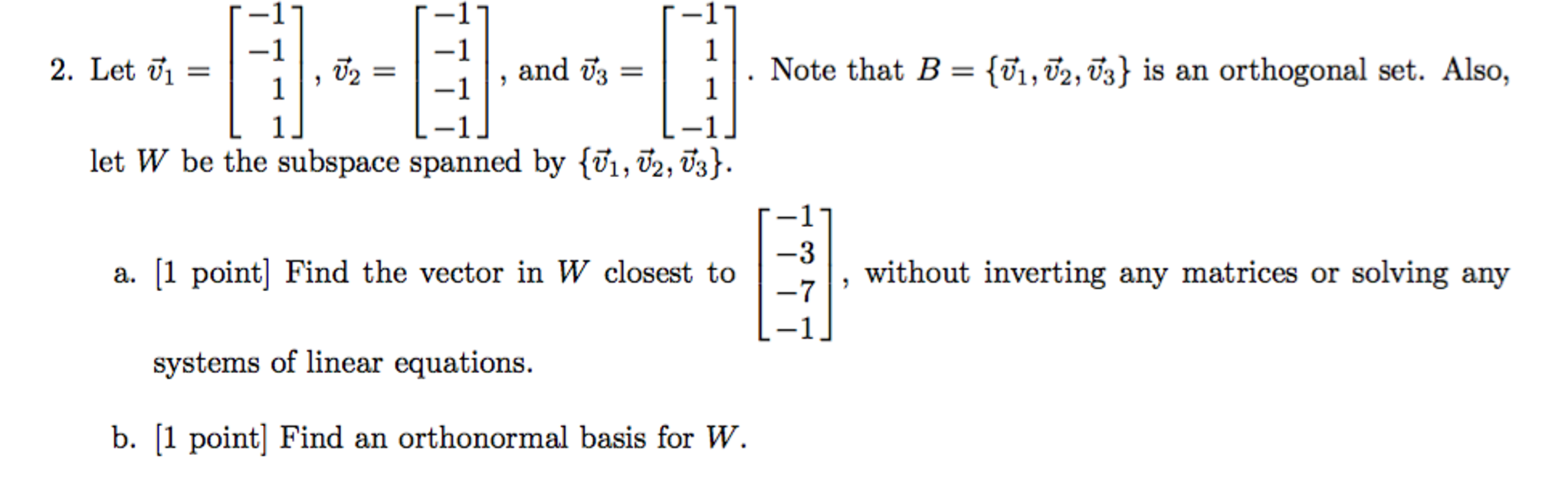
Solved Let V 1 1 1 1 1 V 2 1 1 1 1 And Chegg Our expert help has broken down your problem into an easy to learn solution you can count on. question: problem # 4: let v1= (4,1,0, 1),v2= (0,1,4,1), and b= (1,2, 1, 3). let w be the subspace or r4 spanned by v1 andv2. find projwb. problem # 4: let v 1 = (4, 1, 0, 1), v 2 = (0, 1, 4, 1), and b = (1, 2, 1, 3). Let t : r2 → r3 be defined by t(a1, a2) = (a1 − a2, a1, 2a1 a2). let β be the standard ordered basis for r2 and γ = { (1, 1, 0), (0, 1, 1), (2, 2, 3) }. compute [t]γ β. if α = { (1, 2), (2, 3) }, compute [t]γ α. solution: by definition, β = { (1, 0), (0, 1) }. 0 1 . 3 . 2.2.5. let = α 1 0 0 0. (a) define t : m2×2(f) → m2×2(f) by t(a) = at. Below i've provided a few methods of determining whether or not they are linearly independent. method #1. ⎡⎣⎢1 1 2 1 0 1 2 2 3⎤⎦⎥ [1 1 2 1 0 2 2 1 3] now use gauss jordan elimination to determine the rank of the matrix. if it is 3 3 (the number of columns), then the columns are linearly independent. method #2. construct the same matrix as above. Video answer: hello. we're going to use art for this exercise. with the standard definition of the inner product, is the cleaning even more considered? there are two w. one and w. two. w one is equal to one minus 1301 w two is equal to 4092. to.

Solved Problem 4 Let V1 4 1 0 1 V2 0 1 4 1 And Chegg Below i've provided a few methods of determining whether or not they are linearly independent. method #1. ⎡⎣⎢1 1 2 1 0 1 2 2 3⎤⎦⎥ [1 1 2 1 0 2 2 1 3] now use gauss jordan elimination to determine the rank of the matrix. if it is 3 3 (the number of columns), then the columns are linearly independent. method #2. construct the same matrix as above. Video answer: hello. we're going to use art for this exercise. with the standard definition of the inner product, is the cleaning even more considered? there are two w. one and w. two. w one is equal to one minus 1301 w two is equal to 4092. to. Let m = 2, let v = r2, let v 1 = „1;0”;v 2 = „0;1”be a list of vectors in r2, and let w 1 = v 1 = „1;0”and w 2 = v 2 = „0;1”. suppose a 1;a 2 satisfy a 1v 1 a 2v 2 = „0;0”: then we have „0;0”= a 1v 1 a 2v 2 = a 1„1;0” a 2„0;1” = „a 1;0” „0;a 2” = „a 1;a 2”; from which we get a 1 = 0;a 2 = 0, and. Let x (t) and y (t) determine two quadratic bézier curves, with control points {p0, p1, p2} and {p2, p3, p4}, respectively. the curves are joined at p2 = x (1) = y (0). a. suppose the combined curve has g^1 continuity (at p2). what algebraic restriction does this condition impose on the control points?. There are 2 steps to solve this one. let v 1 = (− 4, 1, 0, − 1), v 2 = (0, 1, − 4, 1), and b = (1, 8, − 1, − 9). w be the subspace. r 4 spanned by v 1 and v 2. find p r o j w b. = 0 1 0 − 1 not the question you’re looking for? post any question and get expert help quickly. answer to let v1= ( 4,1,0, 1),v2= (0,1, 4,1), and b= (1,8, 1, 9). Problem. let v0 be a subspace of dimension k in rn. let x 1,x2, ,xk be a basis for v0. (i) find an orthogonal basis for v0. (ii) extend it to an orthogonal basis for rn. approach 1. extend x1, ,xk to a basis x1,x2, ,xn for rn. then apply the gram schmidt process to the extended basis. we shall obtain an orthogonal basis v1, ,vn for rn.

Problem 4 Let V1 4 1 0 1 V2 0 1 4 1 And Chegg Let m = 2, let v = r2, let v 1 = „1;0”;v 2 = „0;1”be a list of vectors in r2, and let w 1 = v 1 = „1;0”and w 2 = v 2 = „0;1”. suppose a 1;a 2 satisfy a 1v 1 a 2v 2 = „0;0”: then we have „0;0”= a 1v 1 a 2v 2 = a 1„1;0” a 2„0;1” = „a 1;0” „0;a 2” = „a 1;a 2”; from which we get a 1 = 0;a 2 = 0, and. Let x (t) and y (t) determine two quadratic bézier curves, with control points {p0, p1, p2} and {p2, p3, p4}, respectively. the curves are joined at p2 = x (1) = y (0). a. suppose the combined curve has g^1 continuity (at p2). what algebraic restriction does this condition impose on the control points?. There are 2 steps to solve this one. let v 1 = (− 4, 1, 0, − 1), v 2 = (0, 1, − 4, 1), and b = (1, 8, − 1, − 9). w be the subspace. r 4 spanned by v 1 and v 2. find p r o j w b. = 0 1 0 − 1 not the question you’re looking for? post any question and get expert help quickly. answer to let v1= ( 4,1,0, 1),v2= (0,1, 4,1), and b= (1,8, 1, 9). Problem. let v0 be a subspace of dimension k in rn. let x 1,x2, ,xk be a basis for v0. (i) find an orthogonal basis for v0. (ii) extend it to an orthogonal basis for rn. approach 1. extend x1, ,xk to a basis x1,x2, ,xn for rn. then apply the gram schmidt process to the extended basis. we shall obtain an orthogonal basis v1, ,vn for rn.

Solved 1 Let V1 1 1 1 1 V2 1 2 1 4 V3 Chegg There are 2 steps to solve this one. let v 1 = (− 4, 1, 0, − 1), v 2 = (0, 1, − 4, 1), and b = (1, 8, − 1, − 9). w be the subspace. r 4 spanned by v 1 and v 2. find p r o j w b. = 0 1 0 − 1 not the question you’re looking for? post any question and get expert help quickly. answer to let v1= ( 4,1,0, 1),v2= (0,1, 4,1), and b= (1,8, 1, 9). Problem. let v0 be a subspace of dimension k in rn. let x 1,x2, ,xk be a basis for v0. (i) find an orthogonal basis for v0. (ii) extend it to an orthogonal basis for rn. approach 1. extend x1, ,xk to a basis x1,x2, ,xn for rn. then apply the gram schmidt process to the extended basis. we shall obtain an orthogonal basis v1, ,vn for rn.

Solved Problem 4 Let V1 4 1 0 1 V2 0 1 4 1 And Chegg
