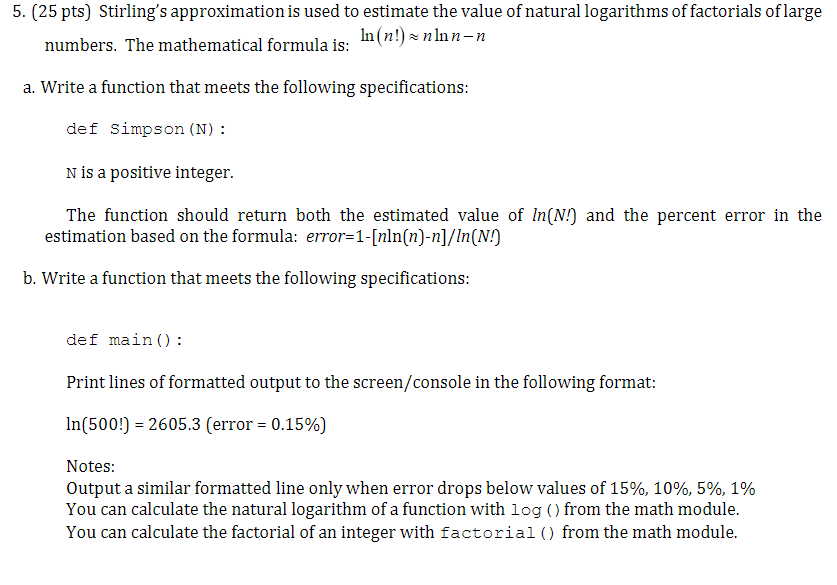
Solved 5 25 Pts Stirling S Approximation Is Used To Chegg Question: 5. (25 pts) stirling's approximation is used to estimate the value of natural logarithms of factorials of large numbers. the mathematical formula is. in (n!) = n lnn n a. write a function that meets the following specifications: def simpson (n): n is a positive integer. In mathematics, stirling's approximation (or stirling's formula) is an asymptotic approximation for factorials. it is a good approximation, leading to accurate results even for small values of n {\displaystyle n} .

Solved 5 10 Pts Stirling S Approximation Is Used To Chegg Stirling’s approximation is an important approximation for factorials. it is leading to accurate results even for small values of \ (n\). it is named after james stirling, though it was first stated by abraham de moivre. first, we know that \ (n!=1\cdot2\cdot\cdot\cdot n\). What is the value of ‘p’ in stirling’s formula? explanation: the stirling formula or stirling’s approximation formula is used to give the approximate value for a factorial function. it helps in finding out the factorial of larger numbers. although, the chief value comes from limiting the factorials. 5. what kind of relation is stirling’s formula?. The stirling formula is an approximation used to estimate the factorial of a large positive integer. it's given by \(n! \approx \sqrt{(2\pi n)} \times \left(\frac{n}{e}\right )^{n}\), where \(n\) is the integer and \(e\) is the base of the natural logarithm . Write a function that meets the following specifications: def simpson (n): n is a positive integer. the function should return both the estimated value of in (n!) and the percent error in the estimation based on the formula: error=1 [nln (n) n] in (n!) b.
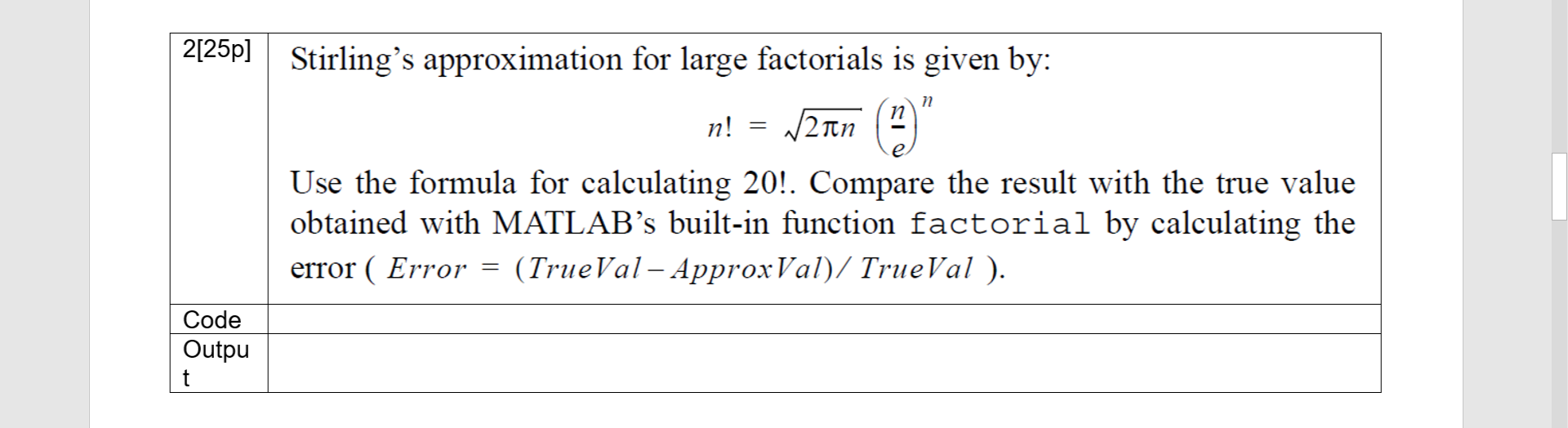
Solved N 2 25 Stirling S Approximation For Large Factorials Chegg The stirling formula is an approximation used to estimate the factorial of a large positive integer. it's given by \(n! \approx \sqrt{(2\pi n)} \times \left(\frac{n}{e}\right )^{n}\), where \(n\) is the integer and \(e\) is the base of the natural logarithm . Write a function that meets the following specifications: def simpson (n): n is a positive integer. the function should return both the estimated value of in (n!) and the percent error in the estimation based on the formula: error=1 [nln (n) n] in (n!) b. Use stirling's formula to evaluate lim n → ∞ Γ (n 3 2) n Γ (n 1). the limit is 1. stirling's formula is an approximation for factorials, given by n! ≈ 2 π n (n e) n for the gamma function, the approximation is similar: Γ (n 1) ≈ 2 π n (n e) n. using stirling's formula for Γ (n 1), we have: Γ (n 1) ≈ 2 π n (n e) n. Stirling’s formula provides an approximation to n! which is relatively easy to compute and is sufficient for most purposes. using it, one can evaluate log n! to better and better accuracy as n becomes large, provided that one can evaluate log n as accurately as needed. Given stirling's formula, we have \[\begin{align} n! &\approx \sqrt{2 \pi n} \left ( \frac {n}{e} \right )^n \\ (2n)! &\approx \sqrt{4 \pi n} \left ( \frac {2n}{e} \right )^{2n}. \end{align} \] taking the ratio of the second approximation to the square of the first approximation,. Find the limit $$\displaystyle \lim {n\rightarrow\infty}{\left(1 \frac{1}{n}\right)^{n^2}\frac{n!}{n^n\sqrt{n}}},$$ using stirling's approximation $$n!=\sqrt{2\pi n}\left(\frac{n}{e}\right)^n e^{\f.

Solved 1 59 We Can Derive Stirling S Approximation Form An Chegg Use stirling's formula to evaluate lim n → ∞ Γ (n 3 2) n Γ (n 1). the limit is 1. stirling's formula is an approximation for factorials, given by n! ≈ 2 π n (n e) n for the gamma function, the approximation is similar: Γ (n 1) ≈ 2 π n (n e) n. using stirling's formula for Γ (n 1), we have: Γ (n 1) ≈ 2 π n (n e) n. Stirling’s formula provides an approximation to n! which is relatively easy to compute and is sufficient for most purposes. using it, one can evaluate log n! to better and better accuracy as n becomes large, provided that one can evaluate log n as accurately as needed. Given stirling's formula, we have \[\begin{align} n! &\approx \sqrt{2 \pi n} \left ( \frac {n}{e} \right )^n \\ (2n)! &\approx \sqrt{4 \pi n} \left ( \frac {2n}{e} \right )^{2n}. \end{align} \] taking the ratio of the second approximation to the square of the first approximation,. Find the limit $$\displaystyle \lim {n\rightarrow\infty}{\left(1 \frac{1}{n}\right)^{n^2}\frac{n!}{n^n\sqrt{n}}},$$ using stirling's approximation $$n!=\sqrt{2\pi n}\left(\frac{n}{e}\right)^n e^{\f.

Solved 5 Use Stirling S Formula To Find An Approximation Chegg Given stirling's formula, we have \[\begin{align} n! &\approx \sqrt{2 \pi n} \left ( \frac {n}{e} \right )^n \\ (2n)! &\approx \sqrt{4 \pi n} \left ( \frac {2n}{e} \right )^{2n}. \end{align} \] taking the ratio of the second approximation to the square of the first approximation,. Find the limit $$\displaystyle \lim {n\rightarrow\infty}{\left(1 \frac{1}{n}\right)^{n^2}\frac{n!}{n^n\sqrt{n}}},$$ using stirling's approximation $$n!=\sqrt{2\pi n}\left(\frac{n}{e}\right)^n e^{\f.
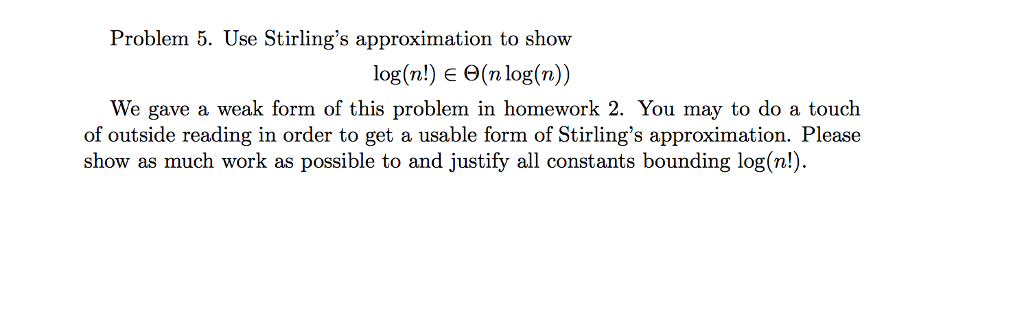
Solved Problem 5 Use Stirling S Approximation To Show Chegg
