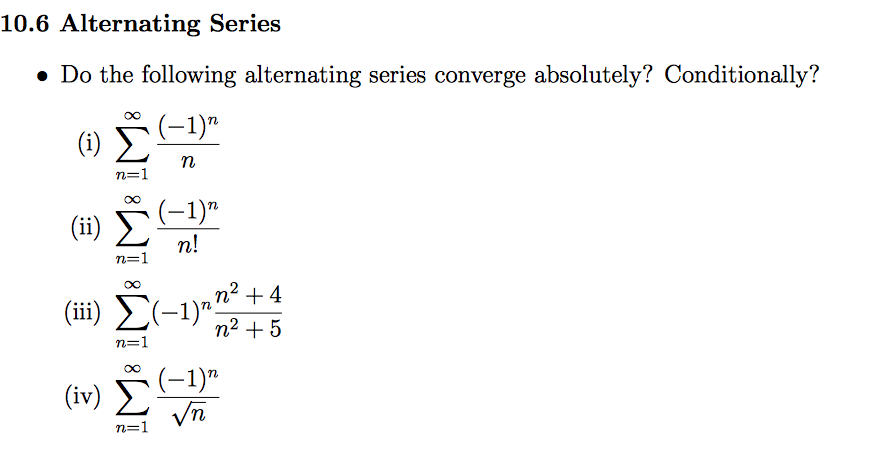
Solved Alternating Series Do The Following Alternating Chegg Analyze the following alternating series for absolute and conditional convergence. ∑n=2∞2n3 n−2(−1)n 1 your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. Our expert help has broken down your problem into an easy to learn solution you can count on. (5) use the alternating series test to determine whether the following series converge. there are 3 steps to solve this one. not the question you’re looking for? post any question and get expert help quickly.

Solved 5 Analyze The Following Alternating Series For Chegg 5) use the alternating series test to show that the following series converge. 1. p 1 n=1 ( 1)n 1 p answer: let a n = 1= p n. then replacing nby n 1 we have a n 1 = 1= n 1. since n 1 > p n, we have p1 n 1
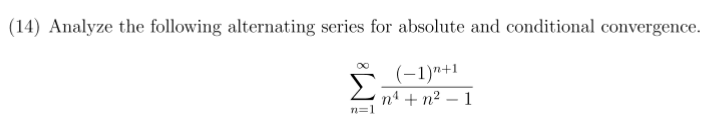
Solved 14 Analyze The Following Alternating Series For Chegg One of the two test conditions that need to be met to establish that an alternating series is convergent isan inequality. question: consider the following alternating series.∑n=1∞( 1)n25n 3= 14 213 19 223 cdotsyou will show that this alternating series is convergent by running the alternating series test.a. The alternating series test: state the conditions necessary to apply the alternating series test, and how to draw a conclusion. then use the alternating series test to determine if the following series is conditionally convergent. 1) which test is used to check the behaviour of alternating series? give an example. 2) find all the eigenvalues of a $3 \times 3$ matrix whose all entries are 3 . 3) find the taylor series generated by $f(x)=\theta^{x}$ at $x=2$. 4) determine whether the series $\sum {n=1}^{\infty} \frac{2^{n 2}}{n^{n}}$ converges or diverges. In this section we explore series whose summation includes negative terms. we start with a very specific form of series, where the terms of the summation alternate between being positive and negative. let {an} be a positive sequence. an alternating series is a series of either the form. ∞ ∑ n = 1(− 1)nan or ∞ ∑ n = 1(− 1)n 1an. Alternating series test if the numbers an satisfy the three conditions (i) an > 0 for all n (each an is positive) (ii) an > an 1 (the terms an are monotonically decreasing) (iii) ! lim n"# an = 0 then ∑the alternating series n=1 ∞ (–1) n 1 an converges. proof: in order to show that the alternating series converges, we need to show that. For each of the following series determine if the series converges or diverges. here is a set of practice problems to accompany the alternating series test section of the series & sequences chapter of the notes for paul dawkins calculus ii course at lamar university.
