
Solved Spherical Shell Charge Consider A Spherical Shell Chegg The charge is uniformly distributed,so the surface charge density is η=q4πr2.which of the following gives the radial component of the electric field at a point justoutside the sphere, at location r=r ? there may be more than one correct. your solution’s ready to go!. Find the energy of a uniformly charged spherical shell of total charge q and radius r. solution 1 use eq. 2.43, in the version appropriate to surface charges: w=21∫σvda. now, the potential at the surface of this sphere is (1 4πϵ0)q r (a constantex. 2.7), so w=8πϵ01rq∫σda=8πϵ01rq2. solution 2 use eq. 2.45. inside the sphere, e=0; outside,.
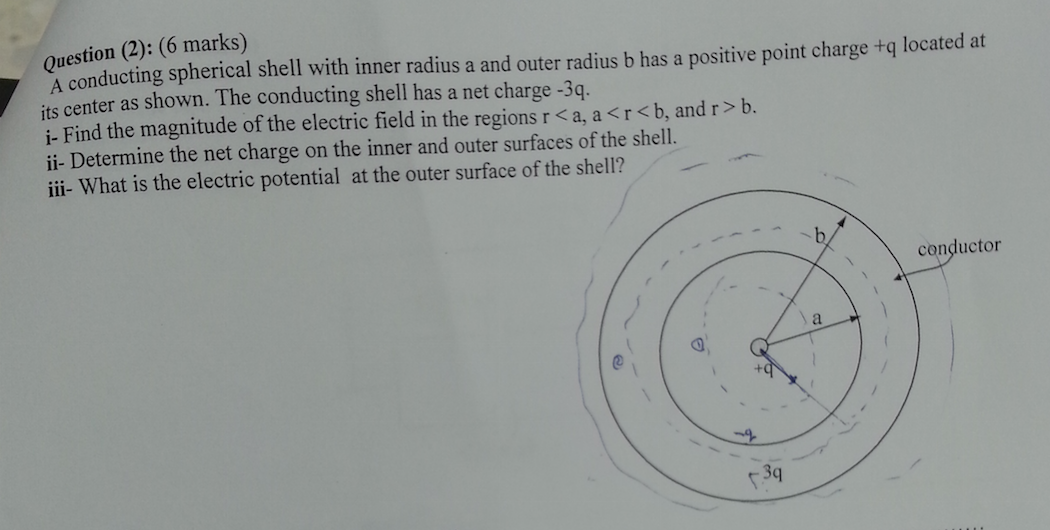
Solved A Conducting Spherical Shell With Inner Radius A And Chegg Griffiths 2.8, 2.32 a solid sphere of radius r has a uniform charge density ρ and total charge q. derive an expression for its total electric potential energy. (suggestion: imagine that the sphere is constructed by adding successive layers of concentric shells of charge dq =(4πr2 dr)ρ and use du =v dq.). A spherical shell has total charge q and radius r. the charge is uniformly distributed, so the surface charge density is η=q4πr2.which of the following gives the radial component of the electric field at a point just outside the sphere, at location r=r ? there may be more than one correct response. your solution’s ready to go!. A thin spherical shell of radius r has total charge q. what is the electric potential at the center of the shell? find the equation of the tangent to the curve y=frac e2xx at the point 1,e2. The total charge inside is q, the charge on the shell. gauss’s law, which relates the electric ux through the gaussian surface to the net charge inside, thus produces an equation that can be solved for the eld e(r), which is the familiar coulomb eld. if r < r, then the spherical shell is outside the gaussian surface, which implies that there.

Solved A Spherical Shell Has Total Charge Q ï And Radius R Chegg A thin spherical shell of radius r has total charge q. what is the electric potential at the center of the shell? find the equation of the tangent to the curve y=frac e2xx at the point 1,e2. The total charge inside is q, the charge on the shell. gauss’s law, which relates the electric ux through the gaussian surface to the net charge inside, thus produces an equation that can be solved for the eld e(r), which is the familiar coulomb eld. if r < r, then the spherical shell is outside the gaussian surface, which implies that there. Inner radius and outer radius of the spherical shell : r i n and r o u t. the shell contains total charge : q. the interior of the shell is empty of charge and matter. theory used : the quantity of electric field that passes through a closed surface is referred to as the electric flux. The correct answer for the electric field outside of a spherical shell is $$e {r \geq r} = \frac{\sigma r^2}{\epsilon 0 r^2} \hat{r}.$$ inside the spherical shell ($r \leq r$), there is no charge! therefore, the electric field is $0$. In a uniform charge distribution, the charge density \( \rho \) can be defined for a volume as \[ \rho = \frac{q}{v}, \] where \( q \) is the total charge and \( v \) is the volume of the distribution. A spherical shell of radius r has a uniformly distributed total charge q on its surface. the shell is rotated around an axis that passes through its geometric center at an angular frequency w. 1. if the angular velocity is constant w=wo, i. calculate the electromagnetic energy of the system. ii.
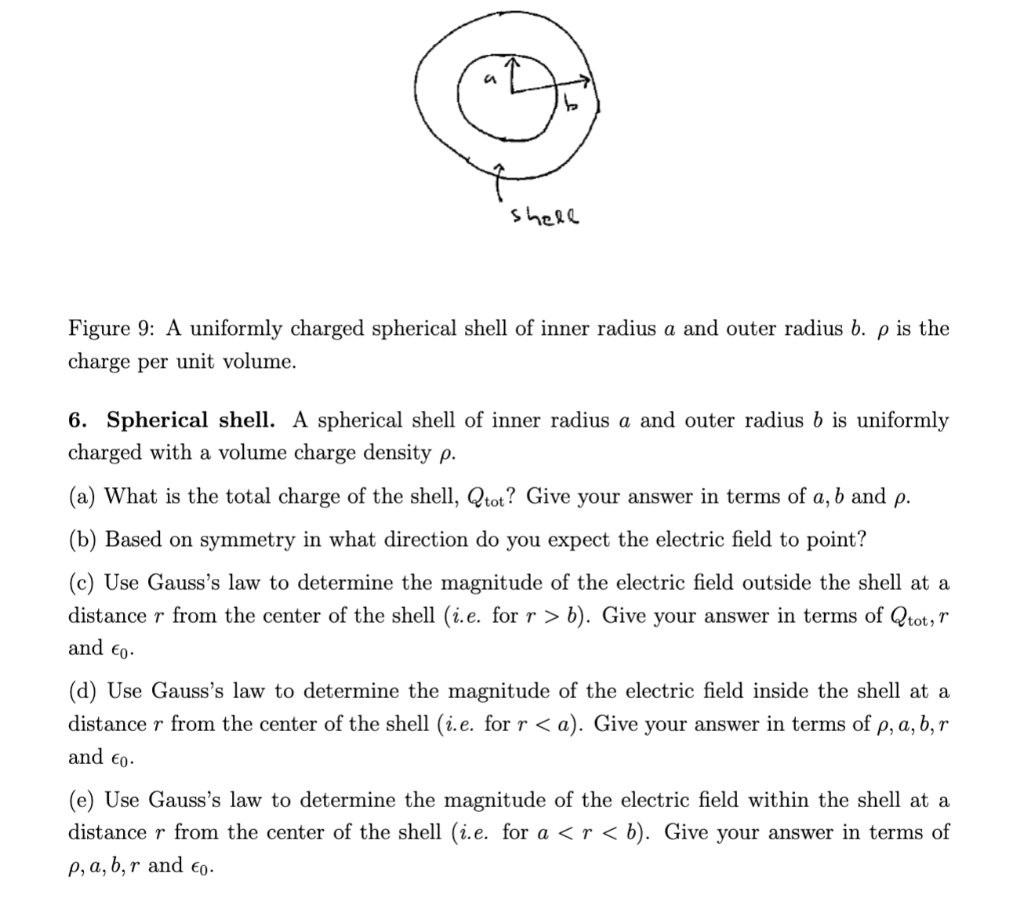
Solved Figure 9 A Uniformly Charged Spherical Shell Of Chegg Inner radius and outer radius of the spherical shell : r i n and r o u t. the shell contains total charge : q. the interior of the shell is empty of charge and matter. theory used : the quantity of electric field that passes through a closed surface is referred to as the electric flux. The correct answer for the electric field outside of a spherical shell is $$e {r \geq r} = \frac{\sigma r^2}{\epsilon 0 r^2} \hat{r}.$$ inside the spherical shell ($r \leq r$), there is no charge! therefore, the electric field is $0$. In a uniform charge distribution, the charge density \( \rho \) can be defined for a volume as \[ \rho = \frac{q}{v}, \] where \( q \) is the total charge and \( v \) is the volume of the distribution. A spherical shell of radius r has a uniformly distributed total charge q on its surface. the shell is rotated around an axis that passes through its geometric center at an angular frequency w. 1. if the angular velocity is constant w=wo, i. calculate the electromagnetic energy of the system. ii.

Solved Calculate The Total Charge In A Spherical Shell With Chegg In a uniform charge distribution, the charge density \( \rho \) can be defined for a volume as \[ \rho = \frac{q}{v}, \] where \( q \) is the total charge and \( v \) is the volume of the distribution. A spherical shell of radius r has a uniformly distributed total charge q on its surface. the shell is rotated around an axis that passes through its geometric center at an angular frequency w. 1. if the angular velocity is constant w=wo, i. calculate the electromagnetic energy of the system. ii.
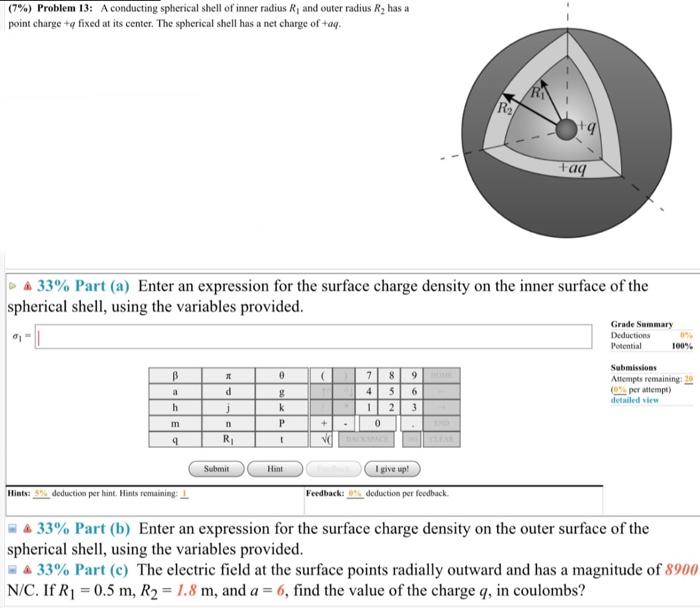
Solved 7 Problem 13 A Conducting Spherical Shell Of Chegg
