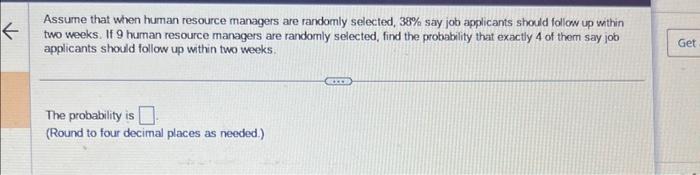
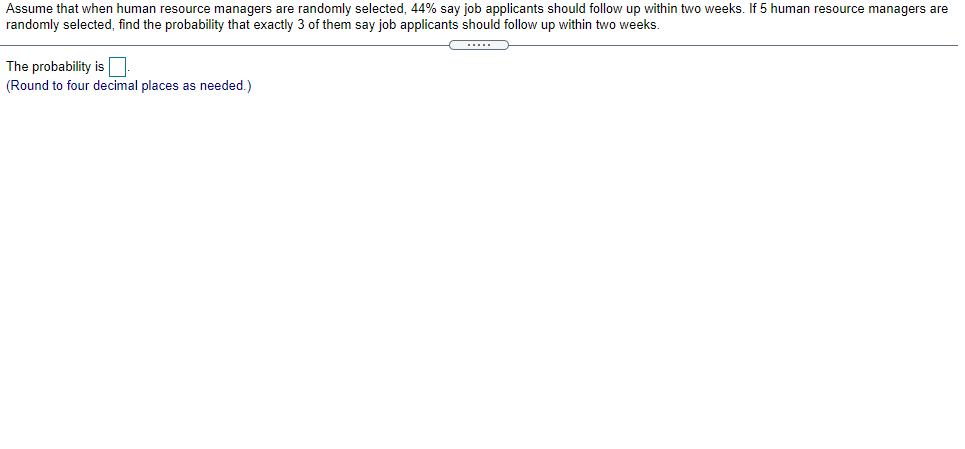
Solved Assume That When Human Resource Managers Are Randomly Chegg Enhanced with ai, our expert help has broken down your problem into an easy to learn solution you can count on. If 5 human resource managers are randomly selected, find the probability that exactly 3 of them say job applicants should follow up within two weeks. sure, this is a binomial probability problem. the binomial distribution model is appropriate for a statistical experiment if the following conditions are met:.
Solved Assume That When Human Resource Managers Are Randomly Chegg In this case, the experiment is selecting 7 human resource managers, the success is a manager saying job applicants should follow up within two weeks, and the probability of success is 41%. the formula for binomial probability is: p(x=k) = c(n, k) * (p^k) * ((1 p)^(n k)) where: p(x=k) is the probability of k successes in n trials. The probability that exactly 2 of the 6 job applicants should follow up with in two weeks is 0.2153. x be the number of job applicants should follow up with in two weeks. x follows the binomial distribution with n = 6, p =52% = 0.52 and q = 1 p = 48% = 0.48. If 8 human resource managers are randomly selected, find the probability that at least 2 of them say job applicants should follow up within two weeks. the probability is (round to four decimal places as needed.) a pharmaceutical company. your solution’s ready to go!. Based on a society for human resource management survey, 36% of human resource professionals are at companies that rejected job candidates because of information found on their social media.

Solved Assume That When Human Resource Managers Are Randomly Chegg If 8 human resource managers are randomly selected, find the probability that at least 2 of them say job applicants should follow up within two weeks. the probability is (round to four decimal places as needed.) a pharmaceutical company. your solution’s ready to go!. Based on a society for human resource management survey, 36% of human resource professionals are at companies that rejected job candidates because of information found on their social media. The problem satisfies the binomial distribution because there are only two possible outcomes in each trial, either the resource manager will say job applicants should follow up within two weeks or not. the probability of success for this is also constant. Question: assume that when human resource managers are randomly selected 43% a job applicant should follow up within two weeks if eight human resource managers are randomly selected. find the probability that exactly 2 of them say job applicants should follow up with two weeks. Managers are randomly selected, find the probability that exactly 3 of them say job applicants should follow up within two weeks. the probability is , (round to four decimal places as needed') see the explanation section for the calculations. answer: the probability is 0.3397. the problem is a binomial probability. Assume that when human resources managers are randomly selected, 55% say job applicants should follow up within two weeks. if 5 human resource managers are randomly selected, find the probability that exactly 2 of them say job applicants should follow up within two weeks. solution this is a binomial probability problem.
