
Solved Spherical Conducting Shell A Spherical Conducting Chegg Consider a spherical conducting shell with inner radius 5 cm, outer radius 10 cm with a charge of 50 nc, concentric with a solid insulating sphere with radius 2 cm and charge of −10nc. calculate the electric field 8 cm away from the center in n c. Physics ninja looks at a classic gauss's law problem involving a sphere and a conducting shell. the inner sphere can be a conductor or an insulator and the outer shell is assumed to be a.

Solved Consider A Conducting Spherical Shell With Inner And Chegg Consider a spherical conducting shell. such a surface will have equal charges on its inner and outer surfaces but, because their areas differ, so will their charge densities. A point charge with magnitude q is located inside the cavity of a spherical conducting shell. the shell has an inner radius equal to a, an outer radius equal to b, and holds a net charge of 3q, as shown in the figure. A point charge q rests at the center of an uncharged thin spherical conducting shell. (see fig. 16–34.) what is the electric field e as a function of r r for r r r less than the inner radius of the shell, inside the shell, and beyond the shell? how does the shell affect the field due to q alone? how does the charge q affect the shell?. Question: consider a spherical conducting shell with inner radius r1=5.0cm and outer radius r2=6.0cm, with a net charge of q=3.6uc and with a particle of charge q=5.2uc at the center (see figure.) (i) determine the charge at the inner surface of the shell (call it q1). i got 5.2uc, correct (ii) determine the charge.
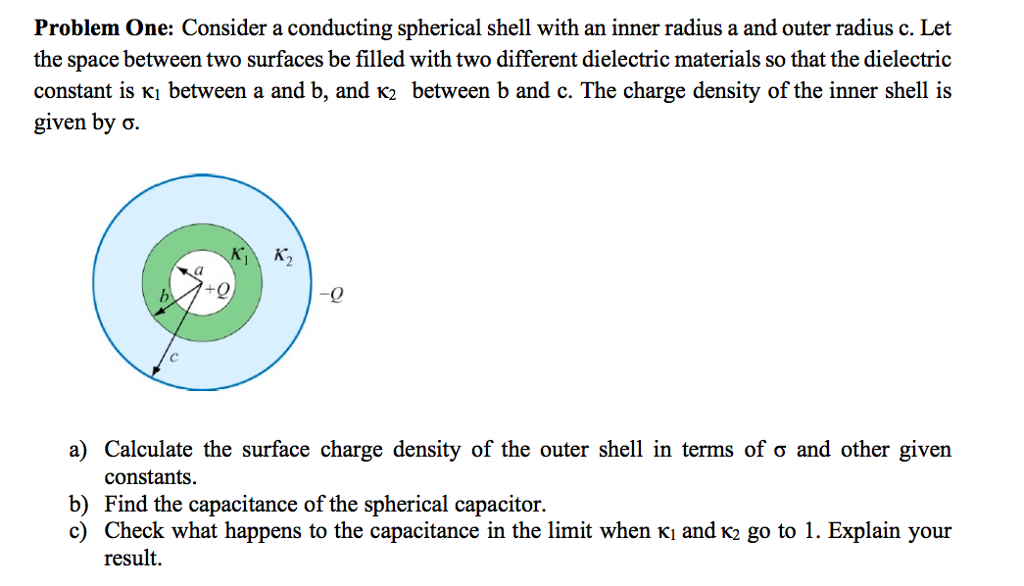
Solved Problem One Consider A Conducting Spherical Shell Chegg A point charge q rests at the center of an uncharged thin spherical conducting shell. (see fig. 16–34.) what is the electric field e as a function of r r for r r r less than the inner radius of the shell, inside the shell, and beyond the shell? how does the shell affect the field due to q alone? how does the charge q affect the shell?. Question: consider a spherical conducting shell with inner radius r1=5.0cm and outer radius r2=6.0cm, with a net charge of q=3.6uc and with a particle of charge q=5.2uc at the center (see figure.) (i) determine the charge at the inner surface of the shell (call it q1). i got 5.2uc, correct (ii) determine the charge. 4πk (b − a) ε0 if r > b e(r) is constant on the spherical gaussian surface, so it can be pulled in front of the integral. Step 2: electric field between the solid sphere and inner surface of the shell (b < r < c) for a spherical shell, the electric field inside is zero. hence, the electric field in this region is also 0. A resistor consists of two concentric conducting spherical shells with the inner shell having radius and the outer shell having radius . the space between the two shells is filled with a material of resistivity . In the present post, i will consider the problem of calculating the electric field due to a point charge q q surrounded by a conductor which has the form of a thick spherical shell. the conductor has zero net electric charge. the inner radius of the shell is r1 r 1, and the outer radius is r2 r 2.
