
Solved Consider The Following A Give The Number Of Edges Chegg (a) give the number of edges in the graph. edges (b) give the number of vertices in the graph. vertices (c) determine the number of vertices that are of odd degree. vertices (d) determine whether the graph is connected yes no (e) determine whether the graph is a complete graph. Answer: ala on sim belo. explanation: daplanation ( give tiu no. of edges. 8 edges. e= (a,b),(a,c),(a,e),(b,c), (c,d),(d,e),(e,f) 6) no. at vestices. →6 vealices. v= a,b,c,d,e,f ( no. c vertices that are add are 6 vealices.
Solved Consider The Following A Give The Number Of Edges Chegg Explanation: 1 (a) nuabex of edgers =6(8dot cx) (3 ) numbex of vertices =4 (c) a has degree 3, b has degree 3, b and i also have degree 3. so, the number of vestices that are of odd degree =4. 1 count the number of line segments connecting the vertices in the graph to find the number of edges. Find the relation between the number of edges, and the in degree and out degree. the number of edges is 13. the number of edges, the sum of the in degrees, and the sum of the out degrees are all equal. consider an acquaintanceship graph, where vertices represent all the people in the world. Count the blue lines that connect two black dots, which will represent the number of edges in the graph.
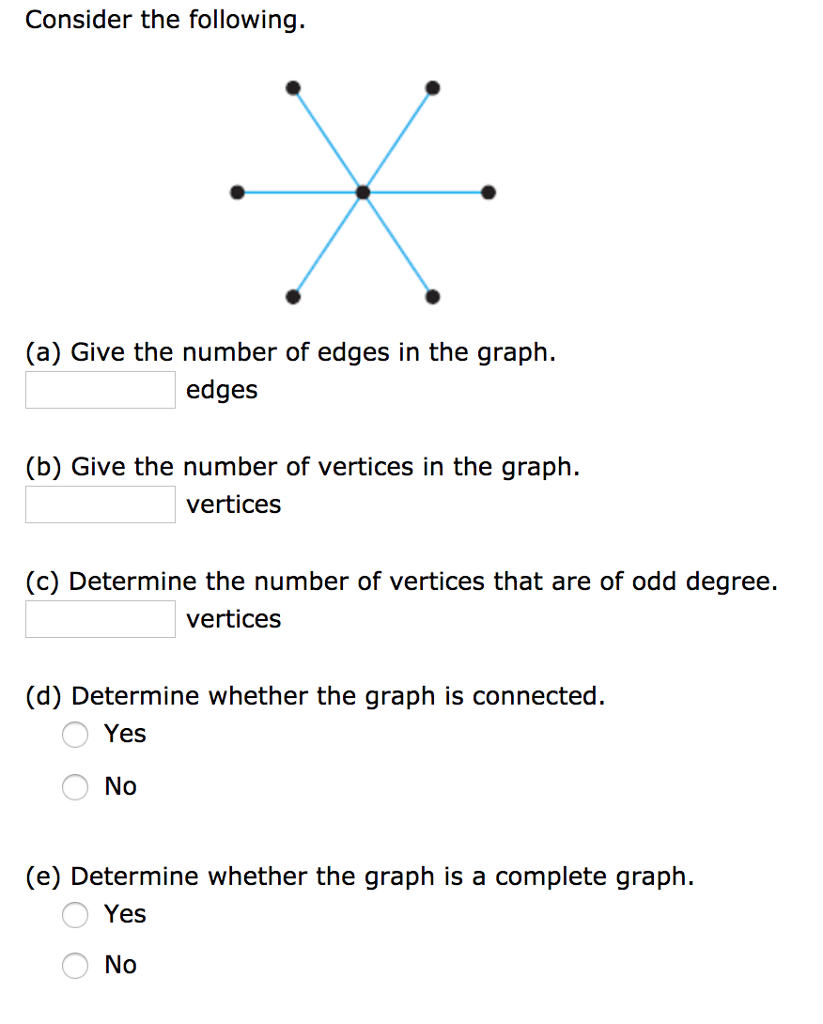
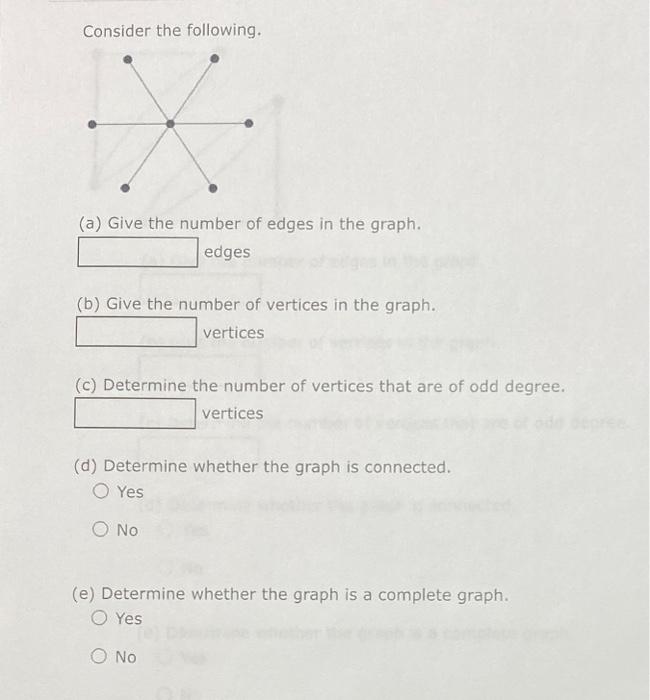
Solved Consider The Following A Give The Number Of Edges Chegg Find the relation between the number of edges, and the in degree and out degree. the number of edges is 13. the number of edges, the sum of the in degrees, and the sum of the out degrees are all equal. consider an acquaintanceship graph, where vertices represent all the people in the world. Count the blue lines that connect two black dots, which will represent the number of edges in the graph. Given a graph g = (v; e) with positive edge weights, the bellman ford algo rithm and dijkstra’s algorithm can produce different shortest path trees despite always producing the same shortest path weights. solution: true. (a) give the number of edges in the graph. $\square$ 6 edges (b) give the number of vertices in the graph. $\square$ 6 vertices (c) determine the number of vertices that are of odd degree. 3 $\square$ vertices (d) determine whether the graph is connected. Answer to consider the following. (a) give the number of edges in the graph . Consider the following. (a) give the number of edges in the graph. (b) give the number of vertices in the graph. (c) determine the number of vertices that are of odd degree.
