
Solved 3 Consider The Sequence Given By An 6тле4тизnтит1 A Find Chegg There are 4 steps to solve this one. we have the sequence (a n) defined by a 1 = 3 and a n 1 = 1 4 − a n . consider the sequence {an} given by a1 =3,an 1 = 4−an1. (a) prove that this sequence converges. (b) explain why the sequence {an 1} also converges to the same value and use this idea to find the limit of the sequence. Consider the sequence (a n ) where a 1 = 3 and a n 1 = 4 − a n 1 (a) (3 points) show that (a n ) is bounded and monotone, using this to show the sequence has a limit. (b) (5 points) argue that lim n → ∞ a n 1 = lim n → ∞ a n and use this and some algebra to calculate what the limit must be.
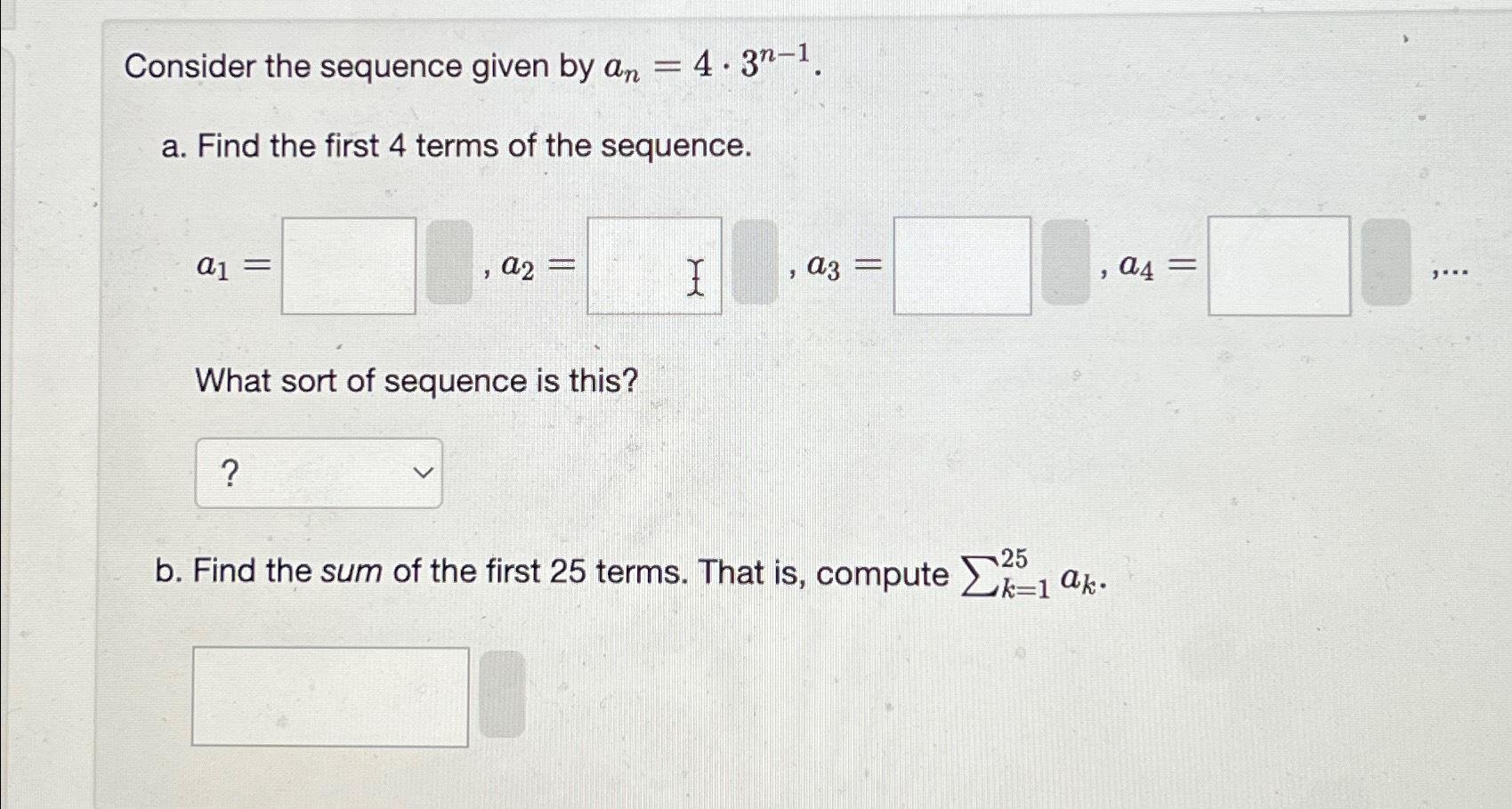
Solved Consider The Sequence Given By An 4 3n 1 A ï Find The Chegg Free sequence calculator step by step solutions to help identify the sequence and find the nth term of arithmetic and geometric sequence types. Check that an = 2n 1 a n = 2 n 1 is a solution to the recurrence relation an = 2an−1−1 a n = 2 a n − 1 − 1 with a1 = 3. a 1 = 3. first, it is easy to check the initial condition: \ (a 1\) should be \ (2^1 1\) according to our closed formula. indeed, \ (2^1 1 = 3\text {,}\) which is what we want. Free online sequences calculator find sequence types, indices, sums and progressions step by step. Solve the equation, and you get two roots 3 5√ 2 3 5 2 and 3− 5√ 2 3 − 5 2. you know the sequence is increasing and a1 = 1 a 1 = 1 so 3 5√ 2 3 5 2 is the correct limit. hint: in the limit as n → ∞ n → ∞, an 1 ∼an a n 1 ∼ a n. so set an 1 =an = a a n 1 = a n = a. then.
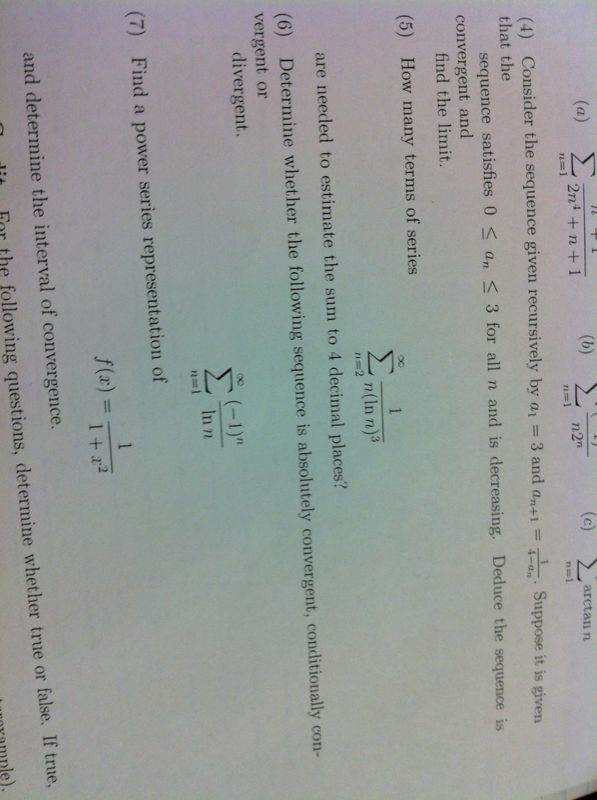
Solved Consider The Sequence Given Recursively By A1 3 And Chegg Free online sequences calculator find sequence types, indices, sums and progressions step by step. Solve the equation, and you get two roots 3 5√ 2 3 5 2 and 3− 5√ 2 3 − 5 2. you know the sequence is increasing and a1 = 1 a 1 = 1 so 3 5√ 2 3 5 2 is the correct limit. hint: in the limit as n → ∞ n → ∞, an 1 ∼an a n 1 ∼ a n. so set an 1 =an = a a n 1 = a n = a. then. Consider the sequence given recursively by a1 = 3 and an 1 = 1 4 an. suppose it is given that the sequence satisfies 0 an 3 for all n and is decreasing. deduce the sequence is convergent and find the limit. Given an , let an 1 = (1 an)^1 2 for n ≥ 1 . then consider the sequence of real numbers: a1 , a2 , a3 , , an , . the goal is to prove that this sequence converges, and then to find its limit l . (a) use a calculator or computer to find. your solution’s ready to go!. Ex9.1 , 11 write the first five terms of the following sequence and obtain the corresponding series: a1 = 3,an = 3an – 1 2 for all n >1 it is given that a1 = 3 for a2 onwards, we have to use formula an = 3an – 1 2 putting n = 2 in (1) a2 = 3a2 1 2 = 3a1 2 = 3 ×. Consider the sequence {an} which is given as a1=0,a2=1, andan=an 1 an 22 for n≥3. that is, every term is the average of the previous two. so, the first few terms of the sequence are: 0,1,12,34,58find the value oflimn→∞an.
